Menu Close

calculate-lim-x-0-sh-2sinx-sin-sh-2x-x-2-

Tinku Tara 0 Comments
Pin




Question Number 148568 by mathmax by abdo last updated on 29/Jul/21
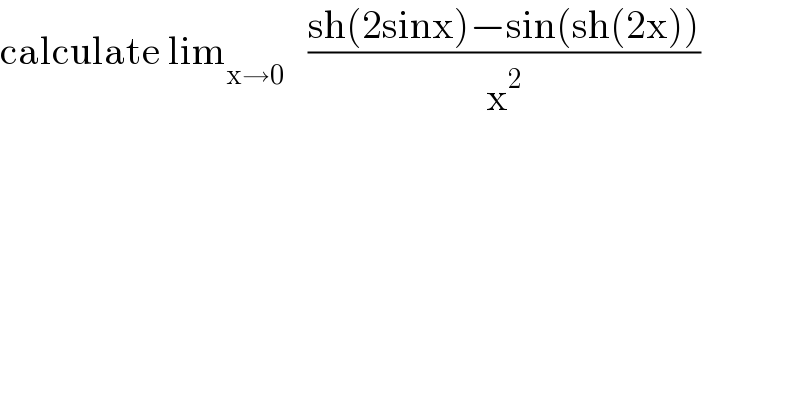
calculate lim_(x→0) ((sh(2sinx)−sin(sh(2x)))/x^2 )
$$\mathrm{calculate}\:\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \:\:\:\frac{\mathrm{sh}\left(\mathrm{2sinx}\right)−\mathrm{sin}\left(\mathrm{sh}\left(\mathrm{2x}\right)\right)}{\mathrm{x}^{\mathrm{2}} } \\ $$
Answered by Ar Brandon last updated on 29/Jul/21
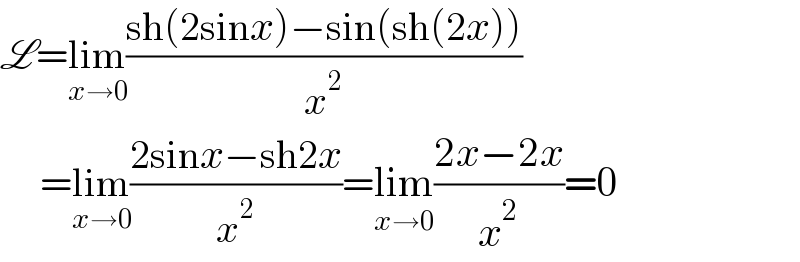
L=lim_(x→0) ((sh(2sinx)−sin(sh(2x)))/x^2 ) =lim_(x→0) ((2sinx−sh2x)/x^2 )=lim_(x→0) ((2x−2x)/x^2 )=0
$$\mathscr{L}=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{sh}\left(\mathrm{2sin}{x}\right)−\mathrm{sin}\left(\mathrm{sh}\left(\mathrm{2}{x}\right)\right)}{{x}^{\mathrm{2}} } \\ $$$$\:\:\:\:\:=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{2sin}{x}−\mathrm{sh2}{x}}{{x}^{\mathrm{2}} }=\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{2}{x}−\mathrm{2}{x}}{{x}^{\mathrm{2}} }=\mathrm{0} \\ $$
Answered by mathmax by abdo last updated on 29/Jul/21
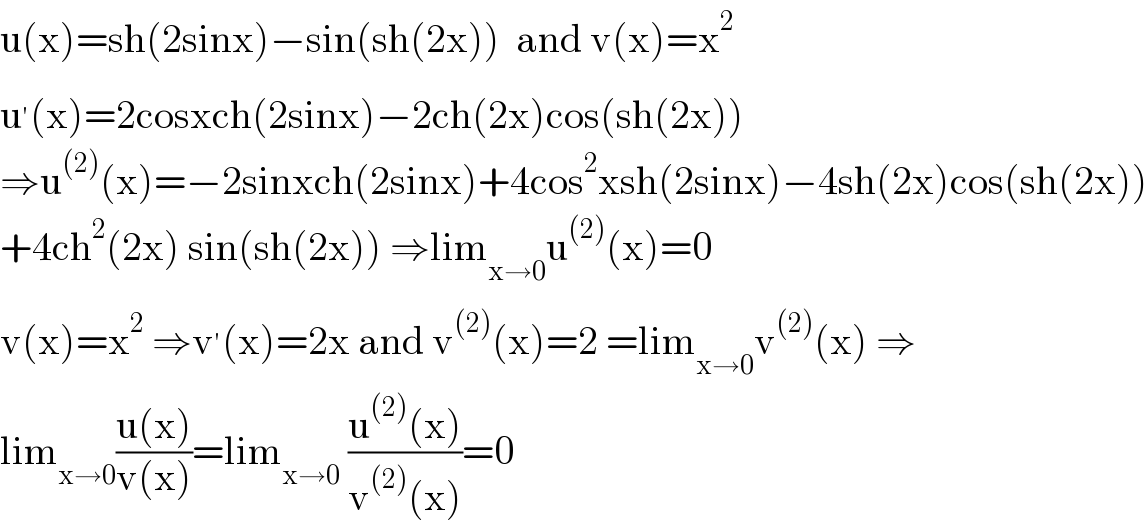
u(x)=sh(2sinx)−sin(sh(2x)) and v(x)=x^2 u^′ (x)=2cosxch(2sinx)−2ch(2x)cos(sh(2x)) ⇒u^((2)) (x)=−2sinxch(2sinx)+4cos^2 xsh(2sinx)−4sh(2x)cos(sh(2x)) +4ch^2 (2x) sin(sh(2x)) ⇒lim_(x→0) u^((2)) (x)=0 v(x)=x^2 ⇒v^′ (x)=2x and v^((2)) (x)=2 =lim_(x→0) v^((2)) (x) ⇒ lim_(x→0) ((u(x))/(v(x)))=lim_(x→0) ((u^((2)) (x))/(v^((2)) (x)))=0
$$\mathrm{u}\left(\mathrm{x}\right)=\mathrm{sh}\left(\mathrm{2sinx}\right)−\mathrm{sin}\left(\mathrm{sh}\left(\mathrm{2x}\right)\right)\:\:\mathrm{and}\:\mathrm{v}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{2}} \\ $$$$\mathrm{u}^{'} \left(\mathrm{x}\right)=\mathrm{2cosxch}\left(\mathrm{2sinx}\right)−\mathrm{2ch}\left(\mathrm{2x}\right)\mathrm{cos}\left(\mathrm{sh}\left(\mathrm{2x}\right)\right) \\ $$$$\Rightarrow\mathrm{u}^{\left(\mathrm{2}\right)} \left(\mathrm{x}\right)=−\mathrm{2sinxch}\left(\mathrm{2sinx}\right)+\mathrm{4cos}^{\mathrm{2}} \mathrm{xsh}\left(\mathrm{2sinx}\right)−\mathrm{4sh}\left(\mathrm{2x}\right)\mathrm{cos}\left(\mathrm{sh}\left(\mathrm{2x}\right)\right) \\ $$$$+\mathrm{4ch}^{\mathrm{2}} \left(\mathrm{2x}\right)\:\mathrm{sin}\left(\mathrm{sh}\left(\mathrm{2x}\right)\right)\:\Rightarrow\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \mathrm{u}^{\left(\mathrm{2}\right)} \left(\mathrm{x}\right)=\mathrm{0} \\ $$$$\mathrm{v}\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{2}} \:\Rightarrow\mathrm{v}^{'} \left(\mathrm{x}\right)=\mathrm{2x}\:\mathrm{and}\:\mathrm{v}^{\left(\mathrm{2}\right)} \left(\mathrm{x}\right)=\mathrm{2}\:=\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \mathrm{v}^{\left(\mathrm{2}\right)} \left(\mathrm{x}\right)\:\Rightarrow \\ $$$$\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \frac{\mathrm{u}\left(\mathrm{x}\right)}{\mathrm{v}\left(\mathrm{x}\right)}=\mathrm{lim}_{\mathrm{x}\rightarrow\mathrm{0}} \:\frac{\mathrm{u}^{\left(\mathrm{2}\right)} \left(\mathrm{x}\right)}{\mathrm{v}^{\left(\mathrm{2}\right)} \left(\mathrm{x}\right)}=\mathrm{0} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *