Question Number 1483 by Rasheed Ahmad last updated on 13/Aug/15

$$\mathrm{Prove}\:\mathrm{that}: \\ $$$$\mathrm{a}+\left(\mathrm{b}+\mathrm{c}\right)+\mathrm{d}=\left(\mathrm{a}+\mathrm{c}\right)+\left(\mathrm{d}+\mathrm{b}\right) \\ $$
Commented by prakash jain last updated on 13/Aug/15
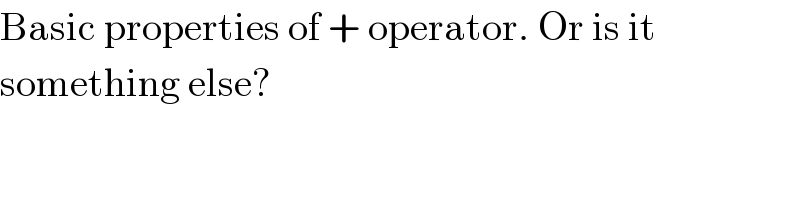
$$\mathrm{Basic}\:\mathrm{properties}\:\mathrm{of}\:+\:\mathrm{operator}.\:\mathrm{Or}\:\mathrm{is}\:\mathrm{it} \\ $$$$\mathrm{something}\:\mathrm{else}? \\ $$
Commented by Rasheed Ahmad last updated on 14/Aug/15
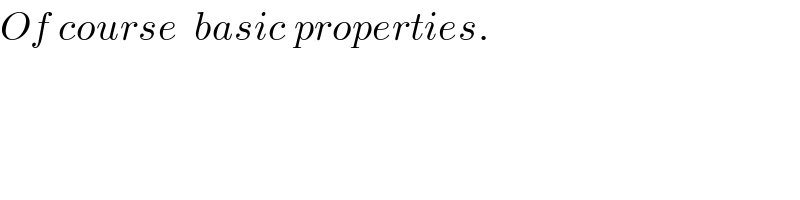
$${Of}\:{course}\:\:{basic}\:{properties}. \\ $$
Answered by Rasheed Soomro last updated on 16/Aug/15
![LHS: a+(b+c)+d =a+(c+b)+d [ Commutative w. r. t +] ={a+(c+b)}+d ={(a+c)+b}+d [Associative wrt + ] =(a+c)+{b+d} [ ∽ ] =(a+c)+(d+b) [ Commutative wrt +] =RHS](https://www.tinkutara.com/question/Q1525.png)
$$\boldsymbol{\mathrm{LHS}}:\:\mathrm{a}+\left(\mathrm{b}+\mathrm{c}\right)+\mathrm{d} \\ $$$$\:\:\:\:\:\:\:=\mathrm{a}+\left(\mathrm{c}+\mathrm{b}\right)+\mathrm{d}\:\:\:\:\:\left[\:\mathrm{Commutative}\:\mathrm{w}.\:\mathrm{r}.\:\mathrm{t}\:+\right] \\ $$$$\:\:\:\:\:\:\:=\left\{\mathrm{a}+\left(\mathrm{c}+\mathrm{b}\right)\right\}+\mathrm{d} \\ $$$$\:\:\:\:\:\:\:=\left\{\left(\mathrm{a}+\mathrm{c}\right)+\mathrm{b}\right\}+\mathrm{d}\:\:\:\left[\mathrm{Associative}\:\mathrm{wrt}\:+\:\:\right] \\ $$$$\:\:\:\:\:\:\:=\left(\mathrm{a}+\mathrm{c}\right)+\left\{\mathrm{b}+\mathrm{d}\right\}\:\:\:\left[\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\backsim\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\right] \\ $$$$\:\:\:\:\:\:\:=\left(\mathrm{a}+\mathrm{c}\right)+\left(\mathrm{d}+\mathrm{b}\right)\:\:\:\:\left[\:\mathrm{Commutative}\:\mathrm{wrt}\:+\right] \\ $$$$\:\:\:\:\:\:\:=\boldsymbol{\mathrm{RHS}} \\ $$
Commented by 123456 last updated on 16/Aug/15

$$\left.\mathrm{good}\::\right) \\ $$
