0-1-ln-1-x-1-x-dx-m-n-

Question Number 149077 by mnjuly1970 last updated on 02/Aug/21

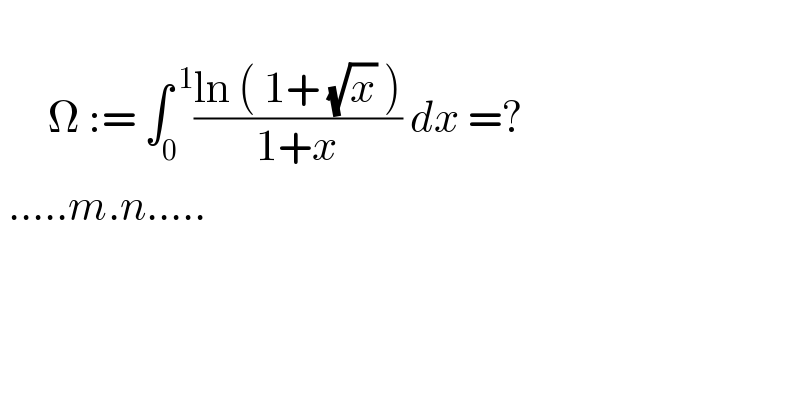
$$ \\ $$$$\:\:\:\:\:\:\Omega\::=\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\mathrm{ln}\:\left(\:\mathrm{1}+\:\sqrt{{x}}\:\right)}{\mathrm{1}+{x}}\:{dx}\:=? \\ $$$$\:…..{m}.{n}….. \\ $$

Answered by mindispower last updated on 02/Aug/21

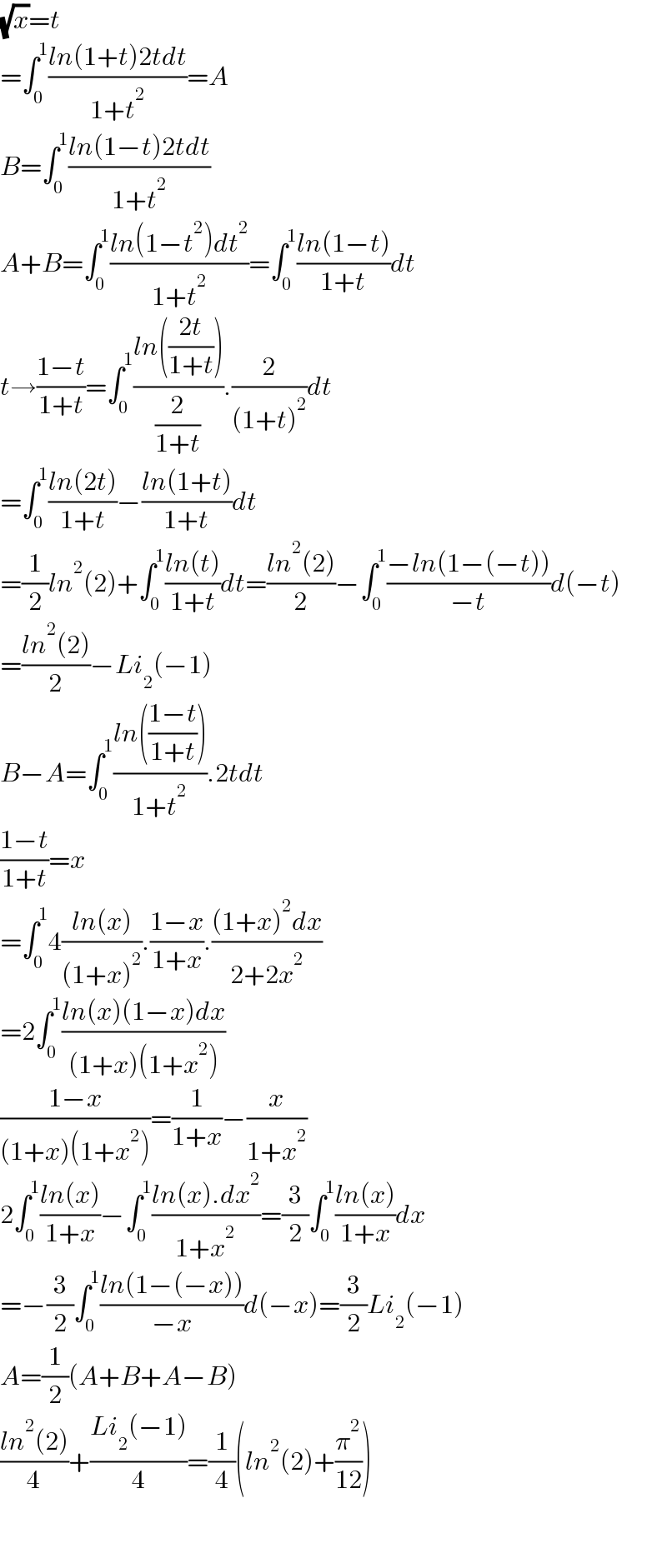
(√x)=t =∫_0 ^1 ((ln(1+t)2tdt)/(1+t^2 ))=A B=∫_0 ^1 ((ln(1−t)2tdt)/(1+t^2 )) A+B=∫_0 ^1 ((ln(1−t^2 )dt^2 )/(1+t^2 ))=∫_0 ^1 ((ln(1−t))/(1+t))dt t→((1−t)/(1+t))=∫_0 ^1 ((ln(((2t)/(1+t))))/(2/(1+t))).(2/((1+t)^2 ))dt =∫_0 ^1 ((ln(2t))/(1+t))−((ln(1+t))/(1+t))dt =(1/2)ln^2 (2)+∫_0 ^1 ((ln(t))/(1+t))dt=((ln^2 (2))/2)−∫_0 ^1 ((−ln(1−(−t)))/(−t))d(−t) =((ln^2 (2))/2)−Li_2 (−1) B−A=∫_0 ^1 ((ln(((1−t)/(1+t))))/(1+t^2 )).2tdt ((1−t)/(1+t))=x =∫_0 ^1 4((ln(x))/((1+x)^2 )).((1−x)/(1+x)).(((1+x)^2 dx)/(2+2x^2 )) =2∫_0 ^1 ((ln(x)(1−x)dx)/((1+x)(1+x^2 ))) ((1−x)/((1+x)(1+x^2 )))=(1/(1+x))−(x/(1+x^2 )) 2∫_0 ^1 ((ln(x))/(1+x))−∫_0 ^1 ((ln(x).dx^2 )/(1+x^2 ))=(3/2)∫_0 ^1 ((ln(x))/(1+x))dx =−(3/2)∫_0 ^1 ((ln(1−(−x)))/(−x))d(−x)=(3/2)Li_2 (−1) A=(1/2)(A+B+A−B) ((ln^2 (2))/4)+((Li_2 (−1))/4)=(1/4)(ln^2 (2)+(π^2 /(12)))

$$\sqrt{{x}}={t} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left(\mathrm{1}+{t}\right)\mathrm{2}{tdt}}{\mathrm{1}+{t}^{\mathrm{2}} }={A} \\ $$$${B}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left(\mathrm{1}−{t}\right)\mathrm{2}{tdt}}{\mathrm{1}+{t}^{\mathrm{2}} } \\ $$$${A}+{B}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left(\mathrm{1}−{t}^{\mathrm{2}} \right){dt}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left(\mathrm{1}−{t}\right)}{\mathrm{1}+{t}}{dt} \\ $$$${t}\rightarrow\frac{\mathrm{1}−{t}}{\mathrm{1}+{t}}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left(\frac{\mathrm{2}{t}}{\mathrm{1}+{t}}\right)}{\frac{\mathrm{2}}{\mathrm{1}+{t}}}.\frac{\mathrm{2}}{\left(\mathrm{1}+{t}\right)^{\mathrm{2}} }{dt} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left(\mathrm{2}{t}\right)}{\mathrm{1}+{t}}−\frac{{ln}\left(\mathrm{1}+{t}\right)}{\mathrm{1}+{t}}{dt} \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{ln}^{\mathrm{2}} \left(\mathrm{2}\right)+\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left({t}\right)}{\mathrm{1}+{t}}{dt}=\frac{{ln}^{\mathrm{2}} \left(\mathrm{2}\right)}{\mathrm{2}}−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{−{ln}\left(\mathrm{1}−\left(−{t}\right)\right)}{−{t}}{d}\left(−{t}\right) \\ $$$$=\frac{{ln}^{\mathrm{2}} \left(\mathrm{2}\right)}{\mathrm{2}}−{Li}_{\mathrm{2}} \left(−\mathrm{1}\right) \\ $$$${B}−{A}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left(\frac{\mathrm{1}−{t}}{\mathrm{1}+{t}}\right)}{\mathrm{1}+{t}^{\mathrm{2}} }.\mathrm{2}{tdt} \\ $$$$\frac{\mathrm{1}−{t}}{\mathrm{1}+{t}}={x} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{4}\frac{{ln}\left({x}\right)}{\left(\mathrm{1}+{x}\right)^{\mathrm{2}} }.\frac{\mathrm{1}−{x}}{\mathrm{1}+{x}}.\frac{\left(\mathrm{1}+{x}\right)^{\mathrm{2}} {dx}}{\mathrm{2}+\mathrm{2}{x}^{\mathrm{2}} } \\ $$$$=\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left({x}\right)\left(\mathrm{1}−{x}\right){dx}}{\left(\mathrm{1}+{x}\right)\left(\mathrm{1}+{x}^{\mathrm{2}} \right)} \\ $$$$\frac{\mathrm{1}−{x}}{\left(\mathrm{1}+{x}\right)\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}=\frac{\mathrm{1}}{\mathrm{1}+{x}}−\frac{{x}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left({x}\right)}{\mathrm{1}+{x}}−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left({x}\right).{dx}^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{2}} }=\frac{\mathrm{3}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left({x}\right)}{\mathrm{1}+{x}}{dx} \\ $$$$=−\frac{\mathrm{3}}{\mathrm{2}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left(\mathrm{1}−\left(−{x}\right)\right)}{−{x}}{d}\left(−{x}\right)=\frac{\mathrm{3}}{\mathrm{2}}{Li}_{\mathrm{2}} \left(−\mathrm{1}\right) \\ $$$${A}=\frac{\mathrm{1}}{\mathrm{2}}\left({A}+{B}+{A}−{B}\right) \\ $$$$\frac{{ln}^{\mathrm{2}} \left(\mathrm{2}\right)}{\mathrm{4}}+\frac{{Li}_{\mathrm{2}} \left(−\mathrm{1}\right)}{\mathrm{4}}=\frac{\mathrm{1}}{\mathrm{4}}\left({ln}^{\mathrm{2}} \left(\mathrm{2}\right)+\frac{\pi^{\mathrm{2}} }{\mathrm{12}}\right) \\ $$$$ \\ $$$$ \\ $$

Commented by mnjuly1970 last updated on 02/Aug/21