Menu Close

Question-83767

Tinku Tara 0 Comments
Pin




Question Number 83767 by mr W last updated on 05/Mar/20
Commented by mr W last updated on 06/Mar/20
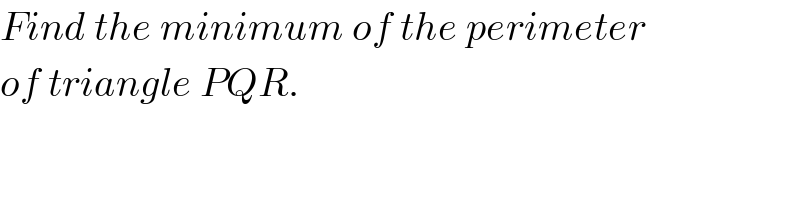
Find the minimum of the perimeter of triangle PQR.
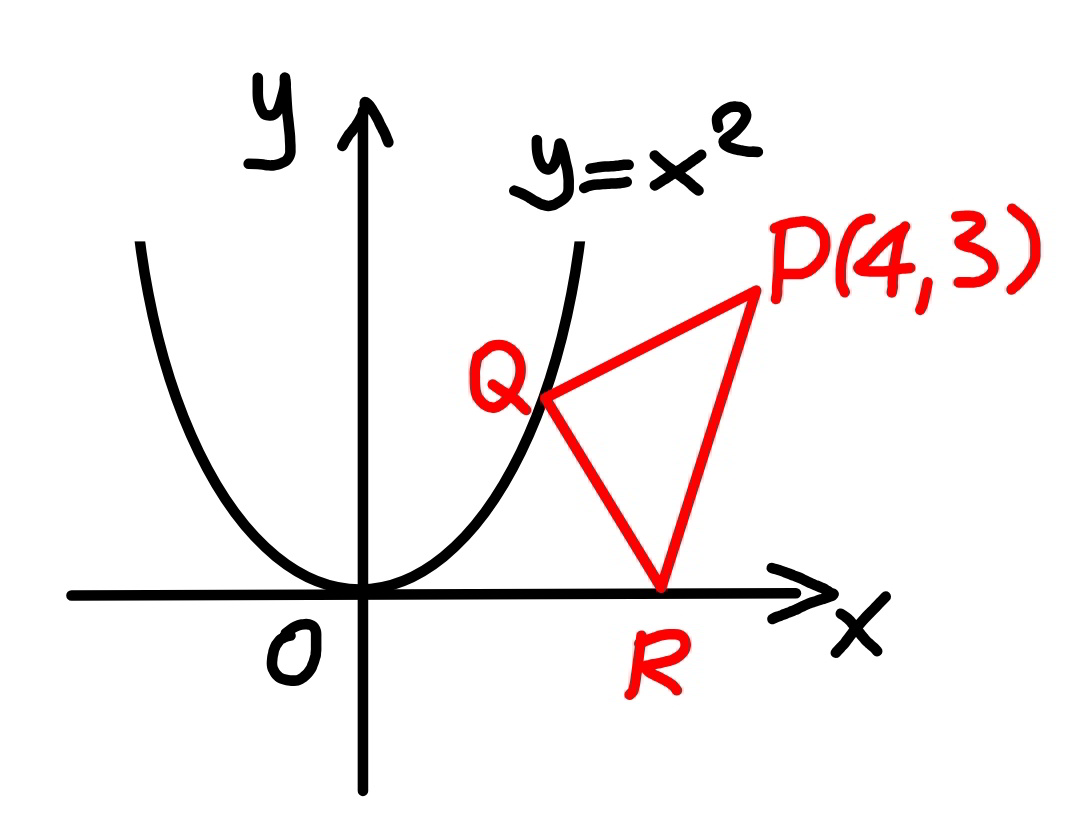
$${Find}\:{the}\:{minimum}\:{of}\:{the}\:{perimeter} \\ $$$${of}\:{triangle}\:{PQR}. \\ $$
Answered by mr W last updated on 06/Mar/20
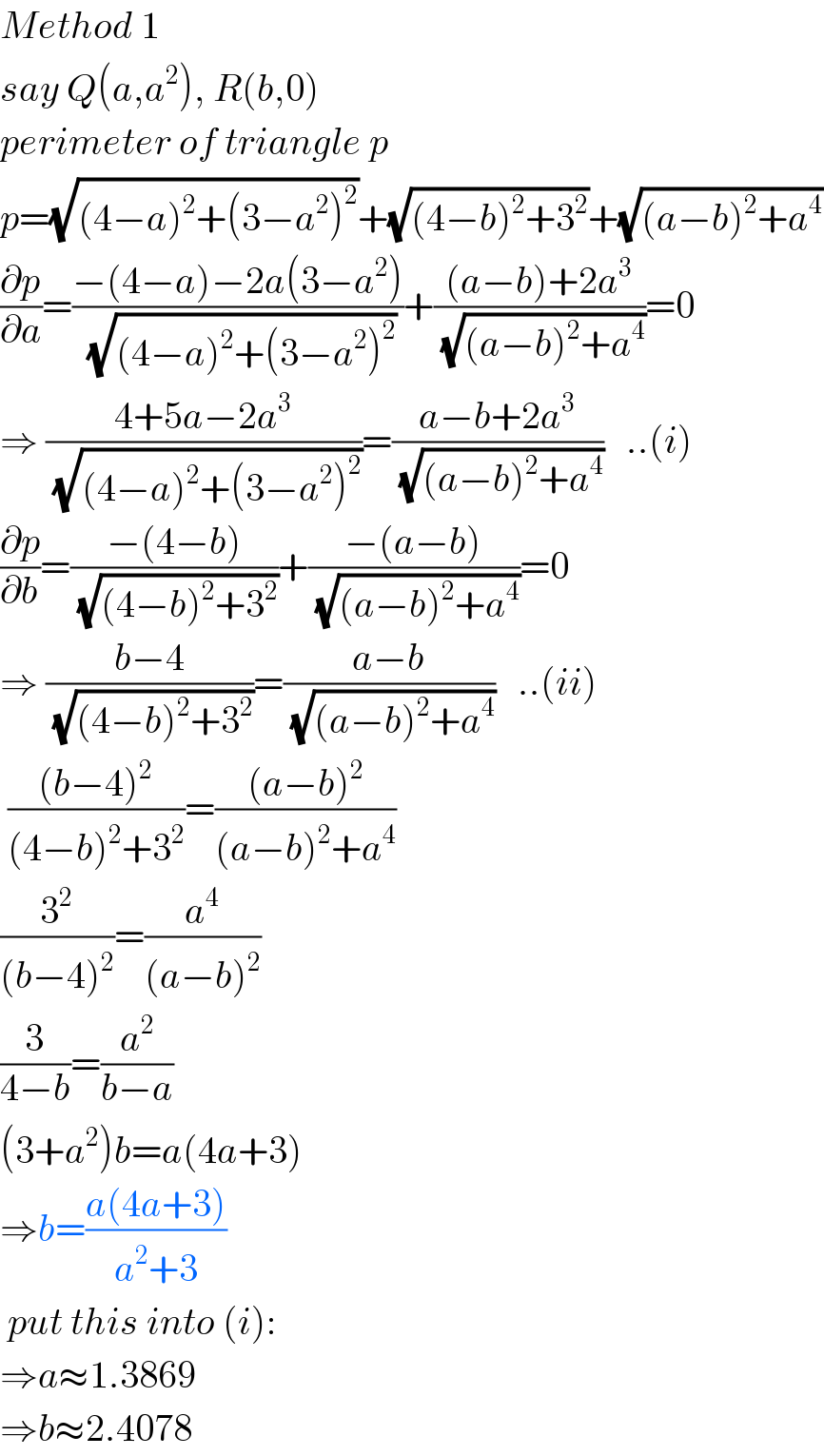
Method 1 say Q(a,a^2 ), R(b,0) perimeter of triangle p p=(√((4−a)^2 +(3−a^2 )^2 ))+(√((4−b)^2 +3^2 ))+(√((a−b)^2 +a^4 )) (∂p/∂a)=((−(4−a)−2a(3−a^2 ))/( (√((4−a)^2 +(3−a^2 )^2 ))))+(((a−b)+2a^3 )/( (√((a−b)^2 +a^4 ))))=0 ⇒ ((4+5a−2a^3 )/( (√((4−a)^2 +(3−a^2 )^2 ))))=((a−b+2a^3 )/( (√((a−b)^2 +a^4 )))) ..(i) (∂p/∂b)=((−(4−b))/( (√((4−b)^2 +3^2 ))))+((−(a−b))/( (√((a−b)^2 +a^4 ))))=0 ⇒ ((b−4)/( (√((4−b)^2 +3^2 ))))=((a−b)/( (√((a−b)^2 +a^4 )))) ..(ii) (((b−4)^2 )/((4−b)^2 +3^2 ))=(((a−b)^2 )/((a−b)^2 +a^4 )) (3^2 /((b−4)^2 ))=(a^4 /((a−b)^2 )) (3/(4−b))=(a^2 /(b−a)) (3+a^2 )b=a(4a+3) ⇒b=((a(4a+3))/(a^2 +3)) put this into (i): ⇒a≈1.3869 ⇒b≈2.4078
$${Method}\:\mathrm{1} \\ $$$${say}\:{Q}\left({a},{a}^{\mathrm{2}} \right),\:{R}\left({b},\mathrm{0}\right) \\ $$$${perimeter}\:{of}\:{triangle}\:{p} \\ $$$${p}=\sqrt{\left(\mathrm{4}−{a}\right)^{\mathrm{2}} +\left(\mathrm{3}−{a}^{\mathrm{2}} \right)^{\mathrm{2}} }+\sqrt{\left(\mathrm{4}−{b}\right)^{\mathrm{2}} +\mathrm{3}^{\mathrm{2}} }+\sqrt{\left({a}−{b}\right)^{\mathrm{2}} +{a}^{\mathrm{4}} } \\ $$$$\frac{\partial{p}}{\partial{a}}=\frac{−\left(\mathrm{4}−{a}\right)−\mathrm{2}{a}\left(\mathrm{3}−{a}^{\mathrm{2}} \right)}{\:\sqrt{\left(\mathrm{4}−{a}\right)^{\mathrm{2}} +\left(\mathrm{3}−{a}^{\mathrm{2}} \right)^{\mathrm{2}} }}+\frac{\left({a}−{b}\right)+\mathrm{2}{a}^{\mathrm{3}} }{\:\sqrt{\left({a}−{b}\right)^{\mathrm{2}} +{a}^{\mathrm{4}} }}=\mathrm{0} \\ $$$$\Rightarrow\:\frac{\mathrm{4}+\mathrm{5}{a}−\mathrm{2}{a}^{\mathrm{3}} }{\:\sqrt{\left(\mathrm{4}−{a}\right)^{\mathrm{2}} +\left(\mathrm{3}−{a}^{\mathrm{2}} \right)^{\mathrm{2}} }}=\frac{{a}−{b}+\mathrm{2}{a}^{\mathrm{3}} }{\:\sqrt{\left({a}−{b}\right)^{\mathrm{2}} +{a}^{\mathrm{4}} }}\:\:\:..\left({i}\right) \\ $$$$\frac{\partial{p}}{\partial{b}}=\frac{−\left(\mathrm{4}−{b}\right)}{\:\sqrt{\left(\mathrm{4}−{b}\right)^{\mathrm{2}} +\mathrm{3}^{\mathrm{2}} }}+\frac{−\left({a}−{b}\right)}{\:\sqrt{\left({a}−{b}\right)^{\mathrm{2}} +{a}^{\mathrm{4}} }}=\mathrm{0} \\ $$$$\Rightarrow\:\frac{{b}−\mathrm{4}}{\:\sqrt{\left(\mathrm{4}−{b}\right)^{\mathrm{2}} +\mathrm{3}^{\mathrm{2}} }}=\frac{{a}−{b}}{\:\sqrt{\left({a}−{b}\right)^{\mathrm{2}} +{a}^{\mathrm{4}} }}\:\:\:..\left({ii}\right) \\ $$$$\:\frac{\left({b}−\mathrm{4}\right)^{\mathrm{2}} }{\left(\mathrm{4}−{b}\right)^{\mathrm{2}} +\mathrm{3}^{\mathrm{2}} }=\frac{\left({a}−{b}\right)^{\mathrm{2}} }{\left({a}−{b}\right)^{\mathrm{2}} +{a}^{\mathrm{4}} } \\ $$$$\frac{\mathrm{3}^{\mathrm{2}} }{\left({b}−\mathrm{4}\right)^{\mathrm{2}} }=\frac{{a}^{\mathrm{4}} }{\left({a}−{b}\right)^{\mathrm{2}} } \\ $$$$\frac{\mathrm{3}}{\mathrm{4}−{b}}=\frac{{a}^{\mathrm{2}} }{{b}−{a}} \\ $$$$\left(\mathrm{3}+{a}^{\mathrm{2}} \right){b}={a}\left(\mathrm{4}{a}+\mathrm{3}\right) \\ $$$$\Rightarrow{b}=\frac{{a}\left(\mathrm{4}{a}+\mathrm{3}\right)}{{a}^{\mathrm{2}} +\mathrm{3}} \\ $$$$\:{put}\:{this}\:{into}\:\left({i}\right): \\ $$$$\Rightarrow{a}\approx\mathrm{1}.\mathrm{3869} \\ $$$$\Rightarrow{b}\approx\mathrm{2}.\mathrm{4078} \\ $$
Commented by jagoll last updated on 06/Mar/20
method 2?
$$\mathrm{method}\:\mathrm{2}? \\ $$
Answered by mr W last updated on 06/Mar/20
Commented by mr W last updated on 06/Mar/20
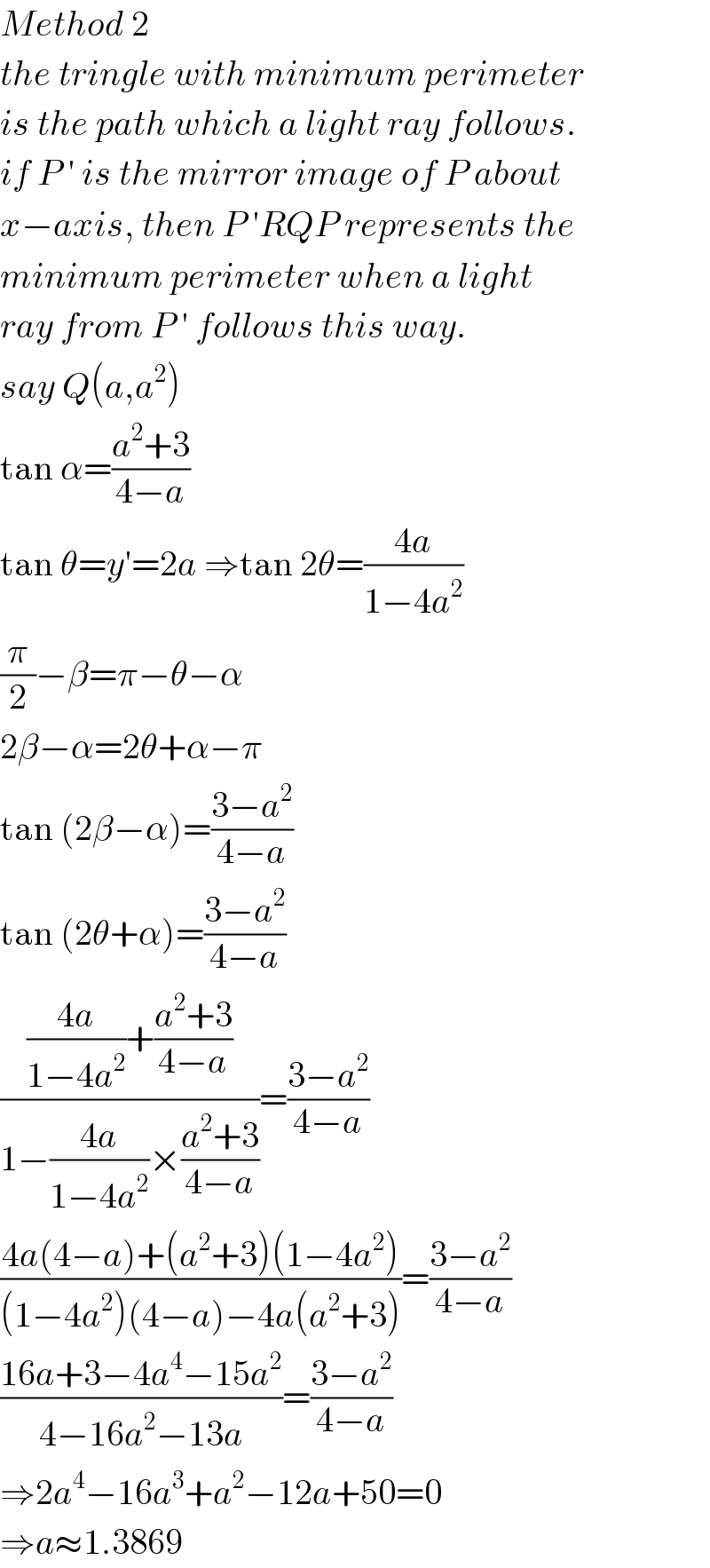
Method 2 the tringle with minimum perimeter is the path which a light ray follows. if P ′ is the mirror image of P about x−axis, then P ′RQP represents the minimum perimeter when a light ray from P ′ follows this way. say Q(a,a^2 ) tan α=((a^2 +3)/(4−a)) tan θ=y′=2a ⇒tan 2θ=((4a)/(1−4a^2 )) (π/2)−β=π−θ−α 2β−α=2θ+α−π tan (2β−α)=((3−a^2 )/(4−a)) tan (2θ+α)=((3−a^2 )/(4−a)) ((((4a)/(1−4a^2 ))+((a^2 +3)/(4−a)))/(1−((4a)/(1−4a^2 ))×((a^2 +3)/(4−a))))=((3−a^2 )/(4−a)) ((4a(4−a)+(a^2 +3)(1−4a^2 ))/((1−4a^2 )(4−a)−4a(a^2 +3)))=((3−a^2 )/(4−a)) ((16a+3−4a^4 −15a^2 )/(4−16a^2 −13a))=((3−a^2 )/(4−a)) ⇒2a^4 −16a^3 +a^2 −12a+50=0 ⇒a≈1.3869
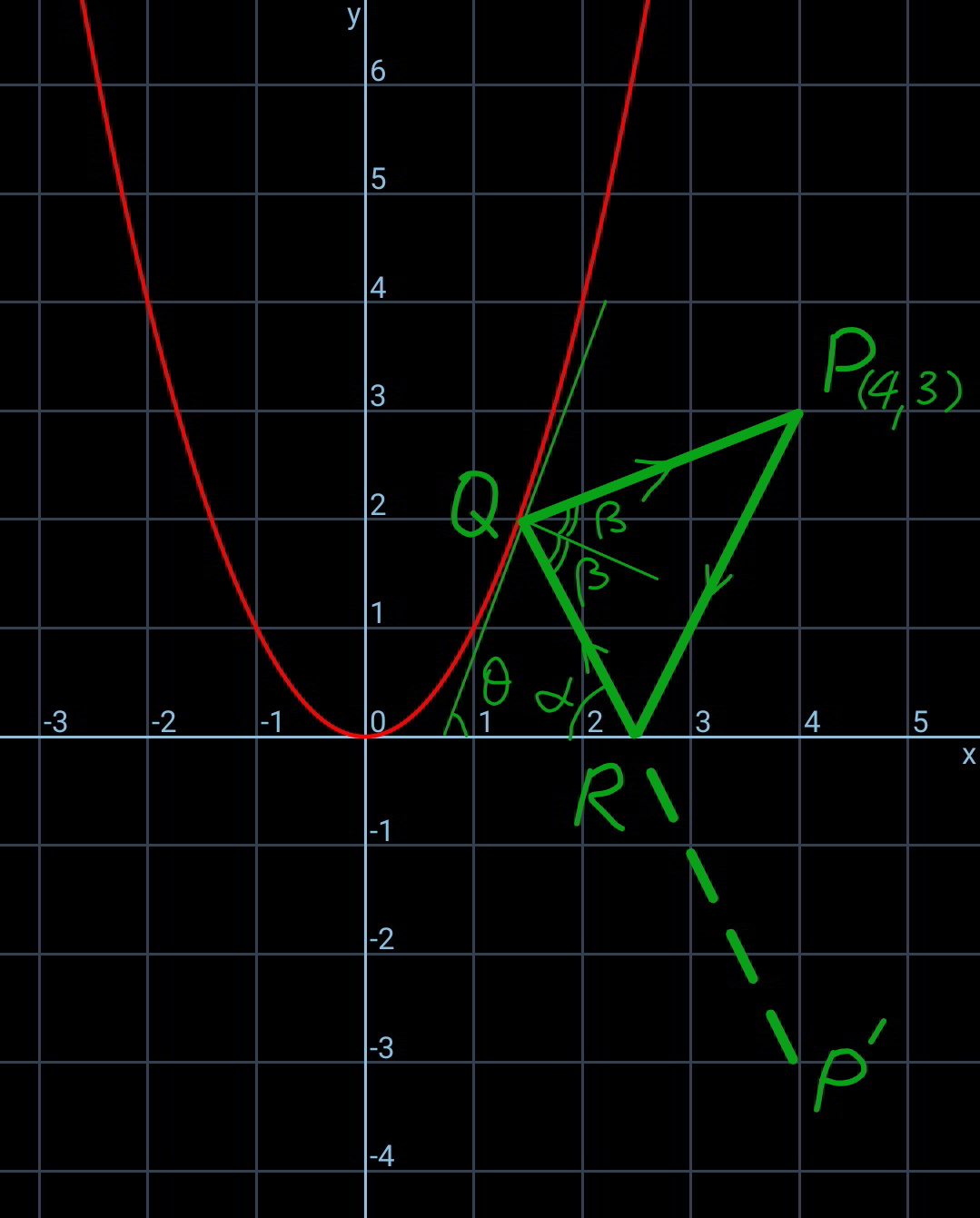
$${Method}\:\mathrm{2} \\ $$$${the}\:{tringle}\:{with}\:{minimum}\:{perimeter} \\ $$$${is}\:{the}\:{path}\:{which}\:{a}\:{light}\:{ray}\:{follows}. \\ $$$${if}\:{P}\:'\:{is}\:{the}\:{mirror}\:{image}\:{of}\:{P}\:{about} \\ $$$${x}−{axis},\:{then}\:{P}\:'{RQP}\:{represents}\:{the} \\ $$$${minimum}\:{perimeter}\:{when}\:{a}\:{light} \\ $$$${ray}\:{from}\:{P}\:'\:{follows}\:{this}\:{way}. \\ $$$${say}\:{Q}\left({a},{a}^{\mathrm{2}} \right) \\ $$$$\mathrm{tan}\:\alpha=\frac{{a}^{\mathrm{2}} +\mathrm{3}}{\mathrm{4}−{a}} \\ $$$$\mathrm{tan}\:\theta={y}'=\mathrm{2}{a}\:\Rightarrow\mathrm{tan}\:\mathrm{2}\theta=\frac{\mathrm{4}{a}}{\mathrm{1}−\mathrm{4}{a}^{\mathrm{2}} } \\ $$$$\frac{\pi}{\mathrm{2}}−\beta=\pi−\theta−\alpha \\ $$$$\mathrm{2}\beta−\alpha=\mathrm{2}\theta+\alpha−\pi \\ $$$$\mathrm{tan}\:\left(\mathrm{2}\beta−\alpha\right)=\frac{\mathrm{3}−{a}^{\mathrm{2}} }{\mathrm{4}−{a}} \\ $$$$\mathrm{tan}\:\left(\mathrm{2}\theta+\alpha\right)=\frac{\mathrm{3}−{a}^{\mathrm{2}} }{\mathrm{4}−{a}} \\ $$$$\frac{\frac{\mathrm{4}{a}}{\mathrm{1}−\mathrm{4}{a}^{\mathrm{2}} }+\frac{{a}^{\mathrm{2}} +\mathrm{3}}{\mathrm{4}−{a}}}{\mathrm{1}−\frac{\mathrm{4}{a}}{\mathrm{1}−\mathrm{4}{a}^{\mathrm{2}} }×\frac{{a}^{\mathrm{2}} +\mathrm{3}}{\mathrm{4}−{a}}}=\frac{\mathrm{3}−{a}^{\mathrm{2}} }{\mathrm{4}−{a}} \\ $$$$\frac{\mathrm{4}{a}\left(\mathrm{4}−{a}\right)+\left({a}^{\mathrm{2}} +\mathrm{3}\right)\left(\mathrm{1}−\mathrm{4}{a}^{\mathrm{2}} \right)}{\left(\mathrm{1}−\mathrm{4}{a}^{\mathrm{2}} \right)\left(\mathrm{4}−{a}\right)−\mathrm{4}{a}\left({a}^{\mathrm{2}} +\mathrm{3}\right)}=\frac{\mathrm{3}−{a}^{\mathrm{2}} }{\mathrm{4}−{a}} \\ $$$$\frac{\mathrm{16}{a}+\mathrm{3}−\mathrm{4}{a}^{\mathrm{4}} −\mathrm{15}{a}^{\mathrm{2}} }{\mathrm{4}−\mathrm{16}{a}^{\mathrm{2}} −\mathrm{13}{a}}=\frac{\mathrm{3}−{a}^{\mathrm{2}} }{\mathrm{4}−{a}} \\ $$$$\Rightarrow\mathrm{2}{a}^{\mathrm{4}} −\mathrm{16}{a}^{\mathrm{3}} +{a}^{\mathrm{2}} −\mathrm{12}{a}+\mathrm{50}=\mathrm{0} \\ $$$$\Rightarrow{a}\approx\mathrm{1}.\mathrm{3869} \\ $$
Commented by jagoll last updated on 06/Mar/20
waw great sir
$$\mathrm{waw}\:\mathrm{great}\:\mathrm{sir} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *