calculate-the-convergence-interval-of-the-serie-n-0-1-n-x-2n-n-

Question Number 150721 by cesarL last updated on 14/Aug/21

calculate the convergence interval of the serie Σ_(n=0) ^∞ (((−1)^n x^(2n) )/(n!))

$${calculate}\:{the}\:{convergence}\:{interval}\:{of}\:{the} \\ $$$${serie} \\ $$$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}} {x}^{\mathrm{2}{n}} }{{n}!} \\ $$

Answered by ArielVyny last updated on 15/Aug/21

Δ=]a−R;a+R[ (convergence inrerval) a=0 R=lim((((−1)^(n+1) )/((n+1)!))×((n!)/((−1)^n )))=lim(−(1/(n+1)))=0 (d′alembert) Δ={0}

$$\left.\Delta=\right]{a}−{R};{a}+{R}\left[\:\left({convergence}\:{inrerval}\right)\:\:\:{a}=\mathrm{0}\:\:{R}={lim}\left(\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\left({n}+\mathrm{1}\right)!}×\frac{{n}!}{\left(−\mathrm{1}\right)^{{n}} }\right)={lim}\left(−\frac{\mathrm{1}}{{n}+\mathrm{1}}\right)=\mathrm{0}\:\left({d}'{alembert}\right)\right. \\ $$$$\Delta=\left\{\mathrm{0}\right\} \\ $$

Answered by mathmax by abdo last updated on 17/Aug/21

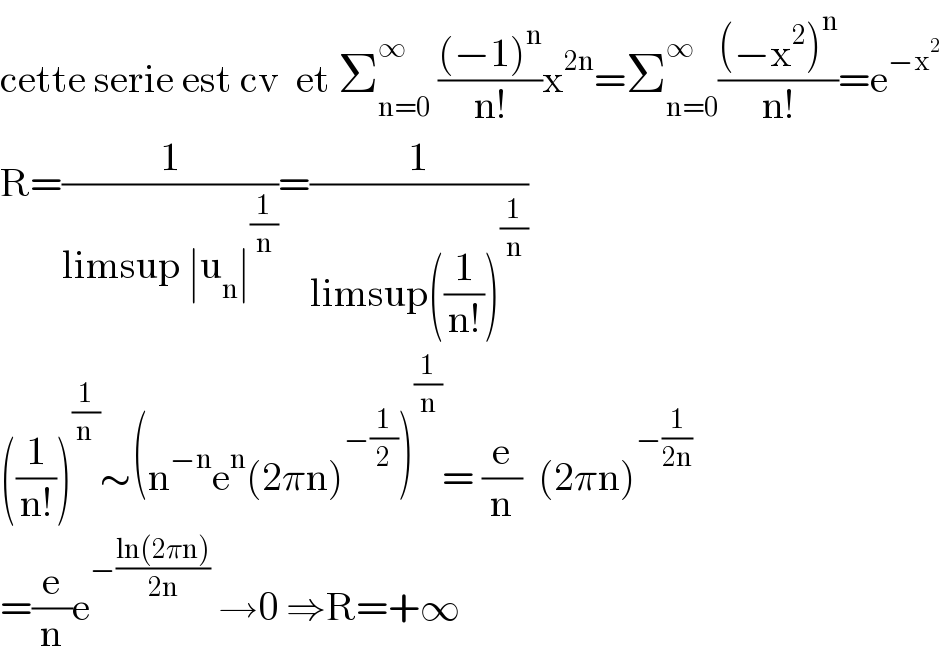
cette serie est cv et Σ_(n=0) ^∞ (((−1)^n )/(n!))x^(2n) =Σ_(n=0) ^∞ (((−x^2 )^n )/(n!))=e^(−x^2 ) R=(1/(limsup ∣u_n ∣^(1/n) ))=(1/(limsup((1/(n!)))^(1/n) )) ((1/(n!)))^(1/(n )) ∼(n^(−n) e^n (2πn)^(−(1/2)) )^(1/n) = (e/n) (2πn)^(−(1/(2n))) =(e/n)e^(−((ln(2πn))/(2n))) →0 ⇒R=+∞

$$\mathrm{cette}\:\mathrm{serie}\:\mathrm{est}\:\mathrm{cv}\:\:\mathrm{et}\:\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{\mathrm{n}} }{\mathrm{n}!}\mathrm{x}^{\mathrm{2n}} =\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \frac{\left(−\mathrm{x}^{\mathrm{2}} \right)^{\mathrm{n}} }{\mathrm{n}!}=\mathrm{e}^{−\mathrm{x}^{\mathrm{2}} } \\ $$$$\mathrm{R}=\frac{\mathrm{1}}{\mathrm{limsup}\:\mid\mathrm{u}_{\mathrm{n}} \mid^{\frac{\mathrm{1}}{\mathrm{n}}} }=\frac{\mathrm{1}}{\mathrm{limsup}\left(\frac{\mathrm{1}}{\mathrm{n}!}\right)^{\frac{\mathrm{1}}{\mathrm{n}}} } \\ $$$$\left(\frac{\mathrm{1}}{\mathrm{n}!}\right)^{\frac{\mathrm{1}}{\mathrm{n}\:}} \sim\left(\mathrm{n}^{−\mathrm{n}} \mathrm{e}^{\mathrm{n}} \left(\mathrm{2}\pi\mathrm{n}\right)^{−\frac{\mathrm{1}}{\mathrm{2}}} \right)^{\frac{\mathrm{1}}{\mathrm{n}}} =\:\frac{\mathrm{e}}{\mathrm{n}}\:\:\left(\mathrm{2}\pi\mathrm{n}\right)^{−\frac{\mathrm{1}}{\mathrm{2n}}} \\ $$$$=\frac{\mathrm{e}}{\mathrm{n}}\mathrm{e}^{−\frac{\mathrm{ln}\left(\mathrm{2}\pi\mathrm{n}\right)}{\mathrm{2n}}} \:\rightarrow\mathrm{0}\:\Rightarrow\mathrm{R}=+\infty \\ $$

Facebook Tweet Pin

calculate-the-convergence-interval-of-the-serie-n-0-1-n-x-2n-n-

Leave a Reply Cancel reply