Question Number 85865 by subhankar10 last updated on 25/Mar/20
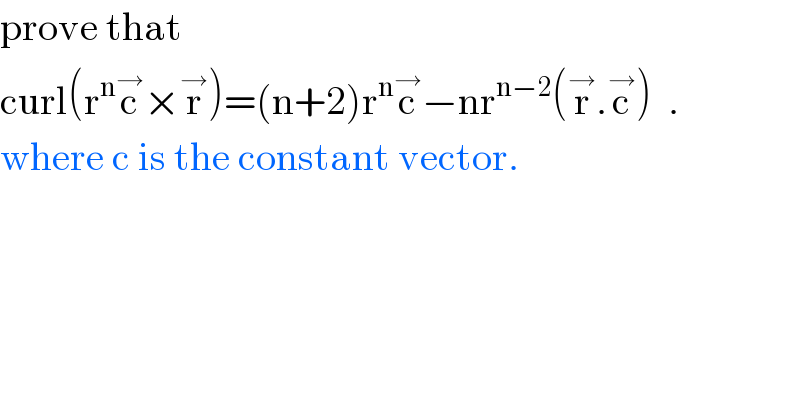
$$\mathrm{prove}\:\mathrm{that}\: \\ $$$$\mathrm{curl}\left(\mathrm{r}^{\mathrm{n}} \overset{\rightarrow} {\mathrm{c}}×\overset{\rightarrow} {\mathrm{r}}\right)=\left(\mathrm{n}+\mathrm{2}\right)\mathrm{r}^{\mathrm{n}} \overset{\rightarrow} {\mathrm{c}}−\mathrm{nr}^{\mathrm{n}−\mathrm{2}} \left(\overset{\rightarrow} {\mathrm{r}}.\overset{\rightarrow} {\mathrm{c}}\right)\:\:. \\ $$$$\mathrm{where}\:\mathrm{c}\:\mathrm{is}\:\mathrm{the}\:\mathrm{constant}\:\mathrm{vector}. \\ $$
Answered by TANMAY PANACEA. last updated on 25/Mar/20
![r^→ =ix+jy+kz→r^2 =x^2 +y^2 +z^2 r^n =(x^2 +y^2 +z^2 )^(n/2) c^→ =ia+ib+kd curlA^→ =▽^→ ×A^→ (i(∂/∂x)+j(∂/∂y)+k(∂/∂z))×A^→ now ▽^→ ×(c^→ ×r^n r^→ ) =(▽^→ .r^n r^→ )c^→ −(▽^→ .c^→ )r^n r^→ =c^→ (i(∂/∂x)+j(∂/∂y)+k(∂/∂z)).(x^2 +y^2 +z^2 )^(n/2) (ix+jy+kz) =c^→ [(∂/∂x){x.(x^2 +y^2 +z^2 )^(n/2) }+(∂/∂y){y(x^2 +y^2 +z^2 )}+(∂/∂z){z(x^2 +y^2 +z^2 )}] calculation of (∂/∂x){x(x^2 +y^2 +z^2 )^(n/2) } =(x^2 +y^2 +z^2 )^(n/2) ×1+x×(n/2)(x^2 +y^2 +z^2 )^((n/2)−1) ×2x =r^n +x^2 ×n(r^2 )^((n/2)−1) =r^n +x^2 ×n×r^(n−2) adding three 3r^n +nr^(n−2) (x^2 +y^2 +z^2 ) =3r^n +nr^n =r^n (n+3) so answer is r^n (n+3)c^→ timorrow i shall solve in paper .=.. c^→ ×r^→ ∣i j k ∣ ∣a b d ∣ ∣x y z ∣ =i(bz−yd)−j(az−xd)+k(ay−bx) now ∣i j](https://www.tinkutara.com/question/Q85889.png)
$$\overset{\rightarrow} {{r}}={ix}+{jy}+{kz}\rightarrow{r}^{\mathrm{2}} ={x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \\ $$$${r}^{{n}} =\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \right)^{\frac{{n}}{\mathrm{2}}} \\ $$$$\overset{\rightarrow} {{c}}={ia}+{ib}+{kd} \\ $$$${curl}\overset{\rightarrow} {{A}}=\overset{\rightarrow} {\bigtriangledown}×\overset{\rightarrow} {{A}} \\ $$$$\left({i}\frac{\partial}{\partial{x}}+{j}\frac{\partial}{\partial{y}}+{k}\frac{\partial}{\partial{z}}\right)×\overset{\rightarrow} {{A}} \\ $$$${now} \\ $$$$\overset{\rightarrow} {\bigtriangledown}×\left(\overset{\rightarrow} {{c}}×{r}^{{n}} \overset{\rightarrow} {{r}}\right) \\ $$$$=\left(\overset{\rightarrow} {\bigtriangledown}.{r}^{{n}} \overset{\rightarrow} {{r}}\right)\overset{\rightarrow} {{c}}−\left(\overset{\rightarrow} {\bigtriangledown}.\overset{\rightarrow} {{c}}\right){r}^{{n}} \overset{\rightarrow} {{r}} \\ $$$$=\overset{\rightarrow} {{c}}\left({i}\frac{\partial}{\partial{x}}+{j}\frac{\partial}{\partial{y}}+{k}\frac{\partial}{\partial{z}}\right).\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \right)^{\frac{{n}}{\mathrm{2}}} \left({ix}+{jy}+{kz}\right) \\ $$$$=\overset{\rightarrow} {{c}}\left[\frac{\partial}{\partial{x}}\left\{{x}.\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \right)^{\frac{{n}}{\mathrm{2}}} \right\}+\frac{\partial}{\partial{y}}\left\{{y}\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \right)\right\}+\frac{\partial}{\partial{z}}\left\{{z}\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \right)\right\}\right] \\ $$$$\boldsymbol{{calculation}}\:\boldsymbol{{of}} \\ $$$$\frac{\partial}{\partial{x}}\left\{{x}\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \right)^{\frac{{n}}{\mathrm{2}}} \right\} \\ $$$$=\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \right)^{\frac{{n}}{\mathrm{2}}} ×\mathrm{1}+{x}×\frac{{n}}{\mathrm{2}}\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \right)^{\frac{{n}}{\mathrm{2}}−\mathrm{1}} ×\mathrm{2}{x} \\ $$$$={r}^{{n}} +{x}^{\mathrm{2}} ×{n}\left({r}^{\mathrm{2}} \right)^{\frac{{n}}{\mathrm{2}}−\mathrm{1}} \\ $$$$={r}^{{n}} +{x}^{\mathrm{2}} ×{n}×{r}^{{n}−\mathrm{2}} \\ $$$${adding}\:{three} \\ $$$$\mathrm{3}{r}^{{n}} +{nr}^{{n}−\mathrm{2}} \left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \right) \\ $$$$=\mathrm{3}{r}^{{n}} +{nr}^{{n}} ={r}^{{n}} \left({n}+\mathrm{3}\right) \\ $$$$\boldsymbol{{so}}\:\boldsymbol{{answer}}\:\boldsymbol{{is}}\:\:\:\:\:\:{r}^{{n}} \left({n}+\mathrm{3}\right)\overset{\rightarrow} {\boldsymbol{{c}}} \\ $$$${timorrow}\:{i}\:{shall}\:{solve}\:{in}\:{paper} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$ \\ $$$$.=.. \\ $$$$\overset{\rightarrow} {{c}}×\overset{\rightarrow} {{r}} \\ $$$$\mid{i}\:\:\:\:\:\:\:\:{j}\:\:\:\:\:\:\:{k}\:\:\:\:\mid \\ $$$$\mid{a}\:\:\:\:\:\:\:\:{b}\:\:\:\:\:\:\:{d}\:\:\:\:\mid \\ $$$$\mid{x}\:\:\:\:\:\:\:\:{y}\:\:\:\:\:\:\:{z}\:\:\:\:\mid \\ $$$$={i}\left({bz}−{yd}\right)−{j}\left({az}−{xd}\right)+{k}\left({ay}−{bx}\right) \\ $$$${now} \\ $$$$\mid{i}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{j}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$
