Question Number 21234 by Tinkutara last updated on 17/Sep/17
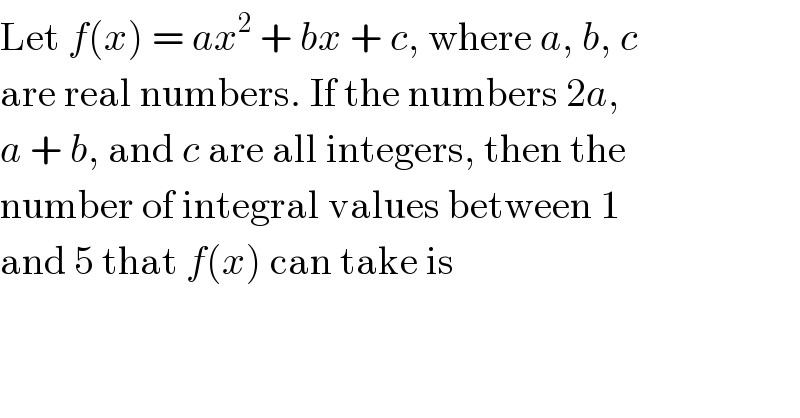
$$\mathrm{Let}\:{f}\left({x}\right)\:=\:{ax}^{\mathrm{2}} \:+\:{bx}\:+\:{c},\:\mathrm{where}\:{a},\:{b},\:{c} \\ $$$$\mathrm{are}\:\mathrm{real}\:\mathrm{numbers}.\:\mathrm{If}\:\mathrm{the}\:\mathrm{numbers}\:\mathrm{2}{a}, \\ $$$${a}\:+\:{b},\:\mathrm{and}\:{c}\:\mathrm{are}\:\mathrm{all}\:\mathrm{integers},\:\mathrm{then}\:\mathrm{the} \\ $$$$\mathrm{number}\:\mathrm{of}\:\mathrm{integral}\:\mathrm{values}\:\mathrm{between}\:\mathrm{1} \\ $$$$\mathrm{and}\:\mathrm{5}\:\mathrm{that}\:{f}\left({x}\right)\:\mathrm{can}\:\mathrm{take}\:\mathrm{is} \\ $$
Answered by Tinkutara last updated on 17/Sep/17
![f(0)=c∈Z f(1)=a+b+c f(−1)=a−b+c Since 2a,a+b,c are integers, so 2a−(a+b)+c=a−b+c∈Z⇒f(−1)∈Z Similarly (a+b)+c=f(1)∈Z. f(1)−f(−1)=2b∈Z f(2)=4a+2b+c∈Z f(3)=9a+3b+c=a+b+c+8a+2b∈Z f(4)=16a+4b+c∈Z f(5)=25a+5b+c=a+b+c+24a+4b∈Z ∴ f(x) has 5 integral values in [1,5].](https://www.tinkutara.com/question/Q21243.png)
$${f}\left(\mathrm{0}\right)={c}\in{Z} \\ $$$${f}\left(\mathrm{1}\right)={a}+{b}+{c} \\ $$$${f}\left(−\mathrm{1}\right)={a}−{b}+{c} \\ $$$${Since}\:\mathrm{2}{a},{a}+{b},{c}\:{are}\:{integers},\:{so} \\ $$$$\mathrm{2}{a}−\left({a}+{b}\right)+{c}={a}−{b}+{c}\in{Z}\Rightarrow{f}\left(−\mathrm{1}\right)\in{Z} \\ $$$${Similarly}\:\left({a}+{b}\right)+{c}={f}\left(\mathrm{1}\right)\in{Z}. \\ $$$${f}\left(\mathrm{1}\right)−{f}\left(−\mathrm{1}\right)=\mathrm{2}{b}\in{Z} \\ $$$${f}\left(\mathrm{2}\right)=\mathrm{4}{a}+\mathrm{2}{b}+{c}\in{Z} \\ $$$${f}\left(\mathrm{3}\right)=\mathrm{9}{a}+\mathrm{3}{b}+{c}={a}+{b}+{c}+\mathrm{8}{a}+\mathrm{2}{b}\in{Z} \\ $$$${f}\left(\mathrm{4}\right)=\mathrm{16}{a}+\mathrm{4}{b}+{c}\in{Z} \\ $$$${f}\left(\mathrm{5}\right)=\mathrm{25}{a}+\mathrm{5}{b}+{c}={a}+{b}+{c}+\mathrm{24}{a}+\mathrm{4}{b}\in{Z} \\ $$$$\therefore\:{f}\left({x}\right)\:{has}\:\mathrm{5}\:{integral}\:{values}\:{in}\:\left[\mathrm{1},\mathrm{5}\right]. \\ $$
