Question Number 133027 by Engr_Jidda last updated on 18/Feb/21
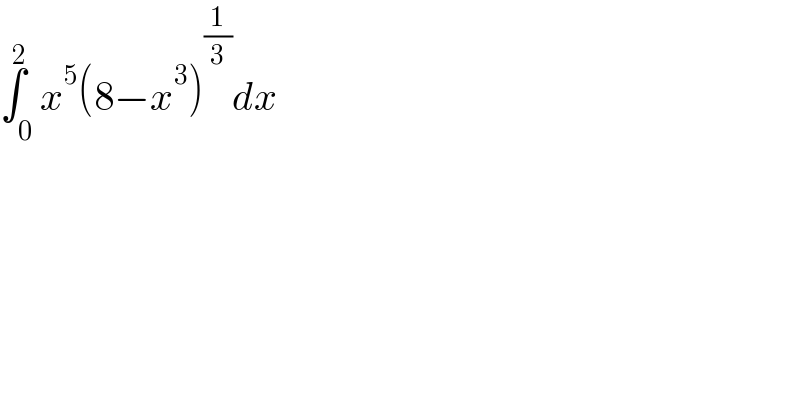
$$\overset{\mathrm{2}} {\int}_{\mathrm{0}} {x}^{\mathrm{5}} \left(\mathrm{8}−{x}^{\mathrm{3}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} {dx} \\ $$
Answered by Olaf last updated on 18/Feb/21
![Ω = ∫_0 ^2 x^5 (8−x^3 )^(1/3) dx Ω = ∫_0 ^2 (−(1/4)x^3 )[−4x^2 (8−x^3 )^(1/3) ]dx Ω = [(−(1/4)x^3 )(8−x^3 )^(4/3) ]_0 ^2 −∫_0 ^2 (−(3/4)x^2 )(8−x^3 )^(4/3) dx Ω = (3/4)∫_0 ^2 x^2 (8−x^3 )^(4/3) dx Ω = (3/4)∫_0 ^2 (−(1/7))[−7x^2 (8−x^3 )^(4/3) ]dx Ω = −(3/(4×7))[(8−x^3 )^(7/3) ]_0 ^2 Ω = ((3×8^(7/3) )/(4×7)) = ((3×2^7 )/(7×2^2 )) = ((96)/7)](https://www.tinkutara.com/question/Q133035.png)
$$\Omega\:=\:\int_{\mathrm{0}} ^{\mathrm{2}} {x}^{\mathrm{5}} \left(\mathrm{8}−{x}^{\mathrm{3}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} {dx} \\ $$$$\Omega\:=\:\int_{\mathrm{0}} ^{\mathrm{2}} \left(−\frac{\mathrm{1}}{\mathrm{4}}{x}^{\mathrm{3}} \right)\left[−\mathrm{4}{x}^{\mathrm{2}} \left(\mathrm{8}−{x}^{\mathrm{3}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} \right]{dx} \\ $$$$\Omega\:=\:\left[\left(−\frac{\mathrm{1}}{\mathrm{4}}{x}^{\mathrm{3}} \right)\left(\mathrm{8}−{x}^{\mathrm{3}} \right)^{\frac{\mathrm{4}}{\mathrm{3}}} \right]_{\mathrm{0}} ^{\mathrm{2}} −\int_{\mathrm{0}} ^{\mathrm{2}} \left(−\frac{\mathrm{3}}{\mathrm{4}}{x}^{\mathrm{2}} \right)\left(\mathrm{8}−{x}^{\mathrm{3}} \right)^{\frac{\mathrm{4}}{\mathrm{3}}} {dx} \\ $$$$\Omega\:=\:\frac{\mathrm{3}}{\mathrm{4}}\int_{\mathrm{0}} ^{\mathrm{2}} {x}^{\mathrm{2}} \left(\mathrm{8}−{x}^{\mathrm{3}} \right)^{\frac{\mathrm{4}}{\mathrm{3}}} {dx} \\ $$$$\Omega\:=\:\frac{\mathrm{3}}{\mathrm{4}}\int_{\mathrm{0}} ^{\mathrm{2}} \left(−\frac{\mathrm{1}}{\mathrm{7}}\right)\left[−\mathrm{7}{x}^{\mathrm{2}} \left(\mathrm{8}−{x}^{\mathrm{3}} \right)^{\frac{\mathrm{4}}{\mathrm{3}}} \right]{dx} \\ $$$$\Omega\:=\:−\frac{\mathrm{3}}{\mathrm{4}×\mathrm{7}}\left[\left(\mathrm{8}−{x}^{\mathrm{3}} \right)^{\frac{\mathrm{7}}{\mathrm{3}}} \right]_{\mathrm{0}} ^{\mathrm{2}} \\ $$$$\Omega\:=\:\frac{\mathrm{3}×\mathrm{8}^{\frac{\mathrm{7}}{\mathrm{3}}} }{\mathrm{4}×\mathrm{7}}\:=\:\frac{\mathrm{3}×\mathrm{2}^{\mathrm{7}} }{\mathrm{7}×\mathrm{2}^{\mathrm{2}} }\:=\:\frac{\mathrm{96}}{\mathrm{7}} \\ $$
Commented by Engr_Jidda last updated on 22/Feb/21

$${thank}\:{you}\:{sir} \\ $$
Answered by liberty last updated on 18/Feb/21
![I=∫^( 2) _0 x^3 .x^2 ((8−x^3 ))^(1/3) dx change of variable put u^3 = 8−x^3 where { ((for x=2→u=0)),((for x=0→u=2)) :} x^2 dx = −u^2 du I=∫_2 ^0 (8−u^3 )u (−u^2 du) I=∫_2 ^0 (u^6 −8u^3 )du I=(u^7 /7)−2u^4 ]_2 ^0 = u^4 ((u^3 /7)−2)]_2 ^0 I=−16((8/7)−2)=−16(−(6/( 7))) I=((96)/7) ≈ 13.714286](https://www.tinkutara.com/question/Q133039.png)
$$\mathrm{I}=\underset{\mathrm{0}} {\int}^{\:\mathrm{2}} \mathrm{x}^{\mathrm{3}} \:.\mathrm{x}^{\mathrm{2}} \:\sqrt[{\mathrm{3}}]{\mathrm{8}−\mathrm{x}^{\mathrm{3}} }\:\mathrm{dx}\: \\ $$$$\mathrm{change}\:\mathrm{of}\:\mathrm{variable}\: \\ $$$$\:\mathrm{put}\:\mathrm{u}^{\mathrm{3}} \:=\:\mathrm{8}−\mathrm{x}^{\mathrm{3}} \:\mathrm{where}\:\begin{cases}{\mathrm{for}\:\mathrm{x}=\mathrm{2}\rightarrow\mathrm{u}=\mathrm{0}}\\{\mathrm{for}\:\mathrm{x}=\mathrm{0}\rightarrow\mathrm{u}=\mathrm{2}}\end{cases} \\ $$$$\:\mathrm{x}^{\mathrm{2}} \:\mathrm{dx}\:=\:−\mathrm{u}^{\mathrm{2}} \:\mathrm{du}\: \\ $$$$\mathrm{I}=\underset{\mathrm{2}} {\overset{\mathrm{0}} {\int}}\left(\mathrm{8}−\mathrm{u}^{\mathrm{3}} \right)\mathrm{u}\:\left(−\mathrm{u}^{\mathrm{2}} \:\mathrm{du}\right) \\ $$$$\mathrm{I}=\underset{\mathrm{2}} {\overset{\mathrm{0}} {\int}}\left(\mathrm{u}^{\mathrm{6}} −\mathrm{8u}^{\mathrm{3}} \right)\mathrm{du}\: \\ $$$$\left.\mathrm{I}\left.=\frac{\mathrm{u}^{\mathrm{7}} }{\mathrm{7}}−\mathrm{2u}^{\mathrm{4}} \:\right]_{\mathrm{2}} ^{\mathrm{0}} \:=\:\mathrm{u}^{\mathrm{4}} \left(\frac{\mathrm{u}^{\mathrm{3}} }{\mathrm{7}}−\mathrm{2}\right)\right]_{\mathrm{2}} ^{\mathrm{0}} \\ $$$$\mathrm{I}=−\mathrm{16}\left(\frac{\mathrm{8}}{\mathrm{7}}−\mathrm{2}\right)=−\mathrm{16}\left(−\frac{\mathrm{6}}{\:\mathrm{7}}\right) \\ $$$$\mathrm{I}=\frac{\mathrm{96}}{\mathrm{7}}\:\approx\:\mathrm{13}.\mathrm{714286} \\ $$
Commented by liberty last updated on 18/Feb/21

Commented by EDWIN88 last updated on 18/Feb/21

Commented by Engr_Jidda last updated on 22/Feb/21

$${thank}\:{you}\:{sir} \\ $$
Answered by Dwaipayan Shikari last updated on 18/Feb/21

$$\int_{\mathrm{0}} ^{\mathrm{2}} {x}^{\mathrm{5}} \left(\mathrm{8}−{x}^{\mathrm{3}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} {dx}\:\:\:\:\:\:\:{x}^{\mathrm{3}} =\mathrm{8}{u}\Rightarrow\mathrm{3}{x}^{\mathrm{2}} =\mathrm{8}\frac{{du}}{{dx}} \\ $$$$=\frac{\mathrm{16}}{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{8}{u}\left(\mathrm{1}−{u}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} {du}=\frac{\mathrm{128}}{\mathrm{3}}.\frac{\Gamma\left(\mathrm{2}\right)\Gamma\left(\frac{\mathrm{4}}{\mathrm{3}}\right)}{\Gamma\left(\frac{\mathrm{10}}{\mathrm{3}}\right)}=\mathrm{128}.\frac{\Gamma\left(\frac{\mathrm{4}}{\mathrm{3}}\right)}{\mathrm{7}\Gamma\left(\frac{\mathrm{7}}{\mathrm{3}}\right)} \\ $$$$=\frac{\mathrm{128}}{\mathrm{7}}.\frac{\mathrm{3}}{\mathrm{4}}=\frac{\mathrm{96}}{\mathrm{7}} \\ $$
Commented by Engr_Jidda last updated on 22/Feb/21

$${thank}\:{you}\:{sir} \\ $$
Answered by mathmax by abdo last updated on 20/Feb/21
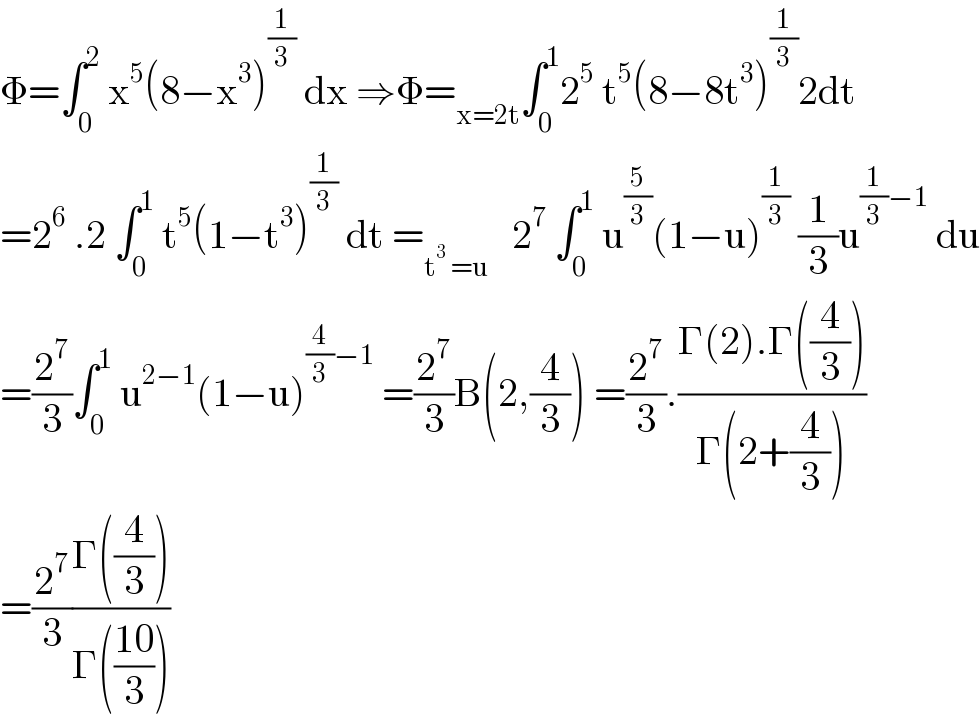
$$\Phi=\int_{\mathrm{0}} ^{\mathrm{2}} \:\mathrm{x}^{\mathrm{5}} \left(\mathrm{8}−\mathrm{x}^{\mathrm{3}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:\mathrm{dx}\:\Rightarrow\Phi=_{\mathrm{x}=\mathrm{2t}} \int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{2}^{\mathrm{5}} \:\mathrm{t}^{\mathrm{5}} \left(\mathrm{8}−\mathrm{8t}^{\mathrm{3}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} \mathrm{2dt} \\ $$$$=\mathrm{2}^{\mathrm{6}} \:.\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{t}^{\mathrm{5}} \left(\mathrm{1}−\mathrm{t}^{\mathrm{3}} \right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:\mathrm{dt}\:=_{\mathrm{t}^{\mathrm{3}} \:=\mathrm{u}} \:\:\:\mathrm{2}^{\mathrm{7}} \:\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{u}^{\frac{\mathrm{5}}{\mathrm{3}}} \left(\mathrm{1}−\mathrm{u}\right)^{\frac{\mathrm{1}}{\mathrm{3}}} \:\frac{\mathrm{1}}{\mathrm{3}}\mathrm{u}^{\frac{\mathrm{1}}{\mathrm{3}}−\mathrm{1}} \:\mathrm{du} \\ $$$$=\frac{\mathrm{2}^{\mathrm{7}} }{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{u}^{\mathrm{2}−\mathrm{1}} \left(\mathrm{1}−\mathrm{u}\right)^{\frac{\mathrm{4}}{\mathrm{3}}−\mathrm{1}} \:=\frac{\mathrm{2}^{\mathrm{7}} }{\mathrm{3}}\mathrm{B}\left(\mathrm{2},\frac{\mathrm{4}}{\mathrm{3}}\right)\:=\frac{\mathrm{2}^{\mathrm{7}} }{\mathrm{3}}.\frac{\Gamma\left(\mathrm{2}\right).\Gamma\left(\frac{\mathrm{4}}{\mathrm{3}}\right)}{\Gamma\left(\mathrm{2}+\frac{\mathrm{4}}{\mathrm{3}}\right)} \\ $$$$=\frac{\mathrm{2}^{\mathrm{7}} }{\mathrm{3}}\frac{\Gamma\left(\frac{\mathrm{4}}{\mathrm{3}}\right)}{\Gamma\left(\frac{\mathrm{10}}{\mathrm{3}}\right)} \\ $$
Commented by Engr_Jidda last updated on 22/Feb/21

$${thank}\:{you}\:{sir} \\ $$
