Menu Close

0-1-ln-x-3-1-x-1-dx-

Tinku Tara 0 Comments
Pin




Question Number 153151 by puissant last updated on 05/Sep/21
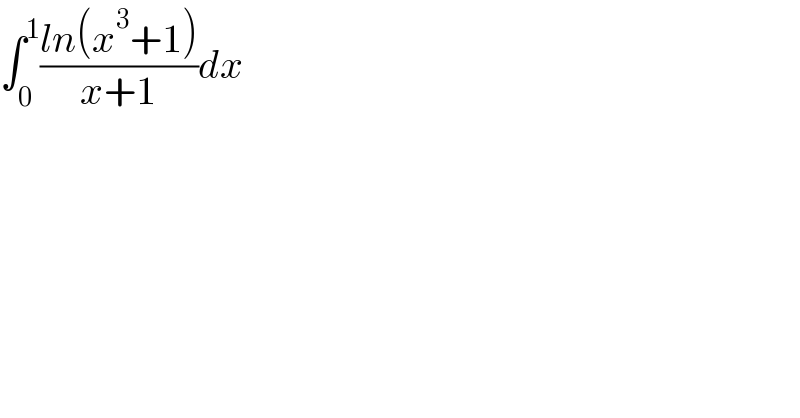
∫_0 ^1 ((ln(x^3 +1))/(x+1))dx
$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left({x}^{\mathrm{3}} +\mathrm{1}\right)}{{x}+\mathrm{1}}{dx} \\ $$
Answered by Ar Brandon last updated on 05/Sep/21
I=∫_0 ^1 ((ln(x^3 +1))/(x+1))dx=∫_0 ^1 Σ_(n≥1) (((−1)^(n+1) )/n)∙(x^(3n) /(1+x))dx =Σ_(n≥1) (((−1)^(n+1) )/n)∫_0 ^1 ((x^(3n) −x^(3n+1) )/(1−x^2 ))dx, x=u^(1/2) ⇒dx=(1/2)u^(−(1/2)) du =Σ_(n≥1) (((−1)^(n+1) )/(2n))∫_0 ^1 ((u^((3n−1)/2) −u^((3n)/2) )/(1−u))du=Σ_(n≥1) (((−1)^(n+1) )/(2n))(ψ(((3n)/2)+1)−ψ(((3n+1)/2)))
$${I}=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{ln}\left({x}^{\mathrm{3}} +\mathrm{1}\right)}{{x}+\mathrm{1}}{dx}=\int_{\mathrm{0}} ^{\mathrm{1}} \underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{{n}}\centerdot\frac{{x}^{\mathrm{3}{n}} }{\mathrm{1}+{x}}{dx} \\ $$$$\:\:=\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{{n}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{x}^{\mathrm{3}{n}} −{x}^{\mathrm{3}{n}+\mathrm{1}} }{\mathrm{1}−{x}^{\mathrm{2}} }{dx},\:{x}={u}^{\frac{\mathrm{1}}{\mathrm{2}}} \Rightarrow{dx}=\frac{\mathrm{1}}{\mathrm{2}}{u}^{−\frac{\mathrm{1}}{\mathrm{2}}} {du} \\ $$$$\:\:=\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\mathrm{2}{n}}\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{u}^{\frac{\mathrm{3}{n}−\mathrm{1}}{\mathrm{2}}} −{u}^{\frac{\mathrm{3}{n}}{\mathrm{2}}} }{\mathrm{1}−{u}}{du}=\underset{{n}\geqslant\mathrm{1}} {\sum}\frac{\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} }{\mathrm{2}{n}}\left(\psi\left(\frac{\mathrm{3}{n}}{\mathrm{2}}+\mathrm{1}\right)−\psi\left(\frac{\mathrm{3}{n}+\mathrm{1}}{\mathrm{2}}\right)\right) \\ $$
Commented by puissant last updated on 05/Sep/21
parfait..
$${parfait}.. \\ $$
Answered by mindispower last updated on 05/Sep/21
=ln(x^3 +1)=ln((x+1)(x^2 −x+1)) =(1/2)ln^2 (2)+∫_0 ^1 ((ln(x^2 −x+1))/(x+1))dx =((ln^2 (2))/2)−∫_0 ^1 ((ln(1+x))/(x^2 −x+1)) =((ln^2 (2))/2)−∫_0 ^1 (((2x−1)ln(1+x))/((x−j)(x−j^− )))dx ((2x−1)/((x−j)(x−j^− )))=((2j+1)/(j−j^− )).(1/(x−j))+((2j^− −1)/(j^− −j)).(1/(x−j^− )) =∫_0 ^1 ((ln(1+x))/(x+a))dx x+a=u =∫_a ^(a+1) ((ln(1−a+u)))/u)du u=(a−1)t,∀a#1 =∫_(−(a/(1−a))) ^(−((a+1)/(1−a))) ((ln(1−a)+ln(1−t))/t)dt =ln(1−a)(ln(((a+1)/(a−1)))−ln((a/(a−1)))),∀a∈C−(1,−1,0) −∫_(1+(2/(a−1))) ^(1+(1/(a−1))) ((ln(1−t))/t)dt=Li_2 (1+(1/(a−1)))−Li_2 (1+(2/(a−1)))′∀((1/(a−1))&(2/(a−1)))∈C−[1,+∞[ replac a by j and j^− we get close form
$$={ln}\left({x}^{\mathrm{3}} +\mathrm{1}\right)={ln}\left(\left({x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)\right) \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}{ln}^{\mathrm{2}} \left(\mathrm{2}\right)+\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left({x}^{\mathrm{2}} −{x}+\mathrm{1}\right)}{{x}+\mathrm{1}}{dx} \\ $$$$=\frac{{ln}^{\mathrm{2}} \left(\mathrm{2}\right)}{\mathrm{2}}−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left(\mathrm{1}+{x}\right)}{{x}^{\mathrm{2}} −{x}+\mathrm{1}} \\ $$$$=\frac{{ln}^{\mathrm{2}} \left(\mathrm{2}\right)}{\mathrm{2}}−\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\left(\mathrm{2}{x}−\mathrm{1}\right){ln}\left(\mathrm{1}+{x}\right)}{\left({x}−{j}\right)\left({x}−\overset{−} {{j}}\right)}{dx} \\ $$$$\frac{\mathrm{2}{x}−\mathrm{1}}{\left({x}−\boldsymbol{{j}}\right)\left(\boldsymbol{{x}}−\overset{−} {\boldsymbol{{j}}}\right)}=\frac{\mathrm{2}\boldsymbol{{j}}+\mathrm{1}}{{j}−\overset{−} {{j}}}.\frac{\mathrm{1}}{\boldsymbol{{x}}−\boldsymbol{{j}}}+\frac{\mathrm{2}\overset{−} {\boldsymbol{{j}}}−\mathrm{1}}{\overset{−} {{j}}−{j}}.\frac{\mathrm{1}}{\boldsymbol{{x}}−\overset{−} {\boldsymbol{{j}}}} \\ $$$$=\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{ln}\left(\mathrm{1}+{x}\right)}{{x}+{a}}{dx} \\ $$$${x}+{a}={u} \\ $$$$=\int_{{a}} ^{{a}+\mathrm{1}} \frac{\left.{ln}\left(\mathrm{1}−{a}+{u}\right)\right)}{{u}}{du} \\ $$$${u}=\left({a}−\mathrm{1}\right){t},\forall{a}#\mathrm{1} \\ $$$$=\int_{−\frac{{a}}{\mathrm{1}−{a}}} ^{−\frac{{a}+\mathrm{1}}{\mathrm{1}−{a}}} \frac{{ln}\left(\mathrm{1}−{a}\right)+{ln}\left(\mathrm{1}−{t}\right)}{{t}}{dt} \\ $$$$={ln}\left(\mathrm{1}−{a}\right)\left({ln}\left(\frac{{a}+\mathrm{1}}{{a}−\mathrm{1}}\right)−{ln}\left(\frac{{a}}{{a}−\mathrm{1}}\right)\right),\forall{a}\in{C}−\left(\mathrm{1},−\mathrm{1},\mathrm{0}\right) \\ $$$$−\int_{\mathrm{1}+\frac{\mathrm{2}}{{a}−\mathrm{1}}} ^{\mathrm{1}+\frac{\mathrm{1}}{{a}−\mathrm{1}}} \frac{{ln}\left(\mathrm{1}−{t}\right)}{{t}}{dt}={Li}_{\mathrm{2}} \left(\mathrm{1}+\frac{\mathrm{1}}{{a}−\mathrm{1}}\right)−{Li}_{\mathrm{2}} \left(\mathrm{1}+\frac{\mathrm{2}}{{a}−\mathrm{1}}\right)'\forall\left(\frac{\mathrm{1}}{{a}−\mathrm{1}}\&\frac{\mathrm{2}}{{a}−\mathrm{1}}\right)\in{C}−\left[\mathrm{1},+\infty\left[\right.\right. \\ $$$${replac}\:{a}\:{by}\:{j}\:{and}\:\overset{−} {{j}}\:{we}\:{get}\:{close}\:{form} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by puissant last updated on 05/Sep/21
Sir mindispower, you are a boss !!
$${Sir}\:{mindispower},\:{you}\:{are}\:{a}\:{boss}\:!! \\ $$
Commented by mindispower last updated on 07/Sep/21
withe pleasur
$${withe}\:{pleasur} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *