Menu Close

1-sin-x-2cos-x-3-dx-

Tinku Tara 0 Comments
Pin




Question Number 87854 by jagoll last updated on 06/Apr/20
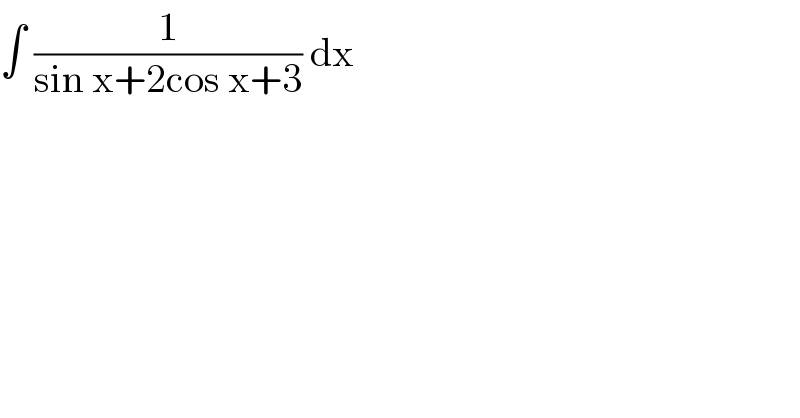
∫ (1/(sin x+2cos x+3)) dx
$$\int\:\frac{\mathrm{1}}{\mathrm{sin}\:\mathrm{x}+\mathrm{2cos}\:\mathrm{x}+\mathrm{3}}\:\mathrm{dx} \\ $$
Commented by mathmax by abdo last updated on 06/Apr/20
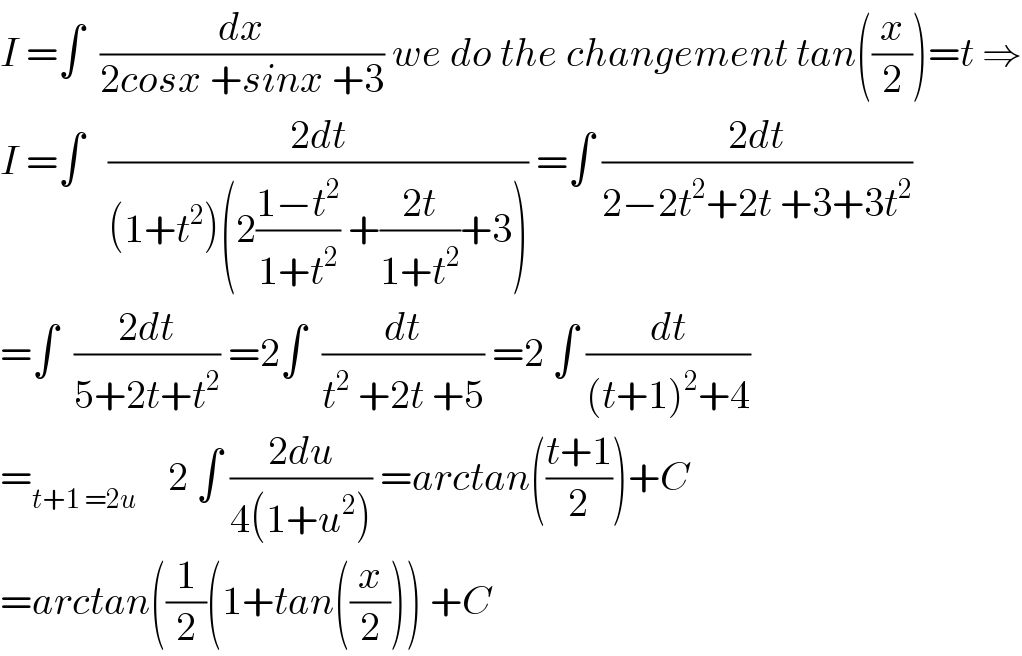
I =∫ (dx/(2cosx +sinx +3)) we do the changement tan((x/2))=t ⇒ I =∫ ((2dt)/((1+t^2 )(2((1−t^2 )/(1+t^2 )) +((2t)/(1+t^2 ))+3))) =∫ ((2dt)/(2−2t^2 +2t +3+3t^2 )) =∫ ((2dt)/(5+2t+t^2 )) =2∫ (dt/(t^2 +2t +5)) =2 ∫ (dt/((t+1)^2 +4)) =_(t+1 =2u) 2 ∫ ((2du)/(4(1+u^2 ))) =arctan(((t+1)/2))+C =arctan((1/2)(1+tan((x/2))) +C
$${I}\:=\int\:\:\frac{{dx}}{\mathrm{2}{cosx}\:+{sinx}\:+\mathrm{3}}\:{we}\:{do}\:{the}\:{changement}\:{tan}\left(\frac{{x}}{\mathrm{2}}\right)={t}\:\Rightarrow \\ $$$${I}\:=\int\:\:\:\frac{\mathrm{2}{dt}}{\left(\mathrm{1}+{t}^{\mathrm{2}} \right)\left(\mathrm{2}\frac{\mathrm{1}−{t}^{\mathrm{2}} }{\mathrm{1}+{t}^{\mathrm{2}} }\:+\frac{\mathrm{2}{t}}{\mathrm{1}+{t}^{\mathrm{2}} }+\mathrm{3}\right)}\:=\int\:\frac{\mathrm{2}{dt}}{\mathrm{2}−\mathrm{2}{t}^{\mathrm{2}} +\mathrm{2}{t}\:+\mathrm{3}+\mathrm{3}{t}^{\mathrm{2}} } \\ $$$$=\int\:\:\frac{\mathrm{2}{dt}}{\mathrm{5}+\mathrm{2}{t}+{t}^{\mathrm{2}} }\:=\mathrm{2}\int\:\:\frac{{dt}}{{t}^{\mathrm{2}} \:+\mathrm{2}{t}\:+\mathrm{5}}\:=\mathrm{2}\:\int\:\frac{{dt}}{\left({t}+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}} \\ $$$$=_{{t}+\mathrm{1}\:=\mathrm{2}{u}} \:\:\:\:\mathrm{2}\:\int\:\frac{\mathrm{2}{du}}{\mathrm{4}\left(\mathrm{1}+{u}^{\mathrm{2}} \right)}\:={arctan}\left(\frac{{t}+\mathrm{1}}{\mathrm{2}}\right)+{C} \\ $$$$={arctan}\left(\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{1}+{tan}\left(\frac{{x}}{\mathrm{2}}\right)\right)\:+{C}\right. \\ $$
Commented by john santu last updated on 07/Apr/20
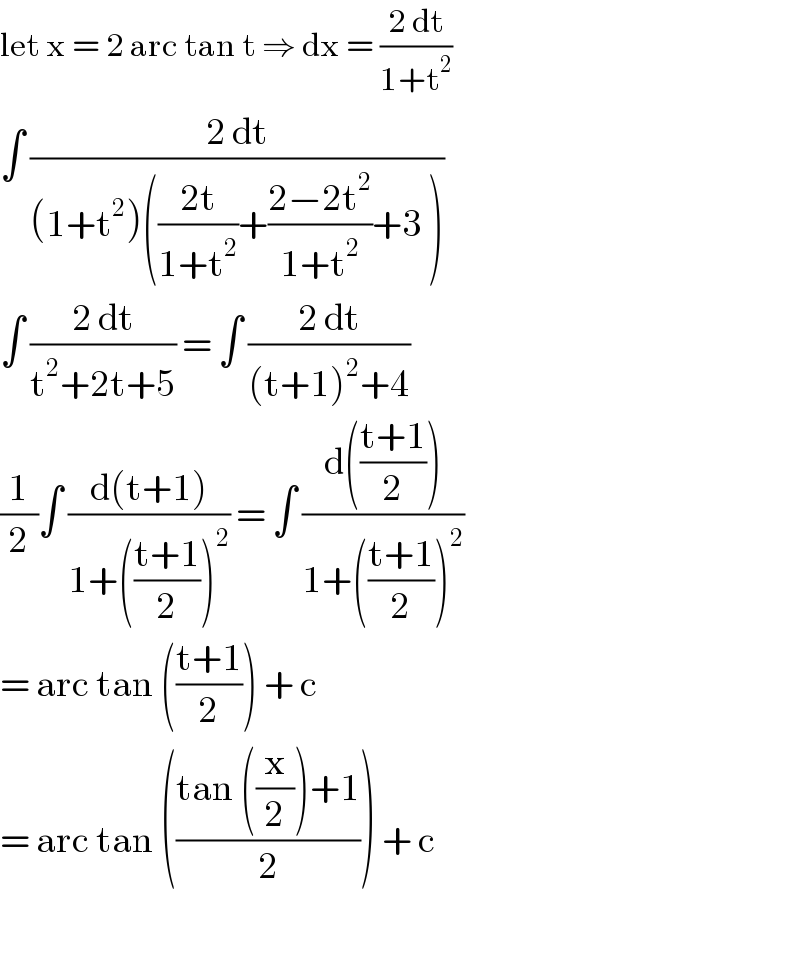
let x = 2 arc tan t ⇒ dx = ((2 dt)/(1+t^2 )) ∫ ((2 dt)/((1+t^2 )(((2t)/(1+t^2 ))+((2−2t^2 )/(1+t^2 ))+3 ))) ∫ ((2 dt)/(t^2 +2t+5)) = ∫ ((2 dt)/((t+1)^2 +4)) (1/2)∫ ((d(t+1))/(1+(((t+1)/2))^2 )) = ∫ ((d(((t+1)/2)))/(1+(((t+1)/2))^2 )) = arc tan (((t+1)/2)) + c = arc tan (((tan ((x/2))+1)/2)) + c
$$\mathrm{let}\:\mathrm{x}\:=\:\mathrm{2}\:\mathrm{arc}\:\mathrm{tan}\:\mathrm{t}\:\Rightarrow\:\mathrm{dx}\:=\:\frac{\mathrm{2}\:\mathrm{dt}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} } \\ $$$$\int\:\frac{\mathrm{2}\:\mathrm{dt}}{\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)\left(\frac{\mathrm{2t}}{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }+\frac{\mathrm{2}−\mathrm{2t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }+\mathrm{3}\:\right)} \\ $$$$\int\:\frac{\mathrm{2}\:\mathrm{dt}}{\mathrm{t}^{\mathrm{2}} +\mathrm{2t}+\mathrm{5}}\:=\:\int\:\frac{\mathrm{2}\:\mathrm{dt}}{\left(\mathrm{t}+\mathrm{1}\right)^{\mathrm{2}} +\mathrm{4}} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}\int\:\frac{\mathrm{d}\left(\mathrm{t}+\mathrm{1}\right)}{\mathrm{1}+\left(\frac{\mathrm{t}+\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} }\:=\:\int\:\frac{\mathrm{d}\left(\frac{\mathrm{t}+\mathrm{1}}{\mathrm{2}}\right)}{\mathrm{1}+\left(\frac{\mathrm{t}+\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} } \\ $$$$=\:\mathrm{arc}\:\mathrm{tan}\:\left(\frac{\mathrm{t}+\mathrm{1}}{\mathrm{2}}\right)\:+\:\mathrm{c} \\ $$$$=\:\mathrm{arc}\:\mathrm{tan}\:\left(\frac{\mathrm{tan}\:\left(\frac{\mathrm{x}}{\mathrm{2}}\right)+\mathrm{1}}{\mathrm{2}}\right)\:+\:\mathrm{c} \\ $$$$ \\ $$
Commented by jagoll last updated on 06/Apr/20
waw...thanks all
$$\mathrm{waw}…\mathrm{thanks}\:\mathrm{all} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *