Question Number 88592 by Rio Michael last updated on 11/Apr/20
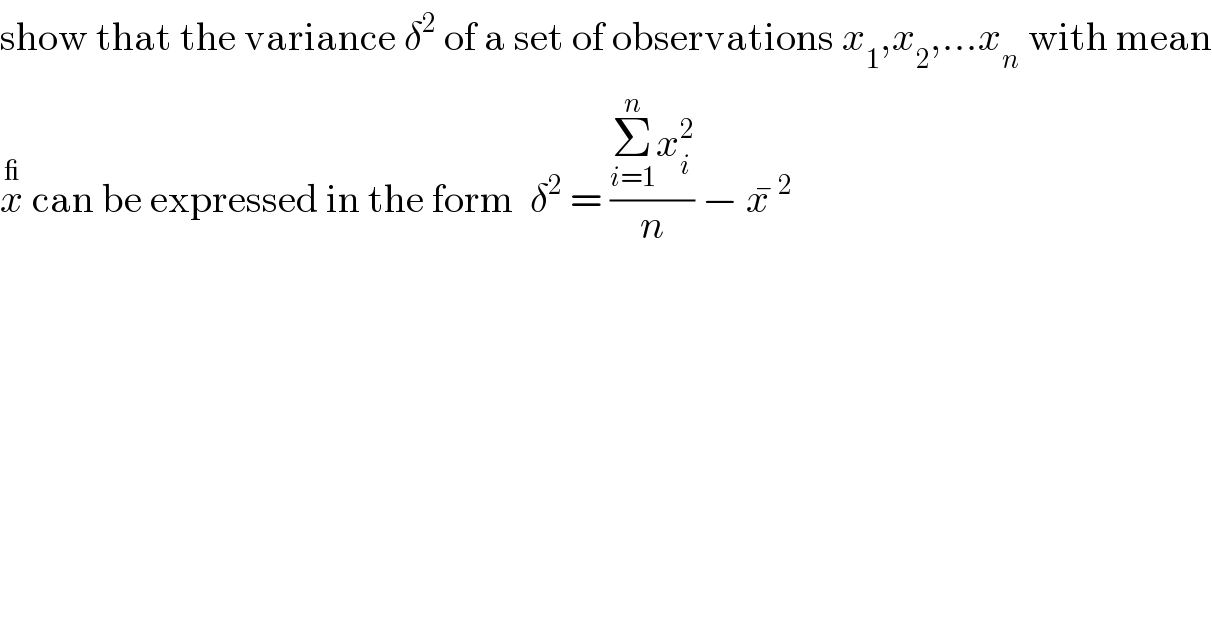
$$\mathrm{show}\:\mathrm{that}\:\mathrm{the}\:\mathrm{variance}\:\delta^{\mathrm{2}} \:\mathrm{of}\:\mathrm{a}\:\mathrm{set}\:\mathrm{of}\:\mathrm{observations}\:{x}_{\mathrm{1}} ,{x}_{\mathrm{2}} ,…{x}_{{n}} \:\mathrm{with}\:\mathrm{mean} \\ $$$$\overset{\_} {{x}}\:\mathrm{can}\:\mathrm{be}\:\mathrm{expressed}\:\mathrm{in}\:\mathrm{the}\:\mathrm{form}\:\:\delta^{\mathrm{2}} \:=\:\frac{\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{x}_{{i}} ^{\mathrm{2}} }{{n}}\:−\:\bar {{x}}\:^{\mathrm{2}\:} \\ $$
Answered by mr W last updated on 11/Apr/20
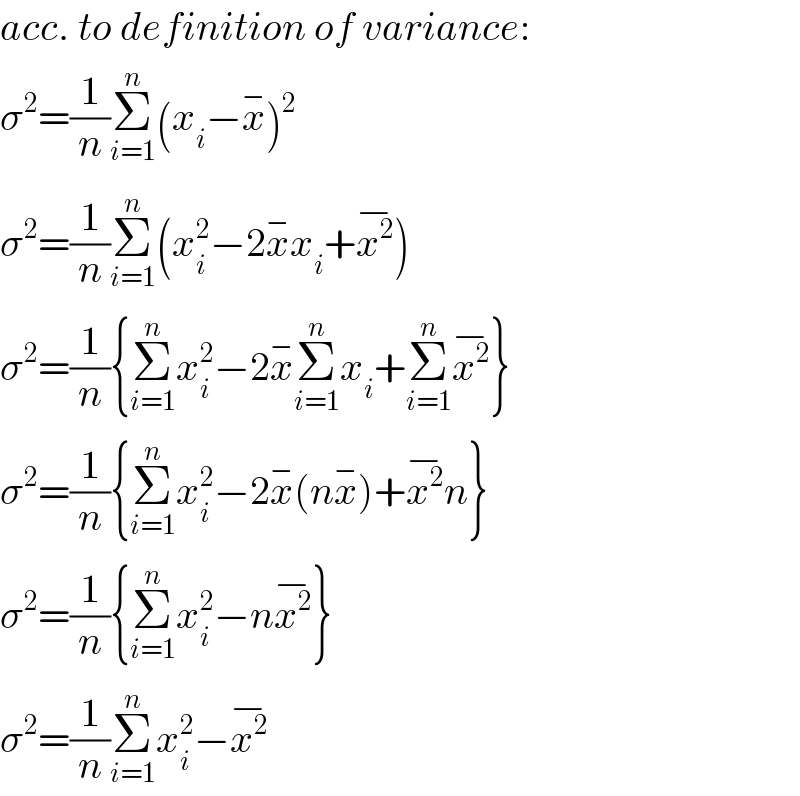
$${acc}.\:{to}\:{definition}\:{of}\:{variance}: \\ $$$$\sigma^{\mathrm{2}} =\frac{\mathrm{1}}{{n}}\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}\left({x}_{{i}} −\overline {{x}}\right)^{\mathrm{2}} \\ $$$$\sigma^{\mathrm{2}} =\frac{\mathrm{1}}{{n}}\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}\left({x}_{{i}} ^{\mathrm{2}} −\mathrm{2}\overline {{x}x}_{{i}} +\overline {{x}^{\mathrm{2}} }\right) \\ $$$$\sigma^{\mathrm{2}} =\frac{\mathrm{1}}{{n}}\left\{\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{x}_{{i}} ^{\mathrm{2}} −\mathrm{2}\overline {{x}}\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{x}_{{i}} +\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}\overline {{x}^{\mathrm{2}} }\right\} \\ $$$$\sigma^{\mathrm{2}} =\frac{\mathrm{1}}{{n}}\left\{\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{x}_{{i}} ^{\mathrm{2}} −\mathrm{2}\overline {{x}}\left({n}\overline {{x}}\right)+\overline {{x}^{\mathrm{2}} }{n}\right\} \\ $$$$\sigma^{\mathrm{2}} =\frac{\mathrm{1}}{{n}}\left\{\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{x}_{{i}} ^{\mathrm{2}} −{n}\overline {{x}^{\mathrm{2}} }\right\} \\ $$$$\sigma^{\mathrm{2}} =\frac{\mathrm{1}}{{n}}\underset{{i}=\mathrm{1}} {\overset{{n}} {\sum}}{x}_{{i}} ^{\mathrm{2}} −\overline {{x}^{\mathrm{2}} } \\ $$
Commented by Rio Michael last updated on 11/Apr/20

$$\mathrm{thanks}\:\mathrm{sir} \\ $$
