Simplify-35-18i-3-1-3-35-18i-3-1-3-4-3-

Question Number 88899 by Ar Brandon last updated on 13/Apr/20

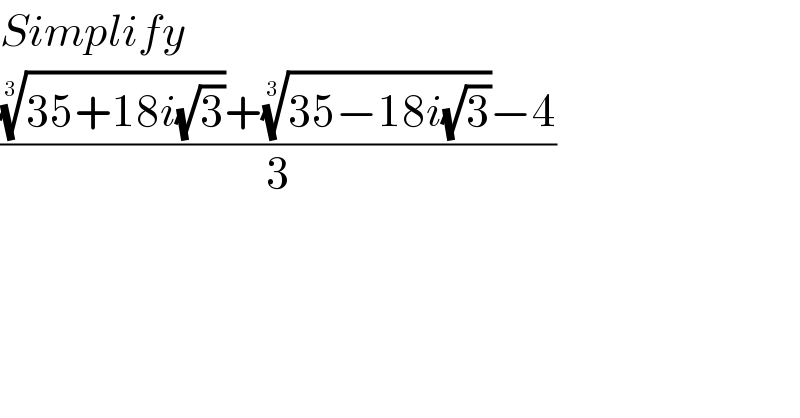
$${Simplify} \\ $$$$\frac{\sqrt[{\mathrm{3}}]{\mathrm{35}+\mathrm{18}{i}\sqrt{\mathrm{3}}}+\sqrt[{\mathrm{3}}]{\mathrm{35}−\mathrm{18}{i}\sqrt{\mathrm{3}}}−\mathrm{4}}{\mathrm{3}} \\ $$

Commented by naka3546 last updated on 14/Apr/20

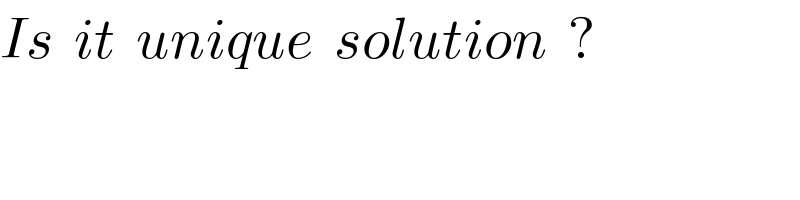
$${Is}\:\:{it}\:\:{unique}\:\:{solution}\:\:? \\ $$

Commented by MJS last updated on 14/Apr/20

usually you get something like this when solving a polynome of 3^(rd) degree using Cardano although D=(p^3 /(27))+(q^2 /4)<0. In these cases Cardano doesn′t work −(q/2)±(√((p^3 /(27))+(q^2 /4)))=35±(√(−972)) ⇒ p=−39∧q=−70 x^3 −39x−70=0 trying factors of 70 ⇒ ⇒ x_1 =−5∧x_2 =−2∧x_3 =7 knowing that the angle of 35+18(√3)i is within [0; (π/2)] the sum of the roots must be >0 ⇒ ((35+18(√3)i))^(1/3) +((35−18(√3)i))^(1/3) =7 ⇒ answer is 1

$$\mathrm{usually}\:\mathrm{you}\:\mathrm{get}\:\mathrm{something}\:\mathrm{like}\:\mathrm{this}\:\mathrm{when} \\ $$$$\mathrm{solving}\:\mathrm{a}\:\mathrm{polynome}\:\mathrm{of}\:\mathrm{3}^{\mathrm{rd}} \:\mathrm{degree}\:\mathrm{using} \\ $$$$\mathrm{Cardano}\:\mathrm{although}\:{D}=\frac{{p}^{\mathrm{3}} }{\mathrm{27}}+\frac{{q}^{\mathrm{2}} }{\mathrm{4}}<\mathrm{0}.\:\mathrm{In}\:\mathrm{these} \\ $$$$\mathrm{cases}\:\mathrm{Cardano}\:\mathrm{doesn}'\mathrm{t}\:\mathrm{work} \\ $$$$−\frac{{q}}{\mathrm{2}}\pm\sqrt{\frac{{p}^{\mathrm{3}} }{\mathrm{27}}+\frac{{q}^{\mathrm{2}} }{\mathrm{4}}}=\mathrm{35}\pm\sqrt{−\mathrm{972}} \\ $$$$\Rightarrow\:{p}=−\mathrm{39}\wedge{q}=−\mathrm{70} \\ $$$${x}^{\mathrm{3}} −\mathrm{39}{x}−\mathrm{70}=\mathrm{0} \\ $$$$\mathrm{trying}\:\mathrm{factors}\:\mathrm{of}\:\mathrm{70}\:\Rightarrow \\ $$$$\Rightarrow\:{x}_{\mathrm{1}} =−\mathrm{5}\wedge{x}_{\mathrm{2}} =−\mathrm{2}\wedge{x}_{\mathrm{3}} =\mathrm{7} \\ $$$$\mathrm{knowing}\:\mathrm{that}\:\mathrm{the}\:\mathrm{angle}\:\mathrm{of}\:\mathrm{35}+\mathrm{18}\sqrt{\mathrm{3}}\mathrm{i}\:\mathrm{is}\:\mathrm{within} \\ $$$$\left[\mathrm{0};\:\frac{\pi}{\mathrm{2}}\right]\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{must}\:\mathrm{be}\:>\mathrm{0} \\ $$$$\Rightarrow\:\sqrt[{\mathrm{3}}]{\mathrm{35}+\mathrm{18}\sqrt{\mathrm{3}}\mathrm{i}}+\sqrt[{\mathrm{3}}]{\mathrm{35}−\mathrm{18}\sqrt{\mathrm{3}}\mathrm{i}}=\mathrm{7} \\ $$$$\Rightarrow\:\mathrm{answer}\:\mathrm{is}\:\mathrm{1} \\ $$

Commented by Ar Brandon last updated on 14/Apr/20

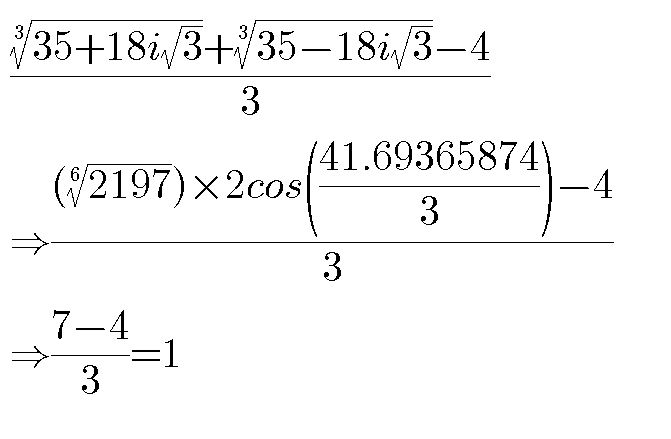
Commented by Ar Brandon last updated on 14/Apr/20

$${I}\:{think}\:{it}\:{goes}\:{in}\:{all}\:{cases}.\:{Just}\:{need}\:{to} \\ $$$${manipulate}\:{the}\:{complex}\:{numbers}. \\ $$

Commented by MJS last updated on 14/Apr/20

but it is not recommended x^3 +ax^2 +bx+c=0 first, try factors of c second, let x=z−(a/3) ⇒ x^3 +px+q=0 D=(p^3 /(27))+(q^2 /4) ⇒ { ((Cardano; D>0)),((trigonometric solution; D<0)) :} try this one using Cardano, exact solutions required: x^3 −8x^2 −154x+332=0

$$\mathrm{but}\:\mathrm{it}\:\mathrm{is}\:\mathrm{not}\:\mathrm{recommended} \\ $$$${x}^{\mathrm{3}} +{ax}^{\mathrm{2}} +{bx}+{c}=\mathrm{0} \\ $$$$\mathrm{first},\:\mathrm{try}\:\mathrm{factors}\:\mathrm{of}\:{c} \\ $$$$\mathrm{second},\:\mathrm{let}\:{x}={z}−\frac{{a}}{\mathrm{3}} \\ $$$$\Rightarrow\:{x}^{\mathrm{3}} +{px}+{q}=\mathrm{0} \\ $$$${D}=\frac{{p}^{\mathrm{3}} }{\mathrm{27}}+\frac{{q}^{\mathrm{2}} }{\mathrm{4}} \\ $$$$\Rightarrow\:\begin{cases}{\mathrm{Cardano};\:{D}>\mathrm{0}}\\{\mathrm{trigonometric}\:\mathrm{solution};\:{D}<\mathrm{0}}\end{cases} \\ $$$$\mathrm{try}\:\mathrm{this}\:\mathrm{one}\:\mathrm{using}\:\mathrm{Cardano},\:\mathrm{exact}\:\mathrm{solutions} \\ $$$$\mathrm{required}: \\ $$$${x}^{\mathrm{3}} −\mathrm{8}{x}^{\mathrm{2}} −\mathrm{154}{x}+\mathrm{332}=\mathrm{0} \\ $$

Commented by Ar Brandon last updated on 02/May/20

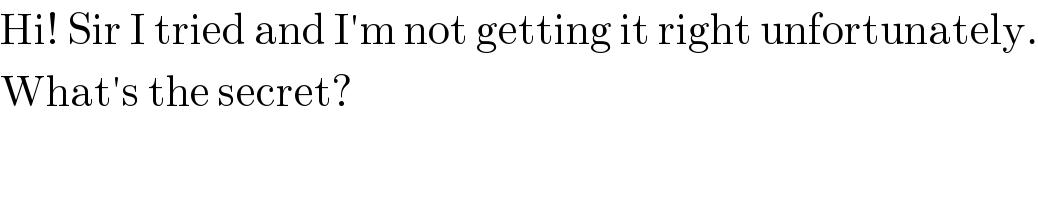
Hi! Sir I tried and I′m not getting it right unfortunately. What′s the secret?

$$\mathrm{Hi}!\:\mathrm{Sir}\:\mathrm{I}\:\mathrm{tried}\:\mathrm{and}\:\mathrm{I}'\mathrm{m}\:\mathrm{not}\:\mathrm{getting}\:\mathrm{it}\:\mathrm{right}\:\mathrm{unfortunately}. \\ $$$$\mathrm{What}'\mathrm{s}\:\mathrm{the}\:\mathrm{secret}? \\ $$

Commented by Ar Brandon last updated on 02/May/20

What PDF document would you recommend me to study in other to master the subject ? ��

Commented by MJS last updated on 02/May/20

I will after the weekend... if I forget, please remind me

$$\mathrm{I}\:\mathrm{will}\:\mathrm{after}\:\mathrm{the}\:\mathrm{weekend}…\:\mathrm{if}\:\mathrm{I}\:\mathrm{forget},\:\mathrm{please} \\ $$$$\mathrm{remind}\:\mathrm{me} \\ $$

Commented by Ar Brandon last updated on 02/May/20

$$\mathrm{OK}\:\mathrm{thanks}\: \\ $$

Commented by Ar Brandon last updated on 05/May/20

Hi Sir ; just to remind you as you said. ��

Commented by MJS last updated on 05/May/20

I only found one pdf in German but there′s an article on Wikipedia, search for “Wikipedia cubic equation”

$$\mathrm{I}\:\mathrm{only}\:\mathrm{found}\:\mathrm{one}\:\mathrm{pdf}\:\mathrm{in}\:\mathrm{German} \\ $$$$\mathrm{but}\:\mathrm{there}'\mathrm{s}\:\mathrm{an}\:\mathrm{article}\:\mathrm{on}\:\mathrm{Wikipedia},\:\mathrm{search} \\ $$$$\mathrm{for}\:“\mathrm{Wikipedia}\:\mathrm{cubic}\:\mathrm{equation}'' \\ $$

Commented by Ar Brandon last updated on 05/May/20

OK, there's no problem. What German PDF did you find ? ��

Commented by MJS last updated on 05/May/20

http://mathemator.org/Media/Themen/Kubische%20Gleichungen.pdf

Commented by Ar Brandon last updated on 05/May/20

thank you Sir ��

Facebook Tweet Pin

Simplify-35-18i-3-1-3-35-18i-3-1-3-4-3-

Leave a Reply Cancel reply