1-x-4-x-3-x-2-1-dx-

Question Number 154780 by talminator2856791 last updated on 21/Sep/21

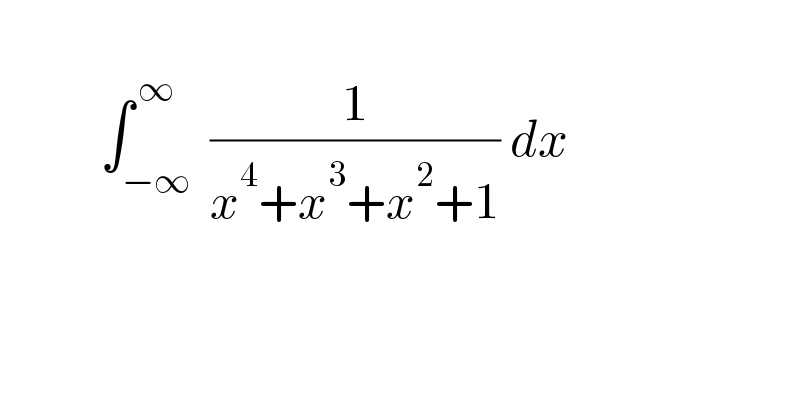
$$\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\int_{−\infty} ^{\:\infty} \:\frac{\mathrm{1}}{{x}^{\mathrm{4}} +{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +\mathrm{1}}\:{dx} \\ $$$$\: \\ $$

Answered by phanphuoc last updated on 21/Sep/21

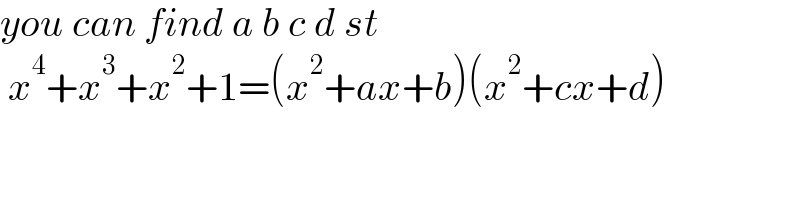
you can find a b c d st x^4 +x^3 +x^2 +1=(x^2 +ax+b)(x^2 +cx+d)

$${you}\:{can}\:{find}\:{a}\:{b}\:{c}\:{d}\:{st} \\ $$$$\:{x}^{\mathrm{4}} +{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +\mathrm{1}=\left({x}^{\mathrm{2}} +{ax}+{b}\right)\left({x}^{\mathrm{2}} +{cx}+{d}\right) \\ $$

Answered by MJS_new last updated on 21/Sep/21

(x−1)(x^4 +x^3 +x^2 +x+1)=x^5 −1 ⇒ x^4 +x^3 +x^2 +x+1=(x^2 +((1−(√5))/2)x+1)(x^2 +((1+(√5))/2)x+1) ∫(dx/(x^4 +x^3 +x^2 +x+1))= =−((√5)/(10))∫((2x+1−(√5))/(x^2 +((1−(√5))/2)x+1))dx+((√5)/(10))∫((2x+1+(√5))/(x^2 +((1+(√5))/2)x+1))dx now use formula

$$\left({x}−\mathrm{1}\right)\left({x}^{\mathrm{4}} +{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +{x}+\mathrm{1}\right)={x}^{\mathrm{5}} −\mathrm{1} \\ $$$$\Rightarrow \\ $$$${x}^{\mathrm{4}} +{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +{x}+\mathrm{1}=\left({x}^{\mathrm{2}} +\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right)\left({x}^{\mathrm{2}} +\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}\right) \\ $$$$\int\frac{{dx}}{{x}^{\mathrm{4}} +{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +{x}+\mathrm{1}}= \\ $$$$=−\frac{\sqrt{\mathrm{5}}}{\mathrm{10}}\int\frac{\mathrm{2}{x}+\mathrm{1}−\sqrt{\mathrm{5}}}{{x}^{\mathrm{2}} +\frac{\mathrm{1}−\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}}{dx}+\frac{\sqrt{\mathrm{5}}}{\mathrm{10}}\int\frac{\mathrm{2}{x}+\mathrm{1}+\sqrt{\mathrm{5}}}{{x}^{\mathrm{2}} +\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{2}}{x}+\mathrm{1}}{dx} \\ $$$$\mathrm{now}\:\mathrm{use}\:\mathrm{formula} \\ $$

Commented by Ar Brandon last updated on 21/Sep/21

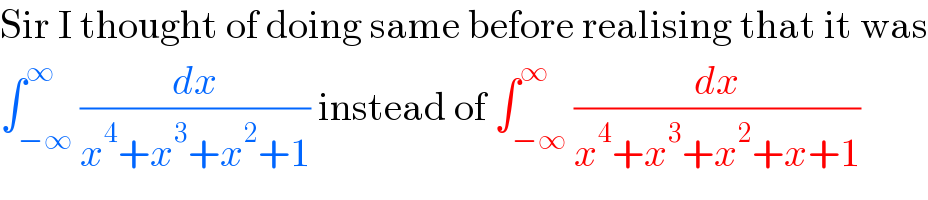
Sir I thought of doing same before realising that it was ∫_(−∞) ^∞ (dx/(x^4 +x^3 +x^2 +1)) instead of ∫_(−∞) ^∞ (dx/(x^4 +x^3 +x^2 +x+1))

$$\mathrm{Sir}\:\mathrm{I}\:\mathrm{thought}\:\mathrm{of}\:\mathrm{doing}\:\mathrm{same}\:\mathrm{before}\:\mathrm{realising}\:\mathrm{that}\:\mathrm{it}\:\mathrm{was} \\ $$$$\int_{−\infty} ^{\infty} \frac{{dx}}{{x}^{\mathrm{4}} +{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +\mathrm{1}}\:\mathrm{instead}\:\mathrm{of}\:\int_{−\infty} ^{\infty} \frac{{dx}}{{x}^{\mathrm{4}} +{x}^{\mathrm{3}} +{x}^{\mathrm{2}} +{x}+\mathrm{1}} \\ $$

Commented by MJS_new last updated on 21/Sep/21

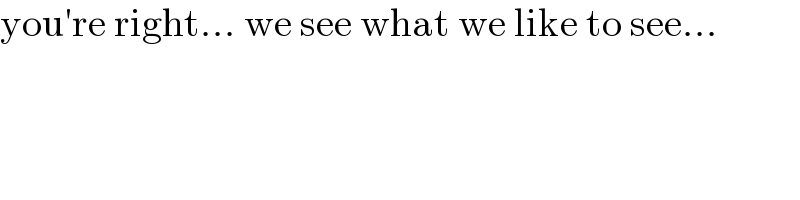
you′re right... we see what we like to see...

$$\mathrm{you}'\mathrm{re}\:\mathrm{right}…\:\mathrm{we}\:\mathrm{see}\:\mathrm{what}\:\mathrm{we}\:\mathrm{like}\:\mathrm{to}\:\mathrm{see}… \\ $$

Commented by Rasheed.Sindhi last updated on 21/Sep/21

Good Saying: we see what we like to see!

$$\mathcal{G}{ood}\:\mathcal{S}{aying}:\:\mathrm{we}\:\mathrm{see}\:\mathrm{what}\:\mathrm{we}\:\mathrm{like}\:\mathrm{to}\:\mathrm{see}! \\ $$

Answered by mindispower last updated on 22/Sep/21

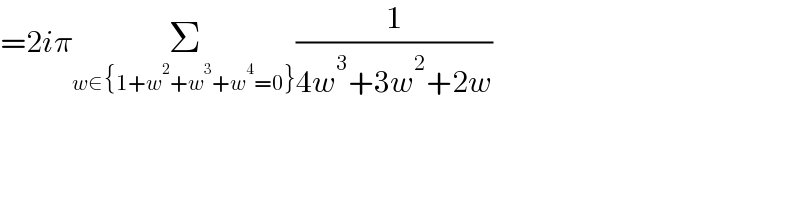
=2iπΣ_(w∈{1+w^2 +w^3 +w^4 =0}) (1/(4w^3 +3w^2 +2w))

$$=\mathrm{2}{i}\pi\underset{{w}\in\left\{\mathrm{1}+{w}^{\mathrm{2}} +{w}^{\mathrm{3}} +{w}^{\mathrm{4}} =\mathrm{0}\right\}} {\sum}\frac{\mathrm{1}}{\mathrm{4}{w}^{\mathrm{3}} +\mathrm{3}{w}^{\mathrm{2}} +\mathrm{2}{w}} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply