Question Number 89314 by abdomathmax last updated on 16/Apr/20
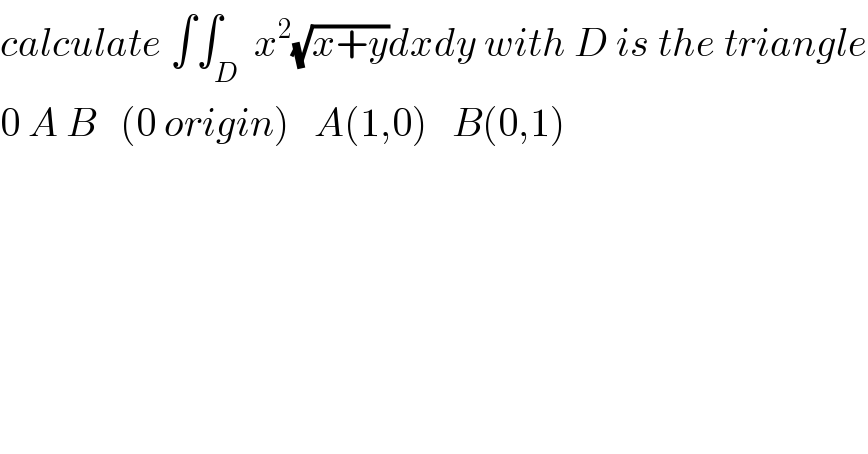
$${calculate}\:\int\int_{{D}} \:{x}^{\mathrm{2}} \sqrt{{x}+{y}}{dxdy}\:{with}\:{D}\:{is}\:{the}\:{triangle} \\ $$$$\mathrm{0}\:{A}\:{B}\:\:\:\left(\mathrm{0}\:{origin}\right)\:\:\:{A}\left(\mathrm{1},\mathrm{0}\right)\:\:\:{B}\left(\mathrm{0},\mathrm{1}\right) \\ $$
Commented by abdomathmax last updated on 18/Apr/20
![the equation of line (AB) is x+y=1 ⇒y=1−x ⇒ ∫∫_D x^2 (√(x+y))dxdy =∫_0 ^1 (∫_0 ^(1−x) (√(x+y))dy)x^2 dx wehave ∫_0 ^(1−x) (√(x+y))dy =_(x+y=t^2 ) ∫_(√x) ^1 t (2t)dt =2 ∫_(√x) ^1 t^2 dt =(2/3)[t^3 ]_(√x) ^1 =(2/3)(1−x(√x)) ⇒ I =(2/3)∫_0 ^1 (1−x(√x))x^2 dx =(2/3) ∫_0 ^1 x^2 dx−(2/3)∫_0 ^1 x^3 (√x)dx =(2/9)−(2/3)∫_0 ^1 x^3 (√x)dx we have ∫_0 ^1 x^3 (√x)dx =_((√x)=u) ∫_0 ^1 u^6 .u(2u)du =2 ∫_0 ^1 u^8 du =(2/9) ⇒ I =(2/9)−(2/3)×(2/9) =(2/9)(1−(2/3))=(2/9)×(1/3)=(2/(27))](https://www.tinkutara.com/question/Q89675.png)
$${the}\:{equation}\:{of}\:{line}\:\left({AB}\right)\:{is}\:{x}+{y}=\mathrm{1}\:\Rightarrow{y}=\mathrm{1}−{x}\:\Rightarrow \\ $$$$\int\int_{{D}} {x}^{\mathrm{2}} \sqrt{{x}+{y}}{dxdy}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \left(\int_{\mathrm{0}} ^{\mathrm{1}−{x}} \sqrt{{x}+{y}}{dy}\right){x}^{\mathrm{2}} \:{dx}\:{wehave} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}−{x}} \sqrt{{x}+{y}}{dy}\:=_{{x}+{y}={t}^{\mathrm{2}} } \:\:\:\:\int_{\sqrt{{x}}} ^{\mathrm{1}} {t}\:\left(\mathrm{2}{t}\right){dt} \\ $$$$=\mathrm{2}\:\int_{\sqrt{{x}}} ^{\mathrm{1}} {t}^{\mathrm{2}} \:{dt}\:=\frac{\mathrm{2}}{\mathrm{3}}\left[{t}^{\mathrm{3}} \right]_{\sqrt{{x}}} ^{\mathrm{1}} \:=\frac{\mathrm{2}}{\mathrm{3}}\left(\mathrm{1}−{x}\sqrt{{x}}\right)\:\Rightarrow \\ $$$${I}\:=\frac{\mathrm{2}}{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{1}} \left(\mathrm{1}−{x}\sqrt{{x}}\right){x}^{\mathrm{2}} {dx} \\ $$$$=\frac{\mathrm{2}}{\mathrm{3}}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:{x}^{\mathrm{2}} \:{dx}−\frac{\mathrm{2}}{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{1}} {x}^{\mathrm{3}} \sqrt{{x}}{dx} \\ $$$$=\frac{\mathrm{2}}{\mathrm{9}}−\frac{\mathrm{2}}{\mathrm{3}}\int_{\mathrm{0}} ^{\mathrm{1}} \:{x}^{\mathrm{3}} \sqrt{{x}}{dx}\:\:{we}\:{have} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:{x}^{\mathrm{3}} \sqrt{{x}}{dx}\:=_{\sqrt{{x}}={u}} \:\:\:\int_{\mathrm{0}} ^{\mathrm{1}} {u}^{\mathrm{6}} .{u}\left(\mathrm{2}{u}\right){du} \\ $$$$=\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{1}} {u}^{\mathrm{8}} \:{du}\:=\frac{\mathrm{2}}{\mathrm{9}}\:\Rightarrow \\ $$$${I}\:=\frac{\mathrm{2}}{\mathrm{9}}−\frac{\mathrm{2}}{\mathrm{3}}×\frac{\mathrm{2}}{\mathrm{9}}\:=\frac{\mathrm{2}}{\mathrm{9}}\left(\mathrm{1}−\frac{\mathrm{2}}{\mathrm{3}}\right)=\frac{\mathrm{2}}{\mathrm{9}}×\frac{\mathrm{1}}{\mathrm{3}}=\frac{\mathrm{2}}{\mathrm{27}} \\ $$
