Question Number 90233 by jagoll last updated on 22/Apr/20

$$\mathrm{3},\mathrm{4},\mathrm{12},\mathrm{39},\mathrm{103},\mathrm{x}\: \\ $$$$\left(\mathrm{a}\right)\:\mathrm{164} \\ $$$$\left(\mathrm{b}\right)\:\mathrm{170} \\ $$$$\left(\mathrm{c}\right)\:\mathrm{172} \\ $$$$\left(\mathrm{d}\right)\:\mathrm{228} \\ $$
Commented by john santu last updated on 22/Apr/20

$$\mathrm{4}\:=\:\mathrm{3}+\mathrm{1}^{\mathrm{3}} \\ $$$$\mathrm{12}=\:\mathrm{4}+\mathrm{2}^{\mathrm{3}} \\ $$$$\mathrm{39}\:=\:\mathrm{12}+\mathrm{3}^{\mathrm{3}} \\ $$$$\mathrm{103}\:=\:\mathrm{39}+\mathrm{4}^{\mathrm{3}} \\ $$$${x}\:=\:\mathrm{103}\:+\mathrm{5}^{\mathrm{3}} \:=\:\mathrm{228} \\ $$
Commented by peter frank last updated on 22/Apr/20

$${thank}\:{you} \\ $$
Commented by MJS last updated on 22/Apr/20
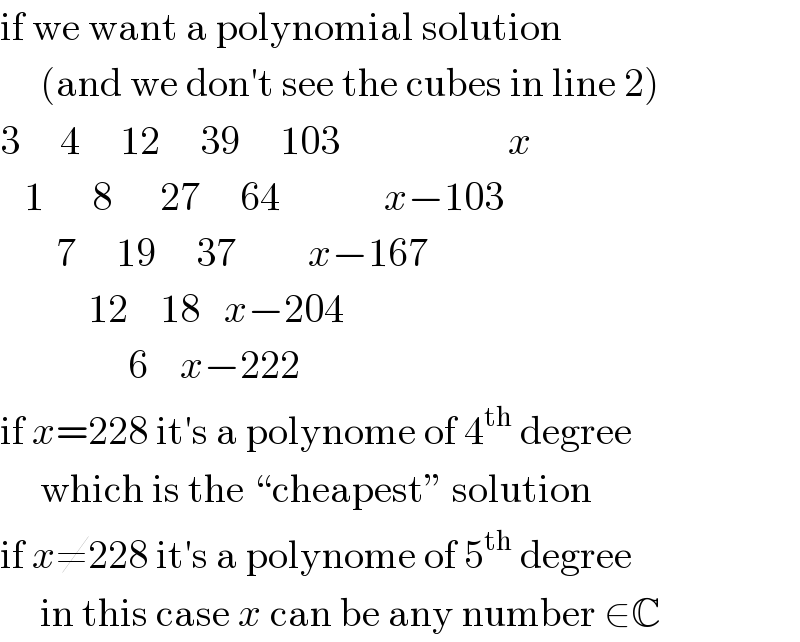
$$\mathrm{if}\:\mathrm{we}\:\mathrm{want}\:\mathrm{a}\:\mathrm{polynomial}\:\mathrm{solution} \\ $$$$\:\:\:\:\:\left(\mathrm{and}\:\mathrm{we}\:\mathrm{don}'\mathrm{t}\:\mathrm{see}\:\mathrm{the}\:\mathrm{cubes}\:\mathrm{in}\:\mathrm{line}\:\mathrm{2}\right) \\ $$$$\mathrm{3}\:\:\:\:\:\mathrm{4}\:\:\:\:\:\mathrm{12}\:\:\:\:\:\mathrm{39}\:\:\:\:\:\mathrm{103}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{x} \\ $$$$\:\:\:\mathrm{1}\:\:\:\:\:\:\mathrm{8}\:\:\:\:\:\:\mathrm{27}\:\:\:\:\:\mathrm{64}\:\:\:\:\:\:\:\:\:\:\:\:\:{x}−\mathrm{103} \\ $$$$\:\:\:\:\:\:\:\mathrm{7}\:\:\:\:\:\mathrm{19}\:\:\:\:\:\mathrm{37}\:\:\:\:\:\:\:\:\:{x}−\mathrm{167} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{12}\:\:\:\:\mathrm{18}\:\:\:{x}−\mathrm{204} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{6}\:\:\:\:{x}−\mathrm{222} \\ $$$$\mathrm{if}\:{x}=\mathrm{228}\:\mathrm{it}'\mathrm{s}\:\mathrm{a}\:\mathrm{polynome}\:\mathrm{of}\:\mathrm{4}^{\mathrm{th}} \:\mathrm{degree} \\ $$$$\:\:\:\:\:\mathrm{which}\:\mathrm{is}\:\mathrm{the}\:“\mathrm{cheapest}''\:\mathrm{solution} \\ $$$$\mathrm{if}\:{x}\neq\mathrm{228}\:\mathrm{it}'\mathrm{s}\:\mathrm{a}\:\mathrm{polynome}\:\mathrm{of}\:\mathrm{5}^{\mathrm{th}} \:\mathrm{degree} \\ $$$$\:\:\:\:\:\mathrm{in}\:\mathrm{this}\:\mathrm{case}\:{x}\:\mathrm{can}\:\mathrm{be}\:\mathrm{any}\:\mathrm{number}\:\in\mathbb{C} \\ $$
Commented by jagoll last updated on 22/Apr/20

$$\mathrm{in}\:\mathrm{line}\:\mathrm{2}\::\:\mathrm{1}^{\mathrm{3}} \:,\:\mathrm{2}^{\mathrm{3}} \:,\:\mathrm{3}^{\mathrm{3}} ,\:\mathrm{4}^{\mathrm{3}} ,\:\mathrm{5}^{\mathrm{3}} \:? \\ $$$$ \\ $$
Commented by MJS last updated on 22/Apr/20

$$\mathrm{yes}.\:{x}−\mathrm{103}=\mathrm{5}^{\mathrm{3}} \\ $$$$\mathrm{that}'\mathrm{s}\:\mathrm{what}\:\mathrm{Sir}\:\mathrm{John}\:\mathrm{Santu}\:\mathrm{found} \\ $$
