Question Number 156795 by tabata last updated on 15/Oct/21
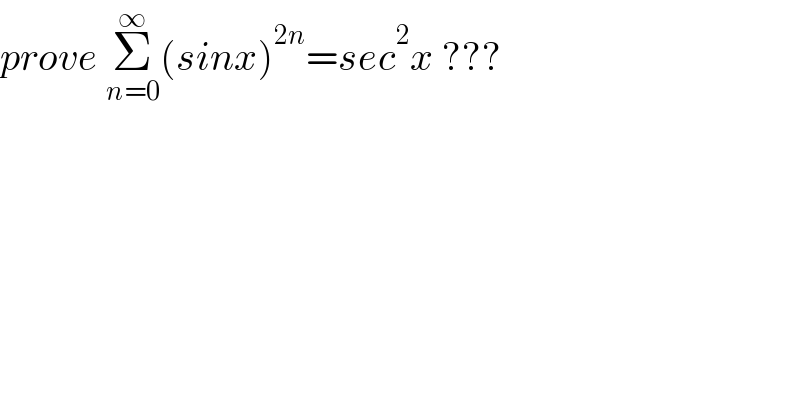
$${prove}\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left({sinx}\right)^{\mathrm{2}{n}} ={sec}^{\mathrm{2}} {x}\:??? \\ $$
Answered by FongXD last updated on 15/Oct/21
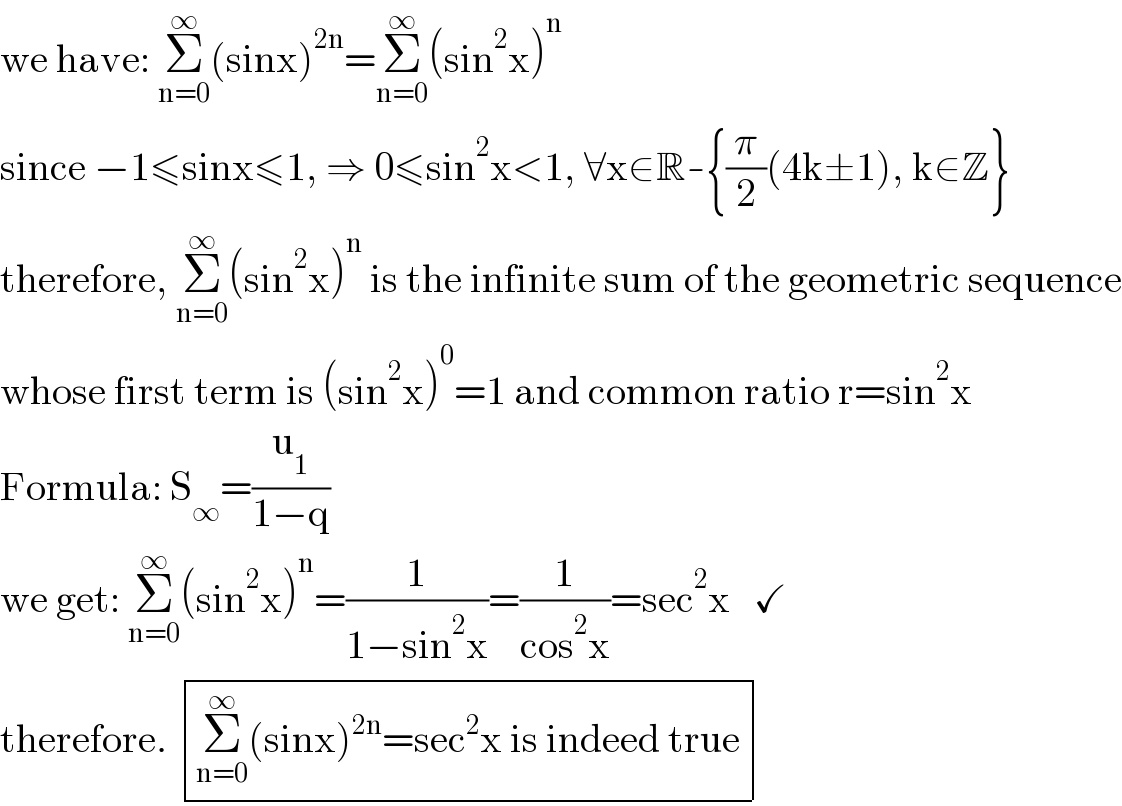
$$\mathrm{we}\:\mathrm{have}:\:\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(\mathrm{sinx}\right)^{\mathrm{2n}} =\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(\mathrm{sin}^{\mathrm{2}} \mathrm{x}\right)^{\mathrm{n}} \\ $$$$\mathrm{since}\:−\mathrm{1}\leqslant\mathrm{sinx}\leqslant\mathrm{1},\:\Rightarrow\:\mathrm{0}\leqslant\mathrm{sin}^{\mathrm{2}} \mathrm{x}<\mathrm{1},\:\forall\mathrm{x}\in\mathbb{R}-\left\{\frac{\pi}{\mathrm{2}}\left(\mathrm{4k}\pm\mathrm{1}\right),\:\mathrm{k}\in\mathbb{Z}\right\} \\ $$$$\mathrm{therefore},\:\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(\mathrm{sin}^{\mathrm{2}} \mathrm{x}\right)^{\mathrm{n}} \:\mathrm{is}\:\mathrm{the}\:\mathrm{infinite}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{geometric}\:\mathrm{sequence} \\ $$$$\mathrm{whose}\:\mathrm{first}\:\mathrm{term}\:\mathrm{is}\:\left(\mathrm{sin}^{\mathrm{2}} \mathrm{x}\right)^{\mathrm{0}} =\mathrm{1}\:\mathrm{and}\:\mathrm{common}\:\mathrm{ratio}\:\mathrm{r}=\mathrm{sin}^{\mathrm{2}} \mathrm{x} \\ $$$$\mathrm{Formula}:\:\mathrm{S}_{\infty} =\frac{\mathrm{u}_{\mathrm{1}} }{\mathrm{1}−\mathrm{q}} \\ $$$$\mathrm{we}\:\mathrm{get}:\:\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(\mathrm{sin}^{\mathrm{2}} \mathrm{x}\right)^{\mathrm{n}} =\frac{\mathrm{1}}{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \mathrm{x}}=\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}=\mathrm{sec}^{\mathrm{2}} \mathrm{x}\:\:\:\checkmark \\ $$$$\mathrm{therefore}.\:\begin{array}{|c|}{\underset{\mathrm{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(\mathrm{sinx}\right)^{\mathrm{2n}} =\mathrm{sec}^{\mathrm{2}} \mathrm{x}\:\mathrm{is}\:\mathrm{indeed}\:\mathrm{true}}\\\hline\end{array} \\ $$
Commented by Ruuudiy last updated on 17/Oct/21

$$\mathrm{Thanks}\:\boldsymbol{\mathrm{Ser}}.\:\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\left(\mathrm{sin}\:{x}\right)^{\mathrm{2}{n}} =\mathrm{sec}^{\mathrm{2}} {x}\:\mathrm{is}\:\mathrm{indeed}\:\mathrm{true}. \\ $$
Answered by som(math1967) last updated on 15/Oct/21
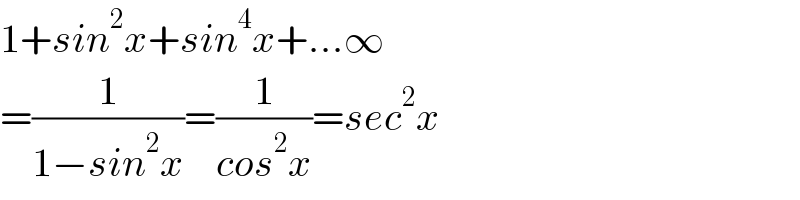
$$\mathrm{1}+{sin}^{\mathrm{2}} {x}+{sin}^{\mathrm{4}} {x}+…\infty \\ $$$$=\frac{\mathrm{1}}{\mathrm{1}−{sin}^{\mathrm{2}} {x}}=\frac{\mathrm{1}}{{cos}^{\mathrm{2}} {x}}={sec}^{\mathrm{2}} {x} \\ $$
