Question Number 157046 by ajfour last updated on 18/Oct/21
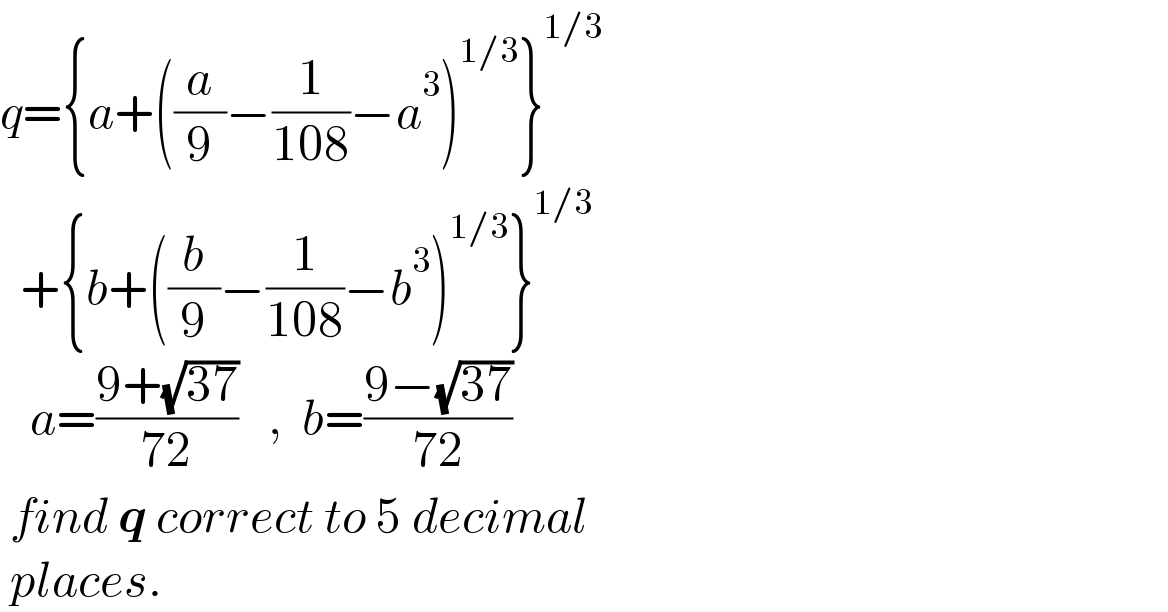
$${q}=\left\{{a}+\left(\frac{{a}}{\mathrm{9}}−\frac{\mathrm{1}}{\mathrm{108}}−{a}^{\mathrm{3}} \right)^{\mathrm{1}/\mathrm{3}} \right\}^{\mathrm{1}/\mathrm{3}} \\ $$$$\:\:+\left\{{b}+\left(\frac{{b}}{\mathrm{9}}−\frac{\mathrm{1}}{\mathrm{108}}−{b}^{\mathrm{3}} \right)^{\mathrm{1}/\mathrm{3}} \right\}^{\mathrm{1}/\mathrm{3}} \\ $$$$\:\:\:{a}=\frac{\mathrm{9}+\sqrt{\mathrm{37}}}{\mathrm{72}}\:\:\:,\:\:{b}=\frac{\mathrm{9}−\sqrt{\mathrm{37}}}{\mathrm{72}} \\ $$$$\:{find}\:\boldsymbol{{q}}\:{correct}\:{to}\:\mathrm{5}\:{decimal} \\ $$$$\:{places}. \\ $$
Commented by ajfour last updated on 18/Oct/21
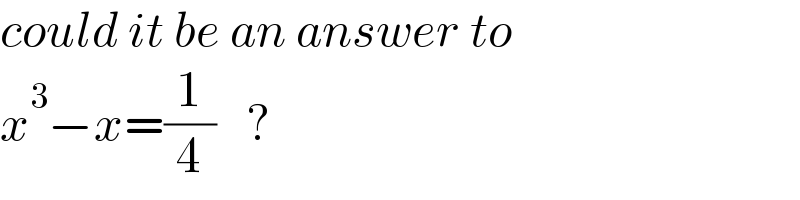
$${could}\:{it}\:{be}\:{an}\:{answer}\:{to} \\ $$$${x}^{\mathrm{3}} −{x}=\frac{\mathrm{1}}{\mathrm{4}}\:\:\:? \\ $$
