Question Number 158543 by ajfour last updated on 06/Nov/21

Commented by ajfour last updated on 06/Nov/21
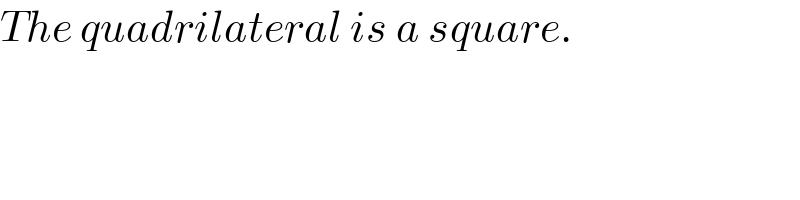
$${The}\:{quadrilateral}\:{is}\:{a}\:{square}. \\ $$
Commented by mr W last updated on 06/Nov/21

Commented by mr W last updated on 06/Nov/21

$$\mathrm{tan}\:\theta={a} \\ $$$$\varphi=\frac{\pi}{\mathrm{4}}+\frac{\theta}{\mathrm{2}} \\ $$$${Radius}\:{big}\:{circle}={a}+\frac{{a}}{\mathrm{sin}\:\varphi}=\frac{{a}}{\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta} \\ $$$$\mathrm{1}+\frac{\mathrm{1}}{\mathrm{sin}\:\left(\frac{\pi}{\mathrm{4}}+\frac{\theta}{\mathrm{2}}\right)}=\frac{\mathrm{1}}{\mathrm{sin}\:\theta\:\mathrm{cos}\:\theta} \\ $$$$\Rightarrow\theta\approx\mathrm{35}.\mathrm{0446}° \\ $$$$\Rightarrow{a}\approx\mathrm{0}.\mathrm{7014} \\ $$
Commented by Tawa11 last updated on 06/Nov/21

$$\mathrm{Weldone}\:\mathrm{sir} \\ $$
Answered by ajfour last updated on 06/Nov/21

$${let}\:\:{a}=\mathrm{tan}\:\theta \\ $$$$\mathrm{1}+{a}^{\mathrm{2}} ={R} \\ $$$$\left({R}−{a}\right)^{\mathrm{2}} ={a}^{\mathrm{2}} +{b}^{\mathrm{2}} \\ $$$${a}^{\mathrm{2}} +{a}^{\mathrm{4}} =\left\{\left({a}^{\mathrm{2}} +{b}\right)\mathrm{sin}\:\theta+{b}\right\}^{\mathrm{2}} \\ $$$$\Rightarrow \\ $$$$\left(\mathrm{1}+{a}^{\mathrm{2}} −{a}\right)^{\mathrm{2}} ={a}^{\mathrm{2}} +{b}^{\mathrm{2}} \\ $$$${a}^{\mathrm{2}} +{a}^{\mathrm{4}} =\left\{\frac{{a}\left({a}^{\mathrm{2}} +{b}\right)+{b}\sqrt{\mathrm{1}+{a}^{\mathrm{2}} }}{\:\sqrt{\mathrm{1}+{a}^{\mathrm{2}} }}\right\}^{\mathrm{2}} \\ $$$${a}\left(\mathrm{1}+{a}^{\mathrm{2}} \right)={a}^{\mathrm{3}} + \\ $$$$\:\:\:\:\:\:\:\left({a}+\sqrt{\mathrm{1}+{a}^{\mathrm{2}} }\right)\left(\mathrm{1}−{a}\right)\sqrt{\mathrm{1}+{a}^{\mathrm{2}} } \\ $$$$\Rightarrow \\ $$$${a}\left(\sqrt{\mathrm{1}+{a}^{\mathrm{2}} }−{a}\right)=\left(\mathrm{1}−{a}\right)\sqrt{\mathrm{1}+{a}^{\mathrm{2}} } \\ $$$$\Rightarrow\:\:\left(\mathrm{2}{a}−\mathrm{1}\right)\sqrt{\mathrm{1}+{a}^{\mathrm{2}} }={a}^{\mathrm{2}} \\ $$$$\Rightarrow\:\:\left(\mathrm{2}−\frac{\mathrm{1}}{{a}}\right)\sqrt{\mathrm{1}+\frac{\mathrm{1}}{{a}^{\mathrm{2}} }}=\mathrm{1} \\ $$$$\Rightarrow\:\:{a}\approx\mathrm{0}.\mathrm{70136}\:\:\left(>\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$
