Question Number 93483 by Rio Michael last updated on 13/May/20
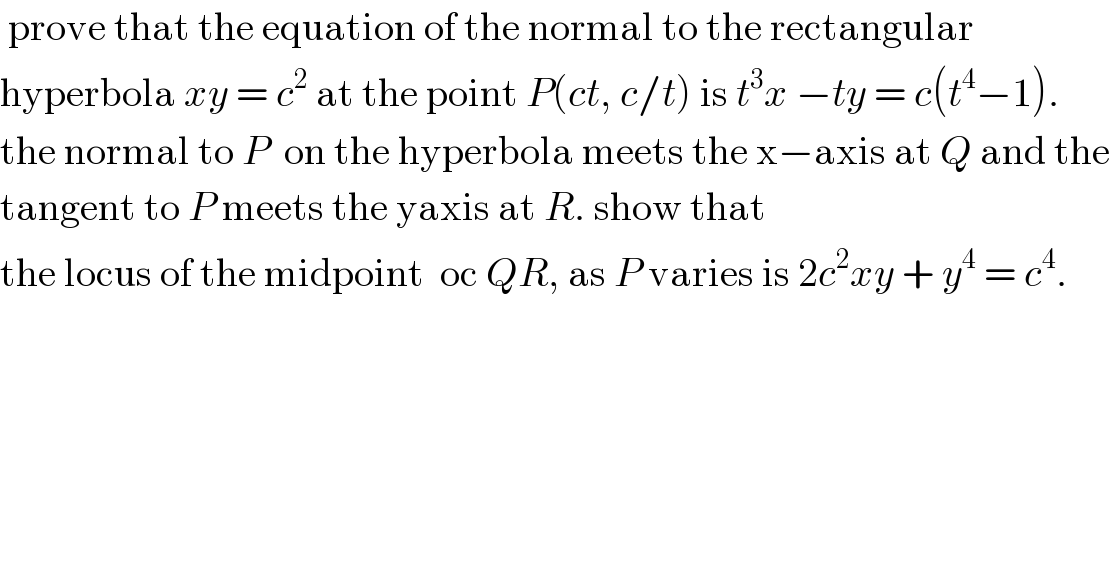
$$\:\mathrm{prove}\:\mathrm{that}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{the}\:\mathrm{normal}\:\mathrm{to}\:\mathrm{the}\:\mathrm{rectangular} \\ $$$$\mathrm{hyperbola}\:{xy}\:=\:{c}^{\mathrm{2}} \:\mathrm{at}\:\mathrm{the}\:\mathrm{point}\:{P}\left({ct},\:{c}/{t}\right)\:\mathrm{is}\:{t}^{\mathrm{3}} {x}\:−{ty}\:=\:{c}\left({t}^{\mathrm{4}} −\mathrm{1}\right). \\ $$$$\mathrm{the}\:\mathrm{normal}\:\mathrm{to}\:{P}\:\:\mathrm{on}\:\mathrm{the}\:\mathrm{hyperbola}\:\mathrm{meets}\:\mathrm{the}\:\mathrm{x}−\mathrm{axis}\:\mathrm{at}\:{Q}\:\mathrm{and}\:\mathrm{the} \\ $$$$\mathrm{tangent}\:\mathrm{to}\:{P}\:\mathrm{meets}\:\mathrm{the}\:\mathrm{yaxis}\:\mathrm{at}\:{R}.\:\mathrm{show}\:\mathrm{that} \\ $$$$\mathrm{the}\:\mathrm{locus}\:\mathrm{of}\:\mathrm{the}\:\mathrm{midpoint}\:\:\mathrm{oc}\:{QR},\:\mathrm{as}\:{P}\:\mathrm{varies}\:\mathrm{is}\:\mathrm{2}{c}^{\mathrm{2}} {xy}\:+\:{y}^{\mathrm{4}} \:=\:{c}^{\mathrm{4}} . \\ $$
