Question Number 159125 by mr W last updated on 13/Nov/21
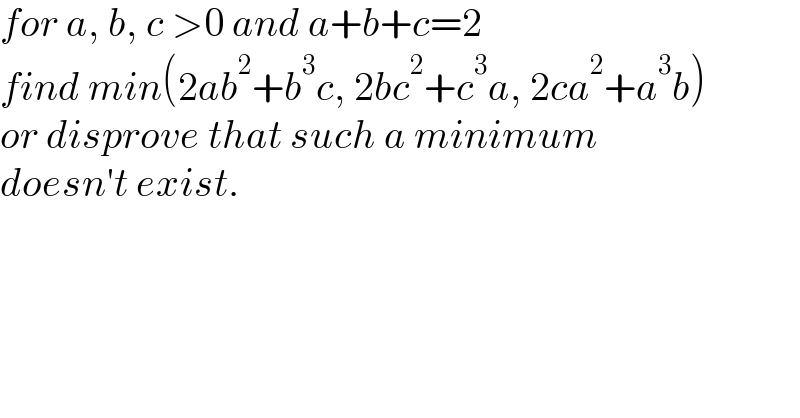
$${for}\:{a},\:{b},\:{c}\:>\mathrm{0}\:{and}\:{a}+{b}+{c}=\mathrm{2} \\ $$$${find}\:{min}\left(\mathrm{2}{ab}^{\mathrm{2}} +{b}^{\mathrm{3}} {c},\:\mathrm{2}{bc}^{\mathrm{2}} +{c}^{\mathrm{3}} {a},\:\mathrm{2}{ca}^{\mathrm{2}} +{a}^{\mathrm{3}} {b}\right) \\ $$$${or}\:{disprove}\:{that}\:{such}\:{a}\:{minimum} \\ $$$${doesn}'{t}\:{exist}. \\ $$
Commented by mr W last updated on 14/Nov/21
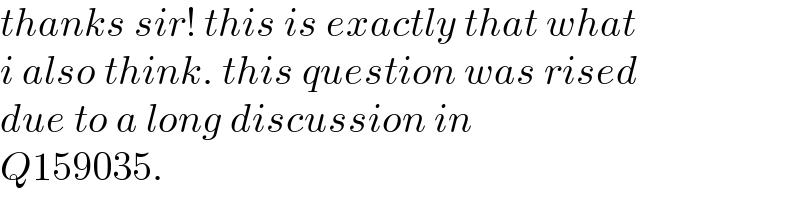
$${thanks}\:{sir}!\:{this}\:{is}\:{exactly}\:{that}\:{what} \\ $$$${i}\:{also}\:{think}.\:{this}\:{question}\:{was}\:{rised} \\ $$$${due}\:{to}\:{a}\:{long}\:{discussion}\:{in} \\ $$$${Q}\mathrm{159035}. \\ $$
Commented by MJS_new last updated on 14/Nov/21
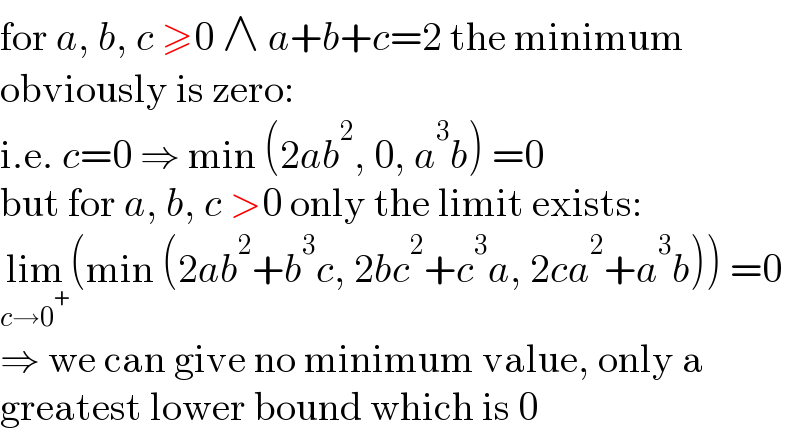
$$\mathrm{for}\:{a},\:{b},\:{c}\:\geqslant\mathrm{0}\:\wedge\:{a}+{b}+{c}=\mathrm{2}\:\mathrm{the}\:\mathrm{minimum} \\ $$$$\mathrm{obviously}\:\mathrm{is}\:\mathrm{zero}: \\ $$$$\mathrm{i}.\mathrm{e}.\:{c}=\mathrm{0}\:\Rightarrow\:\mathrm{min}\:\left(\mathrm{2}{ab}^{\mathrm{2}} ,\:\mathrm{0},\:{a}^{\mathrm{3}} {b}\right)\:=\mathrm{0} \\ $$$$\mathrm{but}\:\mathrm{for}\:{a},\:{b},\:{c}\:>\mathrm{0}\:\mathrm{only}\:\mathrm{the}\:\mathrm{limit}\:\mathrm{exists}: \\ $$$$\underset{{c}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}}\left(\mathrm{min}\:\left(\mathrm{2}{ab}^{\mathrm{2}} +{b}^{\mathrm{3}} {c},\:\mathrm{2}{bc}^{\mathrm{2}} +{c}^{\mathrm{3}} {a},\:\mathrm{2}{ca}^{\mathrm{2}} +{a}^{\mathrm{3}} {b}\right)\right)\:=\mathrm{0} \\ $$$$\Rightarrow\:\mathrm{we}\:\mathrm{can}\:\mathrm{give}\:\mathrm{no}\:\mathrm{minimum}\:\mathrm{value},\:\mathrm{only}\:\mathrm{a} \\ $$$$\mathrm{greatest}\:\mathrm{lower}\:\mathrm{bound}\:\mathrm{which}\:\mathrm{is}\:\mathrm{0} \\ $$
Commented by MJS_new last updated on 14/Nov/21

$$\mathrm{I}\:\mathrm{saw}\:\mathrm{that}… \\ $$
