Question Number 28138 by abdo imad last updated on 21/Jan/18
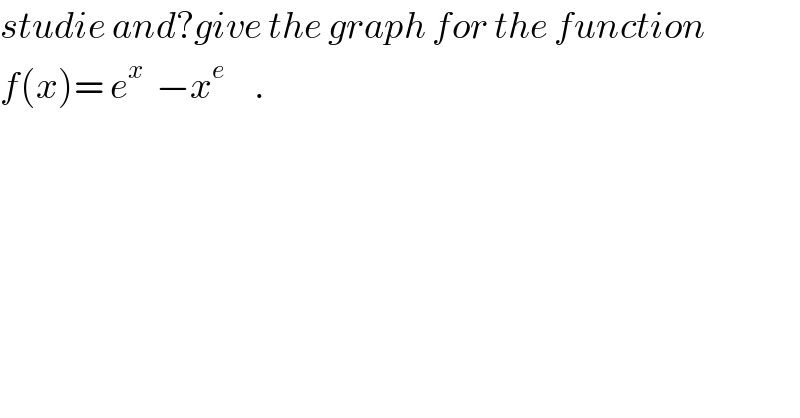
$${studie}\:{and}?{give}\:{the}\:{graph}\:{for}\:{the}\:{function} \\ $$$${f}\left({x}\right)=\:{e}^{{x}} \:\:−{x}^{{e}} \:\:\:\:\:. \\ $$
Commented by abdo imad last updated on 26/Jan/18
![we have f(x)= e^x −e^(elnx) ⇒ D_f =]0,+∝[ lim_(x→0^+ ) f(x)= 1 and lim_(x→+∞) f(x)=lim_(x→+∞) e^x ( 1−e^(−x+elnx) ) =lim_(x→+∞) e^x (1 −e^(−x(1−((elnx)/x))) )=lim _(x→+∞^ ) e^x =+∞ lim_(x→+∞) ((f(x))/x)= lim_(x→+∞) (e^x /x) − x^(e−1) =lim_(x→+∞) (e^x /x)( 1− e^(−x) x^e )=lim_(x→+∞) (e^x /x) =+∞ so the graph of f have a parabolic diction at +∞ f^′ (x)= e^x − e x^(e−1) = e^x (1− e^(−x+1) e^((e−1)lnx) ) = e^x ( 1−e^(−x+1 +(e−1)lnx) ) the sine off^′ (x) is thesine of ψ(x)= 1− e^((e−1)lnx −x+1) ψ^′ (x)= −( ((e−1)/x) −1) e^((...)) =−( ((e−1−x)/x))e^((...)) =((x−(e−1))/x) e^((...)) and ψ^′ (x)=0 ⇔ x=e−1 and f^′ (e−1)=e^(e−1) (1− e^(−e+2) e^((e−1)ln(e−1)) ).....](https://www.tinkutara.com/question/Q28472.png)
$$\left.{we}\:{have}\:{f}\left({x}\right)=\:{e}^{{x}} \:−{e}^{{elnx}} \:\:\Rightarrow\:{D}_{{f}} =\right]\mathrm{0},+\propto\left[\right. \\ $$$${lim}_{{x}\rightarrow\mathrm{0}^{+} } {f}\left({x}\right)=\:\mathrm{1}\:\:\:{and}\:{lim}_{{x}\rightarrow+\infty} {f}\left({x}\right)={lim}_{{x}\rightarrow+\infty} {e}^{{x}} \left(\:\mathrm{1}−{e}^{−{x}+{elnx}} \right) \\ $$$$={lim}_{{x}\rightarrow+\infty} \:{e}^{{x}} \left(\mathrm{1}\:−{e}^{−{x}\left(\mathrm{1}−\frac{{elnx}}{{x}}\right)} \right)={lim}\:_{{x}\rightarrow+\infty^{} } \:\:{e}^{{x}} =+\infty \\ $$$${lim}_{{x}\rightarrow+\infty} \:\frac{{f}\left({x}\right)}{{x}}=\:{lim}_{{x}\rightarrow+\infty} \:\frac{{e}^{{x}} }{{x}}\:−\:{x}^{{e}−\mathrm{1}} \\ $$$$={lim}_{{x}\rightarrow+\infty} \:\:\frac{{e}^{{x}} }{{x}}\left(\:\mathrm{1}−\:{e}^{−{x}} {x}^{{e}} \right)={lim}_{{x}\rightarrow+\infty} \:\frac{{e}^{{x}} }{{x}}\:=+\infty\:{so}\:{the}\:{graph}\: \\ $$$${of}\:{f}\:{have}\:{a}\:{parabolic}\:{diction}\:{at}\:+\infty \\ $$$${f}^{'} \left({x}\right)=\:{e}^{{x}} \:−\:{e}\:{x}^{{e}−\mathrm{1}} \:=\:{e}^{{x}} \:\left(\mathrm{1}−\:{e}^{−{x}+\mathrm{1}} \:{e}^{\left({e}−\mathrm{1}\right){lnx}} \right) \\ $$$$=\:{e}^{{x}} \left(\:\mathrm{1}−{e}^{−{x}+\mathrm{1}\:+\left({e}−\mathrm{1}\right){lnx}} \right)\:{the}\:{sine}\:{off}^{'} \left({x}\right)\:{is}\:{thesine}\:{of} \\ $$$$\psi\left({x}\right)=\:\mathrm{1}−\:{e}^{\left({e}−\mathrm{1}\right){lnx}\:−{x}+\mathrm{1}} \\ $$$$\psi^{'} \left({x}\right)=\:−\left(\:\frac{{e}−\mathrm{1}}{{x}}\:−\mathrm{1}\right)\:{e}^{\left(…\right)} \:=−\left(\:\frac{{e}−\mathrm{1}−{x}}{{x}}\right){e}^{\left(…\right)} \\ $$$$=\frac{{x}−\left({e}−\mathrm{1}\right)}{{x}}\:{e}^{\left(…\right)} \:\:\:{and}\:\:\psi^{'} \left({x}\right)=\mathrm{0}\:\Leftrightarrow\:\:{x}={e}−\mathrm{1}\:{and} \\ $$$${f}^{'} \left({e}−\mathrm{1}\right)={e}^{{e}−\mathrm{1}} \left(\mathrm{1}−\:{e}^{−{e}+\mathrm{2}} \:{e}^{\left({e}−\mathrm{1}\right){ln}\left({e}−\mathrm{1}\right)} \right)….. \\ $$
