Menu Close

simplify-n-1-1-k-1-n-k-3-

Tinku Tara 0 Comments
Pin




Question Number 159465 by mnjuly1970 last updated on 17/Nov/21
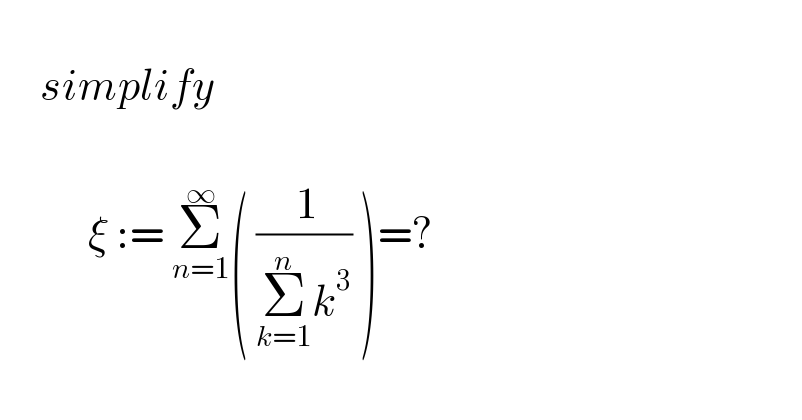
simplify ξ := Σ_(n=1) ^∞ ( (( 1)/(Σ_(k=1) ^n k^3 )) )=?
$$ \\ $$$$\:\:\:\:\:{simplify} \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\xi\::=\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\left(\:\frac{\:\mathrm{1}}{\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}^{\mathrm{3}} }\:\right)=? \\ $$$$ \\ $$
Answered by Ar Brandon last updated on 17/Nov/21
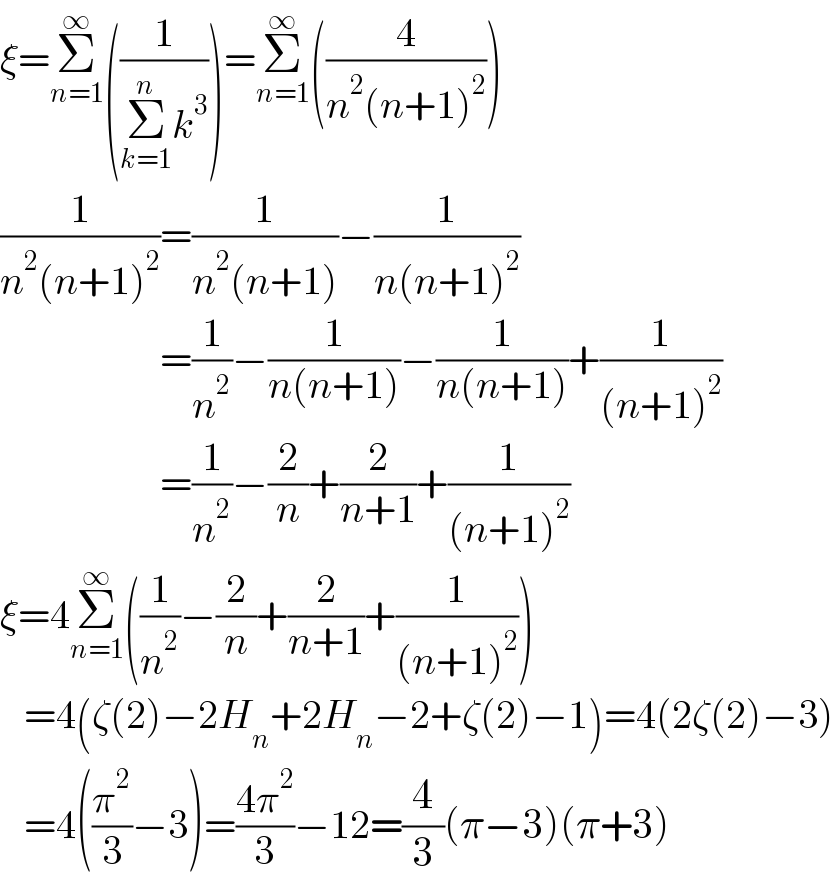
ξ=Σ_(n=1) ^∞ ((1/(Σ_(k=1) ^n k^3 )))=Σ_(n=1) ^∞ ((4/(n^2 (n+1)^2 ))) (1/(n^2 (n+1)^2 ))=(1/(n^2 (n+1)))−(1/(n(n+1)^2 )) =(1/n^2 )−(1/(n(n+1)))−(1/(n(n+1)))+(1/((n+1)^2 )) =(1/n^2 )−(2/n)+(2/(n+1))+(1/((n+1)^2 )) ξ=4Σ_(n=1) ^∞ ((1/n^2 )−(2/n)+(2/(n+1))+(1/((n+1)^2 ))) =4(ζ(2)−2H_n +2H_n −2+ζ(2)−1)=4(2ζ(2)−3) =4((π^2 /3)−3)=((4π^2 )/3)−12=(4/3)(π−3)(π+3)
$$\xi=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\left(\frac{\mathrm{1}}{\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}^{\mathrm{3}} }\right)=\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\left(\frac{\mathrm{4}}{{n}^{\mathrm{2}} \left({n}+\mathrm{1}\right)^{\mathrm{2}} }\right) \\ $$$$\frac{\mathrm{1}}{{n}^{\mathrm{2}} \left({n}+\mathrm{1}\right)^{\mathrm{2}} }=\frac{\mathrm{1}}{{n}^{\mathrm{2}} \left({n}+\mathrm{1}\right)}−\frac{\mathrm{1}}{{n}\left({n}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{{n}^{\mathrm{2}} }−\frac{\mathrm{1}}{{n}\left({n}+\mathrm{1}\right)}−\frac{\mathrm{1}}{{n}\left({n}+\mathrm{1}\right)}+\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{1}}{{n}^{\mathrm{2}} }−\frac{\mathrm{2}}{{n}}+\frac{\mathrm{2}}{{n}+\mathrm{1}}+\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$$\xi=\mathrm{4}\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\left(\frac{\mathrm{1}}{{n}^{\mathrm{2}} }−\frac{\mathrm{2}}{{n}}+\frac{\mathrm{2}}{{n}+\mathrm{1}}+\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)^{\mathrm{2}} }\right) \\ $$$$\:\:\:=\mathrm{4}\left(\zeta\left(\mathrm{2}\right)−\mathrm{2}{H}_{{n}} +\mathrm{2}{H}_{{n}} −\mathrm{2}+\zeta\left(\mathrm{2}\right)−\mathrm{1}\right)=\mathrm{4}\left(\mathrm{2}\zeta\left(\mathrm{2}\right)−\mathrm{3}\right) \\ $$$$\:\:\:=\mathrm{4}\left(\frac{\pi^{\mathrm{2}} }{\mathrm{3}}−\mathrm{3}\right)=\frac{\mathrm{4}\pi^{\mathrm{2}} }{\mathrm{3}}−\mathrm{12}=\frac{\mathrm{4}}{\mathrm{3}}\left(\pi−\mathrm{3}\right)\left(\pi+\mathrm{3}\right) \\ $$
Commented by mnjuly1970 last updated on 17/Nov/21
thanks alot sir brandon.mercey
$$\:\:\:{thanks}\:{alot}\:{sir}\:{brandon}.{mercey} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *