Question Number 29134 by Tinkutara last updated on 04/Feb/18

Commented by ajfour last updated on 04/Feb/18
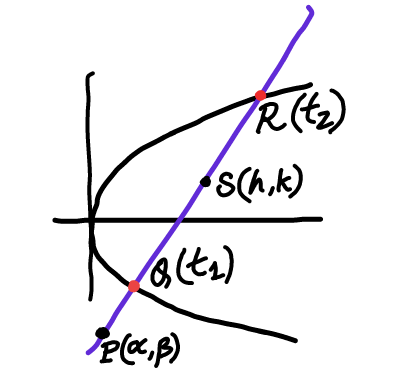
Commented by ajfour last updated on 04/Feb/18
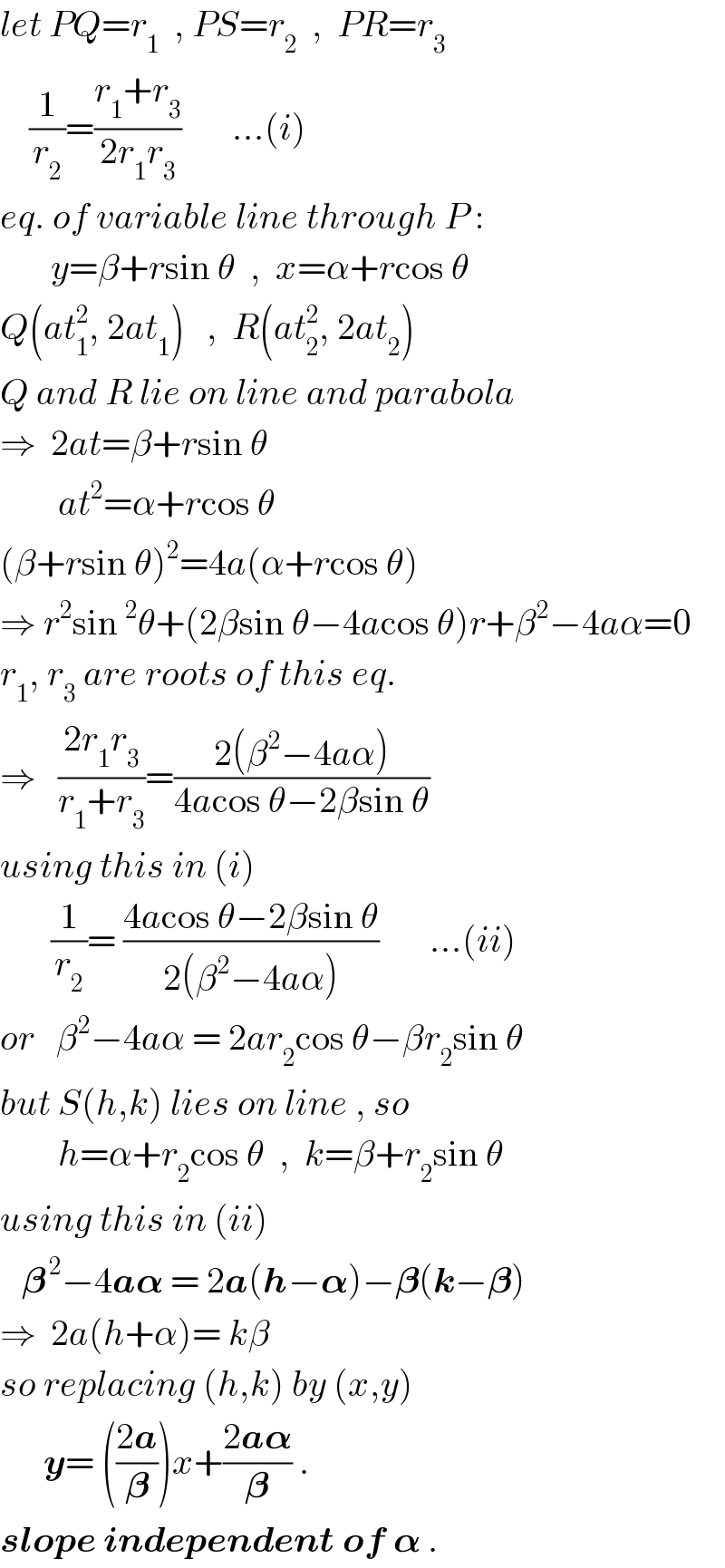
$${let}\:{PQ}={r}_{\mathrm{1}} \:\:,\:{PS}={r}_{\mathrm{2}} \:\:,\:\:{PR}={r}_{\mathrm{3}} \\ $$$$\:\:\:\:\frac{\mathrm{1}}{{r}_{\mathrm{2}} }=\frac{{r}_{\mathrm{1}} +{r}_{\mathrm{3}} }{\mathrm{2}{r}_{\mathrm{1}} {r}_{\mathrm{3}} }\:\:\:\:\:\:\:…\left({i}\right) \\ $$$${eq}.\:{of}\:{variable}\:{line}\:{through}\:{P}\:: \\ $$$$\:\:\:\:\:\:\:{y}=\beta+{r}\mathrm{sin}\:\theta\:\:,\:\:{x}=\alpha+{r}\mathrm{cos}\:\theta \\ $$$${Q}\left({at}_{\mathrm{1}} ^{\mathrm{2}} ,\:\mathrm{2}{at}_{\mathrm{1}} \right)\:\:\:,\:\:{R}\left({at}_{\mathrm{2}} ^{\mathrm{2}} ,\:\mathrm{2}{at}_{\mathrm{2}} \right) \\ $$$${Q}\:{and}\:{R}\:{lie}\:{on}\:{line}\:{and}\:{parabola} \\ $$$$\Rightarrow\:\:\mathrm{2}{at}=\beta+{r}\mathrm{sin}\:\theta \\ $$$$\:\:\:\:\:\:\:\:{at}^{\mathrm{2}} =\alpha+{r}\mathrm{cos}\:\theta \\ $$$$\left(\beta+{r}\mathrm{sin}\:\theta\right)^{\mathrm{2}} =\mathrm{4}{a}\left(\alpha+{r}\mathrm{cos}\:\theta\right) \\ $$$$\Rightarrow\:{r}^{\mathrm{2}} \mathrm{sin}\:^{\mathrm{2}} \theta+\left(\mathrm{2}\beta\mathrm{sin}\:\theta−\mathrm{4}{a}\mathrm{cos}\:\theta\right){r}+\beta^{\mathrm{2}} −\mathrm{4}{a}\alpha=\mathrm{0} \\ $$$${r}_{\mathrm{1}} ,\:{r}_{\mathrm{3}} \:{are}\:{roots}\:{of}\:{this}\:{eq}. \\ $$$$\Rightarrow\:\:\:\frac{\mathrm{2}{r}_{\mathrm{1}} {r}_{\mathrm{3}} }{{r}_{\mathrm{1}} +{r}_{\mathrm{3}} }=\frac{\mathrm{2}\left(\beta^{\mathrm{2}} −\mathrm{4}{a}\alpha\right)}{\mathrm{4}{a}\mathrm{cos}\:\theta−\mathrm{2}\beta\mathrm{sin}\:\theta} \\ $$$${using}\:{this}\:{in}\:\left({i}\right) \\ $$$$\:\:\:\:\:\:\:\frac{\mathrm{1}}{{r}_{\mathrm{2}} }=\:\frac{\mathrm{4}{a}\mathrm{cos}\:\theta−\mathrm{2}\beta\mathrm{sin}\:\theta}{\mathrm{2}\left(\beta^{\mathrm{2}} −\mathrm{4}{a}\alpha\right)}\:\:\:\:\:\:\:…\left({ii}\right) \\ $$$${or}\:\:\:\beta^{\mathrm{2}} −\mathrm{4}{a}\alpha\:=\:\mathrm{2}{ar}_{\mathrm{2}} \mathrm{cos}\:\theta−\beta{r}_{\mathrm{2}} \mathrm{sin}\:\theta \\ $$$${but}\:{S}\left({h},{k}\right)\:{lies}\:{on}\:{line}\:,\:{so} \\ $$$$\:\:\:\:\:\:\:\:{h}=\alpha+{r}_{\mathrm{2}} \mathrm{cos}\:\theta\:\:,\:\:{k}=\beta+{r}_{\mathrm{2}} \mathrm{sin}\:\theta \\ $$$${using}\:{this}\:{in}\:\left({ii}\right) \\ $$$$\:\:\:\boldsymbol{\beta}^{\:\mathrm{2}} −\mathrm{4}\boldsymbol{{a}\alpha}\:=\:\mathrm{2}\boldsymbol{{a}}\left(\boldsymbol{{h}}−\boldsymbol{\alpha}\right)−\boldsymbol{\beta}\left(\boldsymbol{{k}}−\boldsymbol{\beta}\right) \\ $$$$\Rightarrow\:\:\mathrm{2}{a}\left({h}+\alpha\right)=\:{k}\beta \\ $$$${so}\:{replacing}\:\left({h},{k}\right)\:{by}\:\left({x},{y}\right) \\ $$$$\:\:\:\:\:\:\boldsymbol{{y}}=\:\left(\frac{\mathrm{2}\boldsymbol{{a}}}{\boldsymbol{\beta}}\right){x}+\frac{\mathrm{2}\boldsymbol{{a}\alpha}}{\boldsymbol{\beta}}\:. \\ $$$$\boldsymbol{{slope}}\:\boldsymbol{{independent}}\:\boldsymbol{{of}}\:\boldsymbol{\alpha}\:. \\ $$
Commented by Tinkutara last updated on 05/Feb/18
Wonderful Sir! ����
Thanks!
��
