Question Number 95060 by EmericGent last updated on 22/May/20
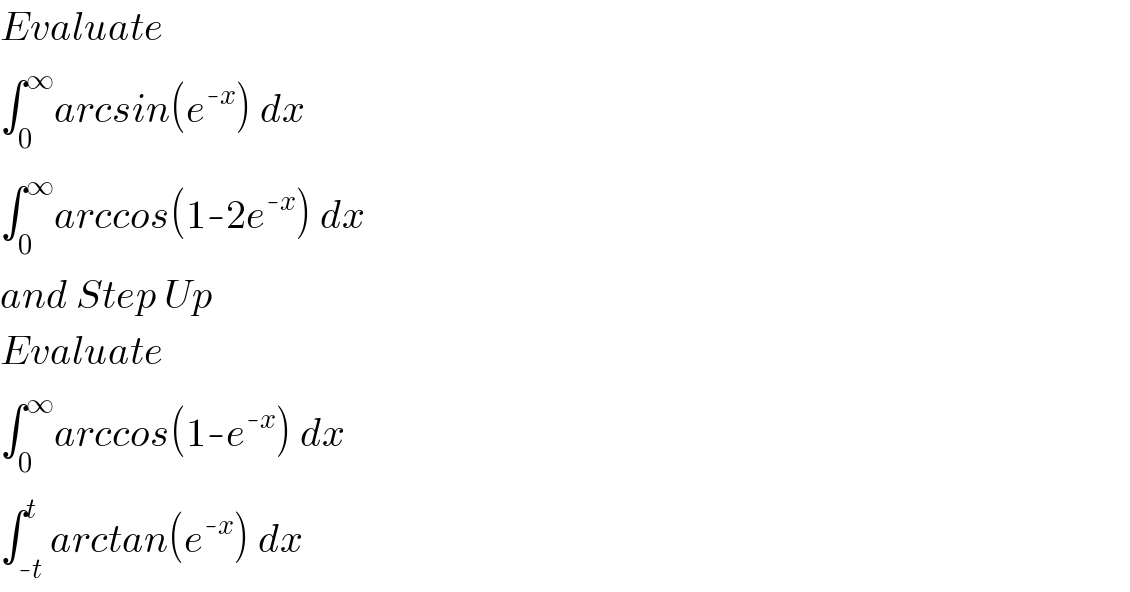
$${Evaluate} \\ $$$$\int_{\mathrm{0}} ^{\infty} {arcsin}\left({e}^{-{x}} \right)\:{dx} \\ $$$$\int_{\mathrm{0}} ^{\infty} {arccos}\left(\mathrm{1}-\mathrm{2}{e}^{-{x}} \right)\:{dx} \\ $$$${and}\:{Step}\:{Up} \\ $$$${Evaluate} \\ $$$$\int_{\mathrm{0}} ^{\infty} {arccos}\left(\mathrm{1}-{e}^{-{x}} \right)\:{dx} \\ $$$$\int_{-{t}} ^{{t}} {arctan}\left({e}^{-{x}} \right)\:{dx} \\ $$
Answered by mathmax by abdo last updated on 23/May/20
![let take a try I = ∫_0 ^∞ arcsin(e^(−x) )dx changement e^(−x) =t give x =−lnt ⇒ I =∫_0 ^1 arcsin(t)(dt/t) =∫_0 ^1 ((arcsin(t))/t)dt let f(x) =∫_0 ^(1 ) ((arcsin(xt))/t)dt (x>0) f^′ (x) =∫_0 ^1 (t/(t(√(1−x^2 t^2 )))) dt =∫_0 ^1 (dt/( (√(1−(xt)^2 )))) =_(xt =sinα) ∫_0 ^(arcsinx) (1/(x cosα))×(1/x)cosα dα =((arcsin(x))/x^2 ) ⇒ f(x) =∫_1 ^x ((arcsin(u))/u^2 ) du +c =[−(1/u) arcsinu]_1 ^x −∫_1 ^x (−(1/u))×(1/( (√(1−u^2 )))) du+c =(π/2) −((arcsinx)/x) +∫_1 ^x (du/(u(√(1−u^2 )))) but ∫_1 ^x (du/(u(√(1−u^2 )))) =_(u=sinz) ∫_(π/2) ^(arcsinx) ((cosz)/(sinz cosz)) dz =∫_(π/2) ^(arcsinx) (dz/(sinz)) =_(tan((z/2)) =λ) ∫_∞ ^(tan(((arcsinx)/2))) ((2dλ)/((1+λ^2 )×((2λ)/(1+λ^2 )))) =−∫_(tan(((arcsinx)/2))) (dλ/λ) =−ln∣tan(((arcsinx)/2))∣ ⇒ f(x) =(π/2) −((arcsinx)/x) −ln∣tan(((arcsinx)/2))∣ +c f(1) =c =∫_0 ^1 ((arcsint)/t) dt ⇒ f(x) =(π/2) −((arcsinx)/x) −ln∣tan(((arcsinx)/2))∣ +∫_0 ^1 ((arcsint)/t) dt for x>0 f((1/x)) =(π/2)−(((π/2)−arcosx)/x) −ln∣tan((((π/2)−arcosx)/2))∣+∫_0 ^1 ((arcsint)/t)dt =(π/2)−(π/(2x)) +((arcosx)/x)−ln∣tan((π/4)−((arcosx)/2))∣ +∫_0 ^1 ((arcsint)/t) dt ...be continued...](https://www.tinkutara.com/question/Q95096.png)
$$\mathrm{let}\:\mathrm{take}\:\mathrm{a}\:\mathrm{try}\:\:\mathrm{I}\:=\:\int_{\mathrm{0}} ^{\infty} \:\mathrm{arcsin}\left(\mathrm{e}^{−\mathrm{x}} \right)\mathrm{dx}\:\:\mathrm{changement}\:\mathrm{e}^{−\mathrm{x}} \:=\mathrm{t}\:\mathrm{give}\:\mathrm{x}\:=−\mathrm{lnt}\:\Rightarrow \\ $$$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\mathrm{arcsin}\left(\mathrm{t}\right)\frac{\mathrm{dt}}{\mathrm{t}}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{arcsin}\left(\mathrm{t}\right)}{\mathrm{t}}\mathrm{dt}\:\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}\:} \:\frac{\mathrm{arcsin}\left(\mathrm{xt}\right)}{\mathrm{t}}\mathrm{dt}\:\left(\mathrm{x}>\mathrm{0}\right) \\ $$$$\mathrm{f}^{'} \left(\mathrm{x}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\frac{\mathrm{t}}{\mathrm{t}\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} \mathrm{t}^{\mathrm{2}} }}\:\mathrm{dt}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{dt}}{\:\sqrt{\mathrm{1}−\left(\mathrm{xt}\right)^{\mathrm{2}} }}\:=_{\mathrm{xt}\:=\mathrm{sin}\alpha} \:\:\int_{\mathrm{0}} ^{\mathrm{arcsinx}} \:\frac{\mathrm{1}}{\mathrm{x}\:\mathrm{cos}\alpha}×\frac{\mathrm{1}}{\mathrm{x}}\mathrm{cos}\alpha\:\mathrm{d}\alpha \\ $$$$=\frac{\mathrm{arcsin}\left(\mathrm{x}\right)}{\mathrm{x}^{\mathrm{2}} }\:\:\:\Rightarrow\:\mathrm{f}\left(\mathrm{x}\right)\:=\int_{\mathrm{1}} ^{\mathrm{x}} \:\frac{\mathrm{arcsin}\left(\mathrm{u}\right)}{\mathrm{u}^{\mathrm{2}} }\:\mathrm{du}\:+\mathrm{c} \\ $$$$=\left[−\frac{\mathrm{1}}{\mathrm{u}}\:\mathrm{arcsinu}\right]_{\mathrm{1}} ^{\mathrm{x}} \:−\int_{\mathrm{1}} ^{\mathrm{x}} \:\left(−\frac{\mathrm{1}}{\mathrm{u}}\right)×\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }}\:\mathrm{du}+\mathrm{c} \\ $$$$=\frac{\pi}{\mathrm{2}}\:−\frac{\mathrm{arcsinx}}{\mathrm{x}}\:+\int_{\mathrm{1}} ^{\mathrm{x}} \:\frac{\mathrm{du}}{\mathrm{u}\sqrt{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }}\:\mathrm{but} \\ $$$$\int_{\mathrm{1}} ^{\mathrm{x}} \:\frac{\mathrm{du}}{\mathrm{u}\sqrt{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }}\:=_{\mathrm{u}=\mathrm{sinz}} \:\:\:\:\int_{\frac{\pi}{\mathrm{2}}} ^{\mathrm{arcsinx}} \:\:\frac{\mathrm{cosz}}{\mathrm{sinz}\:\mathrm{cosz}}\:\mathrm{dz}\:=\int_{\frac{\pi}{\mathrm{2}}} ^{\mathrm{arcsinx}} \:\frac{\mathrm{dz}}{\mathrm{sinz}} \\ $$$$=_{\mathrm{tan}\left(\frac{\mathrm{z}}{\mathrm{2}}\right)\:=\lambda} \:\:\:\:\:\int_{\infty} ^{\mathrm{tan}\left(\frac{\mathrm{arcsinx}}{\mathrm{2}}\right)} \:\frac{\mathrm{2d}\lambda}{\left(\mathrm{1}+\lambda^{\mathrm{2}} \right)×\frac{\mathrm{2}\lambda}{\mathrm{1}+\lambda^{\mathrm{2}} }}\:=−\int_{\mathrm{tan}\left(\frac{\mathrm{arcsinx}}{\mathrm{2}}\right)} \:\:\frac{\mathrm{d}\lambda}{\lambda} \\ $$$$=−\mathrm{ln}\mid\mathrm{tan}\left(\frac{\mathrm{arcsinx}}{\mathrm{2}}\right)\mid\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{x}\right)\:=\frac{\pi}{\mathrm{2}}\:−\frac{\mathrm{arcsinx}}{\mathrm{x}}\:−\mathrm{ln}\mid\mathrm{tan}\left(\frac{\mathrm{arcsinx}}{\mathrm{2}}\right)\mid\:+\mathrm{c} \\ $$$$\mathrm{f}\left(\mathrm{1}\right)\:=\mathrm{c}\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{arcsint}}{\mathrm{t}}\:\mathrm{dt}\:\Rightarrow \\ $$$$\mathrm{f}\left(\mathrm{x}\right)\:=\frac{\pi}{\mathrm{2}}\:−\frac{\mathrm{arcsinx}}{\mathrm{x}}\:−\mathrm{ln}\mid\mathrm{tan}\left(\frac{\mathrm{arcsinx}}{\mathrm{2}}\right)\mid\:+\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{arcsint}}{\mathrm{t}}\:\mathrm{dt}\:\mathrm{for}\:\mathrm{x}>\mathrm{0} \\ $$$$\mathrm{f}\left(\frac{\mathrm{1}}{\mathrm{x}}\right)\:=\frac{\pi}{\mathrm{2}}−\frac{\frac{\pi}{\mathrm{2}}−\mathrm{arcosx}}{\mathrm{x}}\:−\mathrm{ln}\mid\mathrm{tan}\left(\frac{\frac{\pi}{\mathrm{2}}−\mathrm{arcosx}}{\mathrm{2}}\right)\mid+\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{arcsint}}{\mathrm{t}}\mathrm{dt} \\ $$$$=\frac{\pi}{\mathrm{2}}−\frac{\pi}{\mathrm{2x}}\:+\frac{\mathrm{arcosx}}{\mathrm{x}}−\mathrm{ln}\mid\mathrm{tan}\left(\frac{\pi}{\mathrm{4}}−\frac{\mathrm{arcosx}}{\mathrm{2}}\right)\mid\:+\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{arcsint}}{\mathrm{t}}\:\mathrm{dt} \\ $$$$…\mathrm{be}\:\mathrm{continued}… \\ $$
Commented by mathmax by abdo last updated on 23/May/20
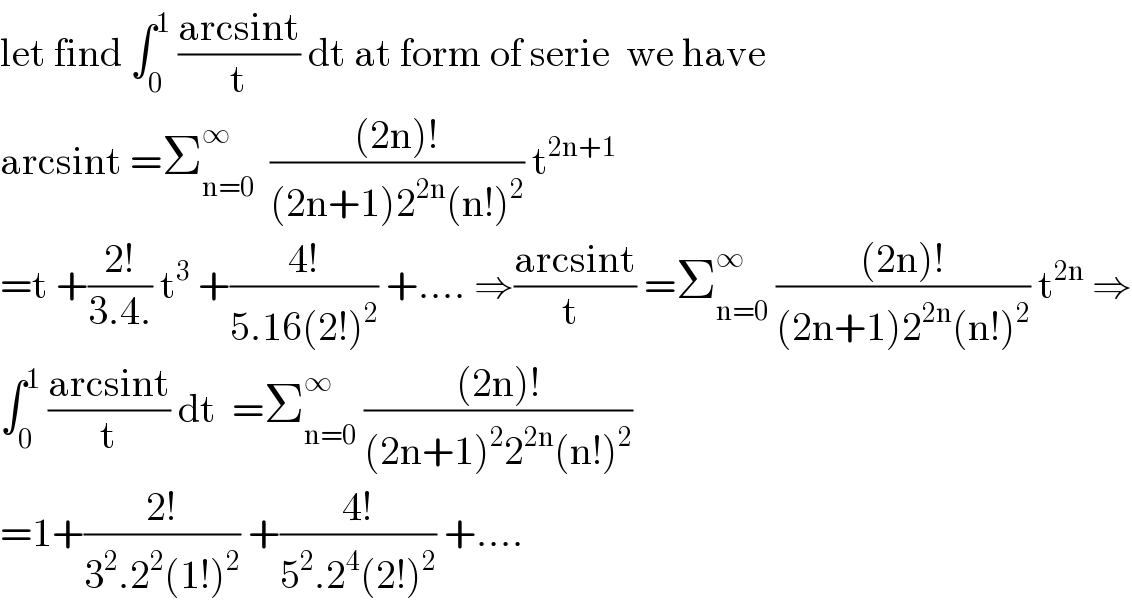
$$\mathrm{let}\:\mathrm{find}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{arcsint}}{\mathrm{t}}\:\mathrm{dt}\:\mathrm{at}\:\mathrm{form}\:\mathrm{of}\:\mathrm{serie}\:\:\mathrm{we}\:\mathrm{have} \\ $$$$\mathrm{arcsint}\:=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\:\frac{\left(\mathrm{2n}\right)!}{\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{2}^{\mathrm{2n}} \left(\mathrm{n}!\right)^{\mathrm{2}} }\:\mathrm{t}^{\mathrm{2n}+\mathrm{1}} \\ $$$$=\mathrm{t}\:+\frac{\mathrm{2}!}{\mathrm{3}.\mathrm{4}.}\:\mathrm{t}^{\mathrm{3}} \:+\frac{\mathrm{4}!}{\mathrm{5}.\mathrm{16}\left(\mathrm{2}!\right)^{\mathrm{2}} }\:+….\:\Rightarrow\frac{\mathrm{arcsint}}{\mathrm{t}\:}\:=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\left(\mathrm{2n}\right)!}{\left(\mathrm{2n}+\mathrm{1}\right)\mathrm{2}^{\mathrm{2n}} \left(\mathrm{n}!\right)^{\mathrm{2}} }\:\mathrm{t}^{\mathrm{2n}} \:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{\mathrm{arcsint}}{\mathrm{t}}\:\mathrm{dt}\:\:=\sum_{\mathrm{n}=\mathrm{0}} ^{\infty} \:\frac{\left(\mathrm{2n}\right)!}{\left(\mathrm{2n}+\mathrm{1}\right)^{\mathrm{2}} \mathrm{2}^{\mathrm{2n}} \left(\mathrm{n}!\right)^{\mathrm{2}} } \\ $$$$=\mathrm{1}+\frac{\mathrm{2}!}{\mathrm{3}^{\mathrm{2}} .\mathrm{2}^{\mathrm{2}} \left(\mathrm{1}!\right)^{\mathrm{2}} }\:+\frac{\mathrm{4}!}{\mathrm{5}^{\mathrm{2}} .\mathrm{2}^{\mathrm{4}} \left(\mathrm{2}!\right)^{\mathrm{2}} }\:+…. \\ $$
Commented by EmericGent last updated on 23/May/20
I used a much easier way (I show you my start)
Commented by EmericGent last updated on 23/May/20

Commented by mathmax by abdo last updated on 23/May/20
![A(y) =∫_0 ^y arcsin(e^(−x) )dx by parts A(y) =[ x arcsin(e^(−x) )]_0 ^y −∫_0 ^y x (((−e^(−x) )/( (√(1−e^(−2x) )))))dx =y arcsin(e^(−y) ) +∫_0 ^y ((xe^(−x) )/( (√(1−e^(−2x) ))))dx e^(−x) =sinα ⇒−x =ln(sinα)⇒ ∫_0 ^y ((xe^(−x) )/( (√(1−e^(−2x) ))))dx =∫_(π/2) ^(arcsin(e^(−y) )) (((ln(sinα))sinα)/(cosα))×((cosα)/(sinα)) dα =−∫_(arcsin(e^(−y) )) ^(π/2) ln(sinα)dα ⇒A(y) =y arcsin(e^(−y) )−∫_(arcsin(e^(−y) )) ^(π/2) ln(sinα)dα lim_(y→+∞) A(y) =−∫_0 ^(π/2) ln(sinα)dα =−(−(π/2)ln(2)) ⇒ ∫_0 ^∞ arcsin(e^(−x) )dx =(π/2)ln(2)](https://www.tinkutara.com/question/Q95184.png)
$$\mathrm{A}\left(\mathrm{y}\right)\:=\int_{\mathrm{0}} ^{\mathrm{y}} \:\mathrm{arcsin}\left(\mathrm{e}^{−\mathrm{x}} \right)\mathrm{dx}\:\:\:\mathrm{by}\:\mathrm{parts} \\ $$$$\mathrm{A}\left(\mathrm{y}\right)\:=\left[\:\mathrm{x}\:\mathrm{arcsin}\left(\mathrm{e}^{−\mathrm{x}} \right)\right]_{\mathrm{0}} ^{\mathrm{y}} \:−\int_{\mathrm{0}} ^{\mathrm{y}} \:\mathrm{x}\:\left(\frac{−\mathrm{e}^{−\mathrm{x}} }{\:\sqrt{\mathrm{1}−\mathrm{e}^{−\mathrm{2x}} }}\right)\mathrm{dx} \\ $$$$=\mathrm{y}\:\mathrm{arcsin}\left(\mathrm{e}^{−\mathrm{y}} \right)\:+\int_{\mathrm{0}} ^{\mathrm{y}} \:\frac{\mathrm{xe}^{−\mathrm{x}} }{\:\sqrt{\mathrm{1}−\mathrm{e}^{−\mathrm{2x}} }}\mathrm{dx}\:\:\:\:\mathrm{e}^{−\mathrm{x}} \:=\mathrm{sin}\alpha\:\Rightarrow−\mathrm{x}\:=\mathrm{ln}\left(\mathrm{sin}\alpha\right)\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\mathrm{y}} \:\frac{\mathrm{xe}^{−\mathrm{x}} }{\:\sqrt{\mathrm{1}−\mathrm{e}^{−\mathrm{2x}} }}\mathrm{dx}\:=\int_{\frac{\pi}{\mathrm{2}}} ^{\mathrm{arcsin}\left(\mathrm{e}^{−\mathrm{y}} \right)} \:\:\frac{\left(\mathrm{ln}\left(\mathrm{sin}\alpha\right)\right)\mathrm{sin}\alpha}{\mathrm{cos}\alpha}×\frac{\mathrm{cos}\alpha}{\mathrm{sin}\alpha}\:\mathrm{d}\alpha \\ $$$$=−\int_{\mathrm{arcsin}\left(\mathrm{e}^{−\mathrm{y}} \right)} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{sin}\alpha\right)\mathrm{d}\alpha\:\Rightarrow\mathrm{A}\left(\mathrm{y}\right)\:=\mathrm{y}\:\mathrm{arcsin}\left(\mathrm{e}^{−\mathrm{y}} \right)−\int_{\mathrm{arcsin}\left(\mathrm{e}^{−\mathrm{y}} \right)} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{sin}\alpha\right)\mathrm{d}\alpha \\ $$$$\mathrm{lim}_{\mathrm{y}\rightarrow+\infty} \:\mathrm{A}\left(\mathrm{y}\right)\:=−\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{sin}\alpha\right)\mathrm{d}\alpha\:=−\left(−\frac{\pi}{\mathrm{2}}\mathrm{ln}\left(\mathrm{2}\right)\right)\:\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\infty} \mathrm{arcsin}\left(\mathrm{e}^{−\mathrm{x}} \right)\mathrm{dx}\:=\frac{\pi}{\mathrm{2}}\mathrm{ln}\left(\mathrm{2}\right) \\ $$
Commented by mathmax by abdo last updated on 23/May/20
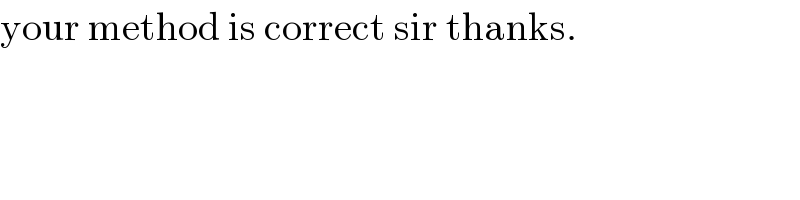
$$\mathrm{your}\:\mathrm{method}\:\mathrm{is}\:\mathrm{correct}\:\mathrm{sir}\:\mathrm{thanks}. \\ $$
Commented by EmericGent last updated on 23/May/20
I did the second and the third by the same way (actually I'm a boy)
Answered by abdomathmax last updated on 23/May/20
![A(y) =∫_0 ^y arcos(1−2e^(−x) )dx by parts A(y) =[x arcos(1−2e^(−x) )]_0 ^y +∫_0 ^y x×((2e^(−x) )/( (√(1−(1−2e^(−x) )^2 ))))dx =y arcos(1−2e^(−y) ) +2 ∫_0 ^y ((xe^(−x) )/( (√(1−(1−2e^(−x) )^2 ))))dx changement 1−2e^(−x) = cosα give 2e^(−x) =1−cosα ⇒e^(−x) =((1−cosα)/2) ⇒x=−ln(((1−cosα)/2)) ⇒dx =−((sinα)/(1−cosα)) ⇒ ∫_0 ^y ((xe^(−x) )/( (√(1−(1−2e^(−x) )^2 ))))dx =∫_π ^(arcos(1−2e^(−y) )) −ln(((1−cosα)/2))×((1−cosα)/2)×(−((sinα)/(1−cosα)))×(1/(sinα))dα =(1/2)∫_π ^(arcos(1−2e^(−y) )) ln(((1−cosα)/2))dα =(1/2) ∫_π ^(arcos(1−2e^(−y) )) 2ln(cos((α/2)))dα =_((α/2)=t) 2∫_(π/2) ^((1/2)arcos(1−2e^(−y) )) ln(cost) dt →2∫_(π/2) ^0 ln(cost)dt =−2∫_0 ^(π/2) ln(cost)dt =−2(−(π/2)ln2) =πln(2) also yarcos(1−2e^(−y) )→0 ⇒ ∫_0 ^∞ arcos(1−2e^(−x) )dx =lim_(y→+∞) A(y) =πln(2)](https://www.tinkutara.com/question/Q95192.png)
$$\mathrm{A}\left(\mathrm{y}\right)\:=\int_{\mathrm{0}} ^{\mathrm{y}} \:\mathrm{arcos}\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{x}} \right)\mathrm{dx}\:\:\mathrm{by}\:\mathrm{parts} \\ $$$$\mathrm{A}\left(\mathrm{y}\right)\:=\left[\mathrm{x}\:\mathrm{arcos}\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{x}} \right)\right]_{\mathrm{0}} ^{\mathrm{y}} +\int_{\mathrm{0}} ^{\mathrm{y}} \:\mathrm{x}×\frac{\mathrm{2e}^{−\mathrm{x}} }{\:\sqrt{\mathrm{1}−\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{x}} \right)^{\mathrm{2}} }}\mathrm{dx} \\ $$$$=\mathrm{y}\:\mathrm{arcos}\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{y}} \right)\:+\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{y}} \:\frac{\mathrm{xe}^{−\mathrm{x}} }{\:\sqrt{\mathrm{1}−\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{x}} \right)^{\mathrm{2}} }}\mathrm{dx} \\ $$$$\mathrm{changement}\:\mathrm{1}−\mathrm{2e}^{−\mathrm{x}} \:=\:\mathrm{cos}\alpha\:\mathrm{give} \\ $$$$\mathrm{2e}^{−\mathrm{x}} \:=\mathrm{1}−\mathrm{cos}\alpha\:\Rightarrow\mathrm{e}^{−\mathrm{x}} \:=\frac{\mathrm{1}−\mathrm{cos}\alpha}{\mathrm{2}}\:\Rightarrow\mathrm{x}=−\mathrm{ln}\left(\frac{\mathrm{1}−\mathrm{cos}\alpha}{\mathrm{2}}\right) \\ $$$$\Rightarrow\mathrm{dx}\:=−\frac{\mathrm{sin}\alpha}{\mathrm{1}−\mathrm{cos}\alpha}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\mathrm{y}} \:\frac{\mathrm{xe}^{−\mathrm{x}} }{\:\sqrt{\mathrm{1}−\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{x}} \right)^{\mathrm{2}} }}\mathrm{dx} \\ $$$$=\int_{\pi} ^{\mathrm{arcos}\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{y}} \right)} −\mathrm{ln}\left(\frac{\mathrm{1}−\mathrm{cos}\alpha}{\mathrm{2}}\right)×\frac{\mathrm{1}−\mathrm{cos}\alpha}{\mathrm{2}}×\left(−\frac{\mathrm{sin}\alpha}{\mathrm{1}−\mathrm{cos}\alpha}\right)×\frac{\mathrm{1}}{\mathrm{sin}\alpha}\mathrm{d}\alpha \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\int_{\pi} ^{\mathrm{arcos}\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{y}} \right)} \:\mathrm{ln}\left(\frac{\mathrm{1}−\mathrm{cos}\alpha}{\mathrm{2}}\right)\mathrm{d}\alpha \\ $$$$=\frac{\mathrm{1}}{\mathrm{2}}\:\int_{\pi} ^{\mathrm{arcos}\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{y}} \right)} \mathrm{2ln}\left(\mathrm{cos}\left(\frac{\alpha}{\mathrm{2}}\right)\right)\mathrm{d}\alpha \\ $$$$=_{\frac{\alpha}{\mathrm{2}}=\mathrm{t}} \:\:\mathrm{2}\int_{\frac{\pi}{\mathrm{2}}} ^{\frac{\mathrm{1}}{\mathrm{2}}\mathrm{arcos}\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{y}} \right)} \:\:\mathrm{ln}\left(\mathrm{cost}\right)\:\mathrm{dt} \\ $$$$\rightarrow\mathrm{2}\int_{\frac{\pi}{\mathrm{2}}} ^{\mathrm{0}} \:\mathrm{ln}\left(\mathrm{cost}\right)\mathrm{dt}\:=−\mathrm{2}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \mathrm{ln}\left(\mathrm{cost}\right)\mathrm{dt}\:=−\mathrm{2}\left(−\frac{\pi}{\mathrm{2}}\mathrm{ln2}\right) \\ $$$$=\pi\mathrm{ln}\left(\mathrm{2}\right)\:\mathrm{also}\:\mathrm{yarcos}\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{y}} \right)\rightarrow\mathrm{0}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\infty} \:\mathrm{arcos}\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{x}} \right)\mathrm{dx}\:=\mathrm{lim}_{\mathrm{y}\rightarrow+\infty} \mathrm{A}\left(\mathrm{y}\right)\:=\pi\mathrm{ln}\left(\mathrm{2}\right) \\ $$$$ \\ $$$$ \\ $$
Commented by EmericGent last updated on 23/May/20
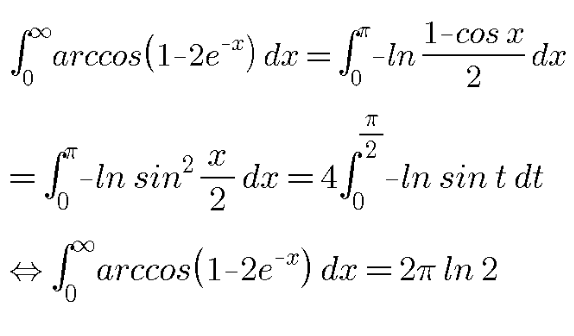
Commented by EmericGent last updated on 23/May/20
Actually you did a small mistake (forgot a *2 somewhere)
Commented by mathmax by abdo last updated on 24/May/20
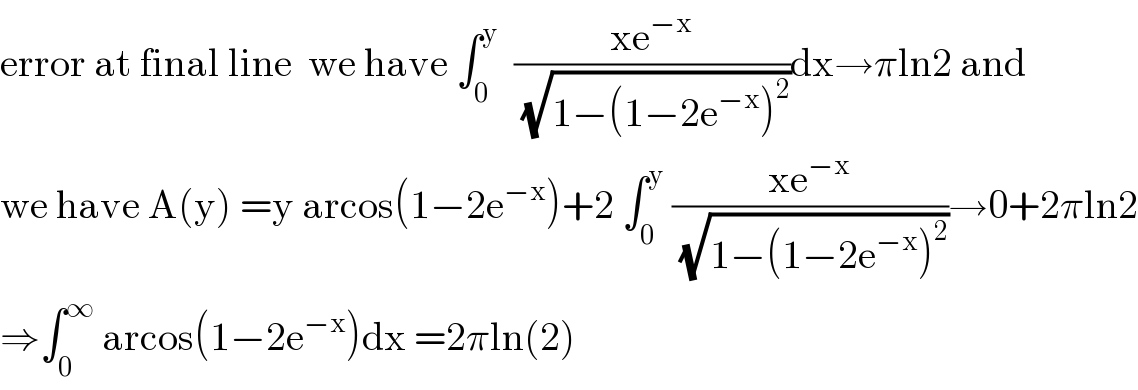
$$\mathrm{error}\:\mathrm{at}\:\mathrm{final}\:\mathrm{line}\:\:\mathrm{we}\:\mathrm{have}\:\int_{\mathrm{0}} ^{\mathrm{y}} \:\:\frac{\mathrm{xe}^{−\mathrm{x}} }{\:\sqrt{\mathrm{1}−\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{x}} \right)^{\mathrm{2}} }}\mathrm{dx}\rightarrow\pi\mathrm{ln2}\:\mathrm{and} \\ $$$$\mathrm{we}\:\mathrm{have}\:\mathrm{A}\left(\mathrm{y}\right)\:=\mathrm{y}\:\mathrm{arcos}\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{x}} \right)+\mathrm{2}\:\int_{\mathrm{0}} ^{\mathrm{y}} \:\frac{\mathrm{xe}^{−\mathrm{x}} }{\:\sqrt{\mathrm{1}−\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{x}} \right)^{\mathrm{2}} }}\rightarrow\mathrm{0}+\mathrm{2}\pi\mathrm{ln2} \\ $$$$\Rightarrow\int_{\mathrm{0}} ^{\infty} \:\mathrm{arcos}\left(\mathrm{1}−\mathrm{2e}^{−\mathrm{x}} \right)\mathrm{dx}\:=\mathrm{2}\pi\mathrm{ln}\left(\mathrm{2}\right) \\ $$
Answered by mathmax by abdo last updated on 24/May/20
![I =∫_0 ^∞ arcos(1−e^(−x) )dx let I(ξ) =∫_0 ^ξ arcos(1−e^(−x) )dx by parts I(ξ) =[x arcos(1−e^(−x) )]_0 ^ξ −∫_0 ^ξ x (−(e^(−x) /( (√(1−(1−e^(−x) )^2 )))))dx =ξ arcos(1−e^(−ξ) ) +∫_0 ^ξ ((xe^(−x) )/( (√(1−(1−e^(−x) )^2 )))) dx changement 1−e^(−x) =cosα give e^(−x) =1−cosα ⇒x=−ln(1−cosα) ⇒(dx/dα) =−((sinα)/(1−cosα)) ⇒ ∫_0 ^ξ ((xe^(−x) )/( (√(1−(1−e^(−x) )^2 ))))dx =∫_(π/2) ^(arcos(1−e^(−ξ) )) ln(1−cosα)×(1−cosα)×(1/(sinα))×((sinα)/(1−cosα))dα =−∫_(arcos(1−e^(−ξ) )) ^(π/2) ln(2sin^2 ((α/2)))dα =−ln(2)((π/2) −arcos(1−e^(−ξ) ))−2 ∫_0 ^(π/2) ln(sin((α/2)))dα ((α/2)=u) =−(π/2)ln(2)+ln(2)arcos(1−e^(−ξ) )−2 ∫_0 ^(π/4) ln(u)(2du) =−(π/2)ln(2) +ln(2)arcos(1−e^(−ξ) )−4∫_0 ^(π/4) ln(u)du →−(π/2)ln(2)−4 ∫_0 ^(π/4) ln(u)du rest to calculate ∫_0 ^(π/4) ln(u)du.. be continued...](https://www.tinkutara.com/question/Q95226.png)
$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\infty} \:\mathrm{arcos}\left(\mathrm{1}−\mathrm{e}^{−\mathrm{x}} \right)\mathrm{dx}\:\:\mathrm{let}\:\mathrm{I}\left(\xi\right)\:=\int_{\mathrm{0}} ^{\xi} \:\mathrm{arcos}\left(\mathrm{1}−\mathrm{e}^{−\mathrm{x}} \right)\mathrm{dx}\:\:\mathrm{by}\:\mathrm{parts} \\ $$$$\mathrm{I}\left(\xi\right)\:=\left[\mathrm{x}\:\mathrm{arcos}\left(\mathrm{1}−\mathrm{e}^{−\mathrm{x}} \right)\right]_{\mathrm{0}} ^{\xi} \:−\int_{\mathrm{0}} ^{\xi} \:\mathrm{x}\:\left(−\frac{\mathrm{e}^{−\mathrm{x}} }{\:\sqrt{\mathrm{1}−\left(\mathrm{1}−\mathrm{e}^{−\mathrm{x}} \right)^{\mathrm{2}} }}\right)\mathrm{dx} \\ $$$$=\xi\:\mathrm{arcos}\left(\mathrm{1}−\mathrm{e}^{−\xi} \right)\:+\int_{\mathrm{0}} ^{\xi} \:\:\frac{\mathrm{xe}^{−\mathrm{x}} }{\:\sqrt{\mathrm{1}−\left(\mathrm{1}−\mathrm{e}^{−\mathrm{x}} \right)^{\mathrm{2}} }}\:\mathrm{dx} \\ $$$$\mathrm{changement}\:\mathrm{1}−\mathrm{e}^{−\mathrm{x}} =\mathrm{cos}\alpha\:\:\mathrm{give}\:\mathrm{e}^{−\mathrm{x}} \:=\mathrm{1}−\mathrm{cos}\alpha\:\Rightarrow\mathrm{x}=−\mathrm{ln}\left(\mathrm{1}−\mathrm{cos}\alpha\right) \\ $$$$\Rightarrow\frac{\mathrm{dx}}{\mathrm{d}\alpha}\:=−\frac{\mathrm{sin}\alpha}{\mathrm{1}−\mathrm{cos}\alpha}\:\Rightarrow \\ $$$$\int_{\mathrm{0}} ^{\xi} \:\:\frac{\mathrm{xe}^{−\mathrm{x}} }{\:\sqrt{\mathrm{1}−\left(\mathrm{1}−\mathrm{e}^{−\mathrm{x}} \right)^{\mathrm{2}} }}\mathrm{dx}\:=\int_{\frac{\pi}{\mathrm{2}}} ^{\mathrm{arcos}\left(\mathrm{1}−\mathrm{e}^{−\xi} \right)} \:\:\mathrm{ln}\left(\mathrm{1}−\mathrm{cos}\alpha\right)×\left(\mathrm{1}−\mathrm{cos}\alpha\right)×\frac{\mathrm{1}}{\mathrm{sin}\alpha}×\frac{\mathrm{sin}\alpha}{\mathrm{1}−\mathrm{cos}\alpha}\mathrm{d}\alpha \\ $$$$=−\int_{\mathrm{arcos}\left(\mathrm{1}−\mathrm{e}^{−\xi} \right)} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{2sin}^{\mathrm{2}} \left(\frac{\alpha}{\mathrm{2}}\right)\right)\mathrm{d}\alpha \\ $$$$=−\mathrm{ln}\left(\mathrm{2}\right)\left(\frac{\pi}{\mathrm{2}}\:−\mathrm{arcos}\left(\mathrm{1}−\mathrm{e}^{−\xi} \right)\right)−\mathrm{2}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\mathrm{ln}\left(\mathrm{sin}\left(\frac{\alpha}{\mathrm{2}}\right)\right)\mathrm{d}\alpha\:\left(\frac{\alpha}{\mathrm{2}}=\mathrm{u}\right) \\ $$$$=−\frac{\pi}{\mathrm{2}}\mathrm{ln}\left(\mathrm{2}\right)+\mathrm{ln}\left(\mathrm{2}\right)\mathrm{arcos}\left(\mathrm{1}−\mathrm{e}^{−\xi} \right)−\mathrm{2}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\mathrm{ln}\left(\mathrm{u}\right)\left(\mathrm{2du}\right) \\ $$$$=−\frac{\pi}{\mathrm{2}}\mathrm{ln}\left(\mathrm{2}\right)\:+\mathrm{ln}\left(\mathrm{2}\right)\mathrm{arcos}\left(\mathrm{1}−\mathrm{e}^{−\xi} \right)−\mathrm{4}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\mathrm{ln}\left(\mathrm{u}\right)\mathrm{du} \\ $$$$\rightarrow−\frac{\pi}{\mathrm{2}}\mathrm{ln}\left(\mathrm{2}\right)−\mathrm{4}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \mathrm{ln}\left(\mathrm{u}\right)\mathrm{du}\:\:\mathrm{rest}\:\mathrm{to}\:\mathrm{calculate}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\mathrm{ln}\left(\mathrm{u}\right)\mathrm{du}.. \\ $$$$\mathrm{be}\:\mathrm{continued}… \\ $$
