Menu Close

Question-161102

Tinku Tara 0 Comments
Pin




Question Number 161102 by cortano last updated on 12/Dec/21
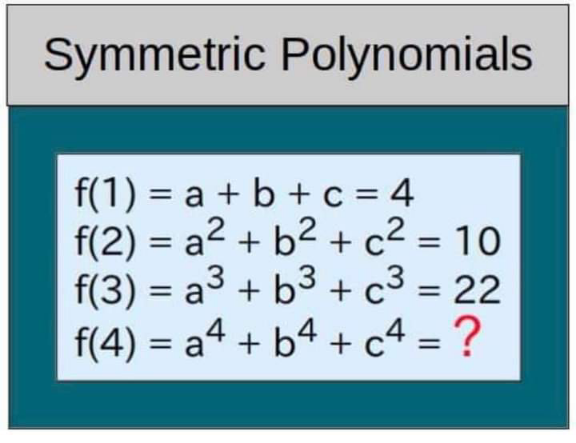
Commented by Abdulazizov last updated on 12/Dec/21
$$ \\ $$
Answered by mr W last updated on 13/Dec/21
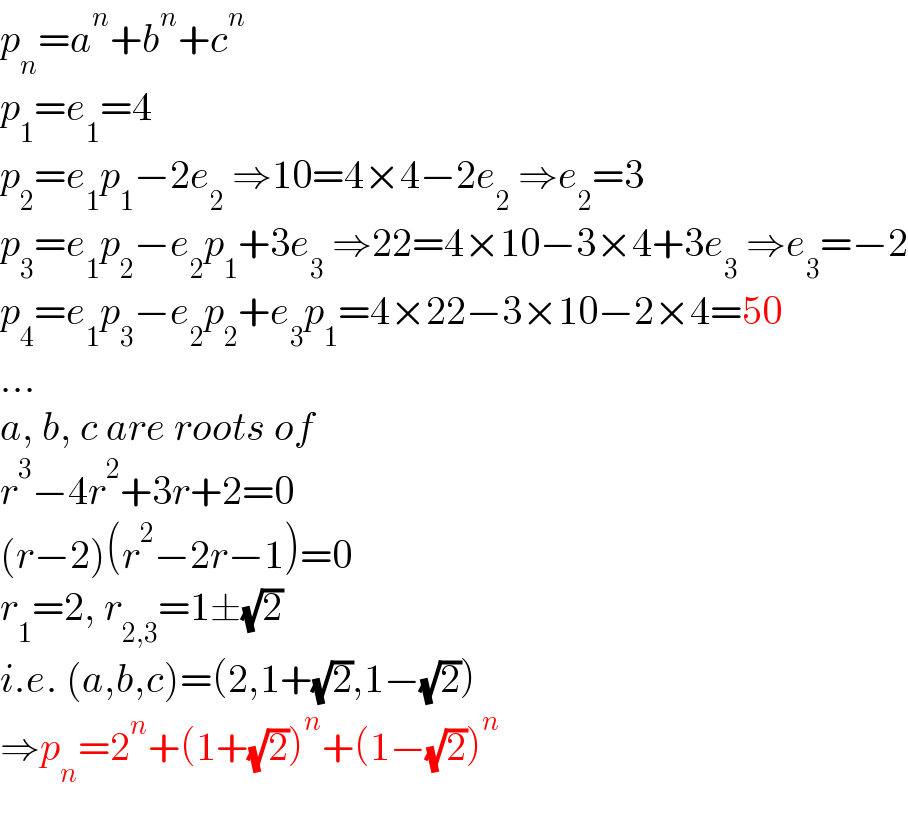
p_n =a^n +b^n +c^n p_1 =e_1 =4 p_2 =e_1 p_1 −2e_2 ⇒10=4×4−2e_2 ⇒e_2 =3 p_3 =e_1 p_2 −e_2 p_1 +3e_3 ⇒22=4×10−3×4+3e_3 ⇒e_3 =−2 p_4 =e_1 p_3 −e_2 p_2 +e_3 p_1 =4×22−3×10−2×4=50 ... a, b, c are roots of r^3 −4r^2 +3r+2=0 (r−2)(r^2 −2r−1)=0 r_1 =2, r_(2,3) =1±(√2) i.e. (a,b,c)=(2,1+(√2),1−(√2)) ⇒p_n =2^n +(1+(√2))^n +(1−(√2))^n
$${p}_{{n}} ={a}^{{n}} +{b}^{{n}} +{c}^{{n}} \\ $$$${p}_{\mathrm{1}} ={e}_{\mathrm{1}} =\mathrm{4} \\ $$$${p}_{\mathrm{2}} ={e}_{\mathrm{1}} {p}_{\mathrm{1}} −\mathrm{2}{e}_{\mathrm{2}} \:\Rightarrow\mathrm{10}=\mathrm{4}×\mathrm{4}−\mathrm{2}{e}_{\mathrm{2}} \:\Rightarrow{e}_{\mathrm{2}} =\mathrm{3} \\ $$$${p}_{\mathrm{3}} ={e}_{\mathrm{1}} {p}_{\mathrm{2}} −{e}_{\mathrm{2}} {p}_{\mathrm{1}} +\mathrm{3}{e}_{\mathrm{3}} \:\Rightarrow\mathrm{22}=\mathrm{4}×\mathrm{10}−\mathrm{3}×\mathrm{4}+\mathrm{3}{e}_{\mathrm{3}} \:\Rightarrow{e}_{\mathrm{3}} =−\mathrm{2} \\ $$$${p}_{\mathrm{4}} ={e}_{\mathrm{1}} {p}_{\mathrm{3}} −{e}_{\mathrm{2}} {p}_{\mathrm{2}} +{e}_{\mathrm{3}} {p}_{\mathrm{1}} =\mathrm{4}×\mathrm{22}−\mathrm{3}×\mathrm{10}−\mathrm{2}×\mathrm{4}=\mathrm{50} \\ $$$$… \\ $$$${a},\:{b},\:{c}\:{are}\:{roots}\:{of} \\ $$$${r}^{\mathrm{3}} −\mathrm{4}{r}^{\mathrm{2}} +\mathrm{3}{r}+\mathrm{2}=\mathrm{0} \\ $$$$\left({r}−\mathrm{2}\right)\left({r}^{\mathrm{2}} −\mathrm{2}{r}−\mathrm{1}\right)=\mathrm{0} \\ $$$${r}_{\mathrm{1}} =\mathrm{2},\:{r}_{\mathrm{2},\mathrm{3}} =\mathrm{1}\pm\sqrt{\mathrm{2}} \\ $$$${i}.{e}.\:\left({a},{b},{c}\right)=\left(\mathrm{2},\mathrm{1}+\sqrt{\mathrm{2}},\mathrm{1}−\sqrt{\mathrm{2}}\right) \\ $$$$\Rightarrow{p}_{{n}} =\mathrm{2}^{{n}} +\left(\mathrm{1}+\sqrt{\mathrm{2}}\right)^{{n}} +\left(\mathrm{1}−\sqrt{\mathrm{2}}\right)^{{n}} \\ $$
Commented by Rasheed.Sindhi last updated on 14/Dec/21
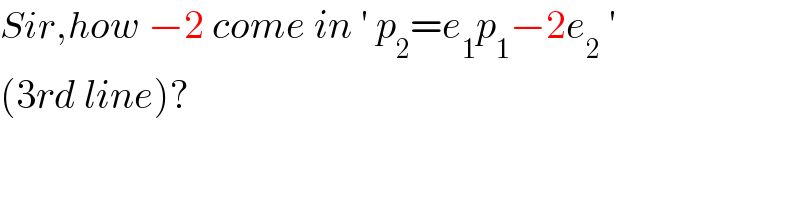
Sir,how −2 come in ′ p_2 =e_1 p_1 −2e_2 ′ (3rd line)?
$${Sir},{how}\:−\mathrm{2}\:{come}\:{in}\:'\:{p}_{\mathrm{2}} ={e}_{\mathrm{1}} {p}_{\mathrm{1}} −\mathrm{2}{e}_{\mathrm{2}} \:'\: \\ $$$$\left(\mathrm{3}{rd}\:{line}\right)? \\ $$
Commented by mr W last updated on 14/Dec/21
generally p_n =e_1 p_(n−1) −e_2 p_(n−2) +e_3 p_(n−3) −...+(−1)^n e_(n−1) p_1 +(−1)^(n+1) ne_n see Newton′s identities. certainly we can also check it: (a+b+c)^2 =a^2 +b^2 +c^2 +2(ab+bc+ca) e_1 p_1 =p_2 +2e_2 ⇒p_2 =e_1 p_1 −2e_2
$${generally} \\ $$$${p}_{{n}} ={e}_{\mathrm{1}} {p}_{{n}−\mathrm{1}} −{e}_{\mathrm{2}} {p}_{{n}−\mathrm{2}} +{e}_{\mathrm{3}} {p}_{{n}−\mathrm{3}} −…+\left(−\mathrm{1}\right)^{{n}} {e}_{{n}−\mathrm{1}} {p}_{\mathrm{1}} +\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} {ne}_{{n}} \\ $$$${see}\:{Newton}'{s}\:{identities}. \\ $$$$ \\ $$$${certainly}\:{we}\:{can}\:{also}\:{check}\:{it}: \\ $$$$\left({a}+{b}+{c}\right)^{\mathrm{2}} ={a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} +\mathrm{2}\left({ab}+{bc}+{ca}\right) \\ $$$${e}_{\mathrm{1}} {p}_{\mathrm{1}} ={p}_{\mathrm{2}} +\mathrm{2}{e}_{\mathrm{2}} \\ $$$$\Rightarrow{p}_{\mathrm{2}} ={e}_{\mathrm{1}} {p}_{\mathrm{1}} −\mathrm{2}{e}_{\mathrm{2}} \\ $$
Commented by Rasheed.Sindhi last updated on 14/Dec/21
Thanks a lOt Sir!
$$\mathbb{T}\mathrm{han}\Bbbk\mathrm{s}\:\mathrm{a}\:\mathrm{lOt}\:\mathbb{S}\mathrm{ir}! \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *