Question Number 95679 by Rio Michael last updated on 26/May/20
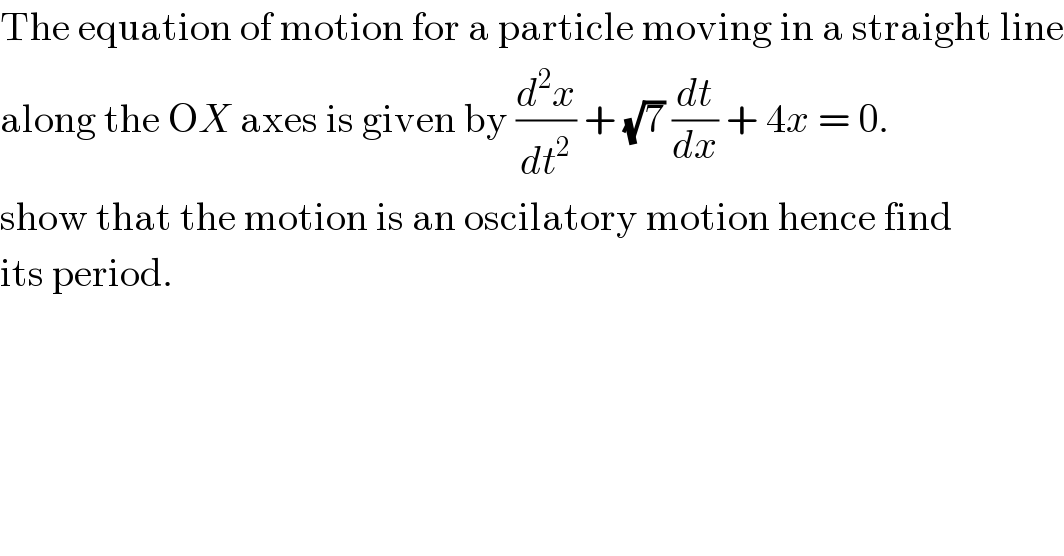
$$\mathrm{The}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{motion}\:\mathrm{for}\:\mathrm{a}\:\mathrm{particle}\:\mathrm{moving}\:\mathrm{in}\:\mathrm{a}\:\mathrm{straight}\:\mathrm{line} \\ $$$$\mathrm{along}\:\mathrm{the}\:\mathrm{O}{X}\:\mathrm{axes}\:\mathrm{is}\:\mathrm{given}\:\mathrm{by}\:\frac{{d}^{\mathrm{2}} {x}}{{dt}^{\mathrm{2}} }\:+\:\sqrt{\mathrm{7}}\:\frac{{dt}}{{dx}}\:+\:\mathrm{4}{x}\:=\:\mathrm{0}. \\ $$$$\mathrm{show}\:\mathrm{that}\:\mathrm{the}\:\mathrm{motion}\:\mathrm{is}\:\mathrm{an}\:\mathrm{oscilatory}\:\mathrm{motion}\:\mathrm{hence}\:\mathrm{find} \\ $$$$\mathrm{its}\:\mathrm{period}. \\ $$
Commented by Tony Lin last updated on 27/May/20
![λ^2 +(√7)λ+4=0 λ=((−(√7)±3i)/2) x=e^(−((√7)/2)t) (c_1 cos(3/2)t+c_2 sin(3/2)t) =e^(−((√7)/2)t) (√(c_1 ^2 +c_2 ^2 ))((c_1 /( (√(c_1 ^2 +c_2 ^2 ))))cos(3/2)t+(c_2 /( (√(c_1 ^2 +c_2 ^2 ))))sin(3/2)t) let (c_1 /( (√(c_1 ^2 +c_2 ^2 ))))=sinθ θ=sin^(−1) (c_1 /( (√(c_1 ^2 +c_2 ^2 )))) ⇒x=e^(−((√7)/2)t) (√(c_1 ^2 +c_2 ^2 ))[sin((3/2)t+θ)] (dx/dt)=−((√7)/2)e^(−((√7)/2)t ) (√(c_1 ^2 +c_2 ^2 ))(3/2)[cos((3/2)t+θ)] (d^2 x/dt^2 )=−(7/4)e^(−((√7)/2)t) (√(c_1 ^2 +c_2 ^2 ))(9/4)[sin((3/2)t+θ)] =−((63)/(16))x F=−((63m)/(16))x →oscillatory motion period T=((2π)/ω)=((2π)/(3/2))=(4/3)π but when t→∞, e^(−((√7)/2)t) →0 so the amplitude would decrease gradually](https://www.tinkutara.com/question/Q95713.png)
$$\lambda^{\mathrm{2}} +\sqrt{\mathrm{7}}\lambda+\mathrm{4}=\mathrm{0} \\ $$$$\lambda=\frac{−\sqrt{\mathrm{7}}\pm\mathrm{3}{i}}{\mathrm{2}} \\ $$$${x}={e}^{−\frac{\sqrt{\mathrm{7}}}{\mathrm{2}}{t}} \left({c}_{\mathrm{1}} {cos}\frac{\mathrm{3}}{\mathrm{2}}{t}+{c}_{\mathrm{2}} {sin}\frac{\mathrm{3}}{\mathrm{2}}{t}\right) \\ $$$$={e}^{−\frac{\sqrt{\mathrm{7}}}{\mathrm{2}}{t}} \sqrt{{c}_{\mathrm{1}} ^{\mathrm{2}} +{c}_{\mathrm{2}} ^{\mathrm{2}} }\left(\frac{{c}_{\mathrm{1}} }{\:\sqrt{{c}_{\mathrm{1}} ^{\mathrm{2}} +{c}_{\mathrm{2}} ^{\mathrm{2}} }}{cos}\frac{\mathrm{3}}{\mathrm{2}}{t}+\frac{{c}_{\mathrm{2}} }{\:\sqrt{{c}_{\mathrm{1}} ^{\mathrm{2}} +{c}_{\mathrm{2}} ^{\mathrm{2}} }}{sin}\frac{\mathrm{3}}{\mathrm{2}}{t}\right) \\ $$$${let}\:\frac{{c}_{\mathrm{1}} }{\:\sqrt{{c}_{\mathrm{1}} ^{\mathrm{2}} +{c}_{\mathrm{2}} ^{\mathrm{2}} }}={sin}\theta \\ $$$$\theta={sin}^{−\mathrm{1}} \frac{{c}_{\mathrm{1}} }{\:\sqrt{{c}_{\mathrm{1}} ^{\mathrm{2}} +{c}_{\mathrm{2}} ^{\mathrm{2}} }} \\ $$$$\Rightarrow{x}={e}^{−\frac{\sqrt{\mathrm{7}}}{\mathrm{2}}{t}} \sqrt{{c}_{\mathrm{1}} ^{\mathrm{2}} +{c}_{\mathrm{2}} ^{\mathrm{2}} }\left[{sin}\left(\frac{\mathrm{3}}{\mathrm{2}}{t}+\theta\right)\right] \\ $$$$\frac{{dx}}{{dt}}=−\frac{\sqrt{\mathrm{7}}}{\mathrm{2}}{e}^{−\frac{\sqrt{\mathrm{7}}}{\mathrm{2}}{t}\:} \sqrt{{c}_{\mathrm{1}} ^{\mathrm{2}} +{c}_{\mathrm{2}} ^{\mathrm{2}} }\frac{\mathrm{3}}{\mathrm{2}}\left[{cos}\left(\frac{\mathrm{3}}{\mathrm{2}}{t}+\theta\right)\right] \\ $$$$\frac{{d}^{\mathrm{2}} {x}}{{dt}^{\mathrm{2}} }=−\frac{\mathrm{7}}{\mathrm{4}}{e}^{−\frac{\sqrt{\mathrm{7}}}{\mathrm{2}}{t}} \sqrt{{c}_{\mathrm{1}} ^{\mathrm{2}} +{c}_{\mathrm{2}} ^{\mathrm{2}} }\frac{\mathrm{9}}{\mathrm{4}}\left[{sin}\left(\frac{\mathrm{3}}{\mathrm{2}}{t}+\theta\right)\right] \\ $$$$=−\frac{\mathrm{63}}{\mathrm{16}}{x} \\ $$$${F}=−\frac{\mathrm{63}{m}}{\mathrm{16}}{x} \\ $$$$\rightarrow{oscillatory}\:{motion} \\ $$$${period}\:{T}=\frac{\mathrm{2}\pi}{\omega}=\frac{\mathrm{2}\pi}{\frac{\mathrm{3}}{\mathrm{2}}}=\frac{\mathrm{4}}{\mathrm{3}}\pi \\ $$$${but}\:{when}\:{t}\rightarrow\infty,\:{e}^{−\frac{\sqrt{\mathrm{7}}}{\mathrm{2}}{t}} \rightarrow\mathrm{0} \\ $$$${so}\:{the}\:{amplitude}\:{would}\:{decrease}\:{gradually} \\ $$
Commented by Rio Michael last updated on 27/May/20

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{sir} \\ $$
