Question Number 2806 by prakash jain last updated on 27/Nov/15

$$\mathrm{Prove}\:\mathrm{that} \\ $$$$\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\:\frac{\mathrm{cos}\:{ix}}{{i}^{\mathrm{2}} }\:\mathrm{is}\:\mathrm{uniformly}\:\mathrm{convergent}\:\mathrm{on}\:\mathrm{real}\:\mathrm{line}. \\ $$
Answered by Yozzi last updated on 27/Nov/15
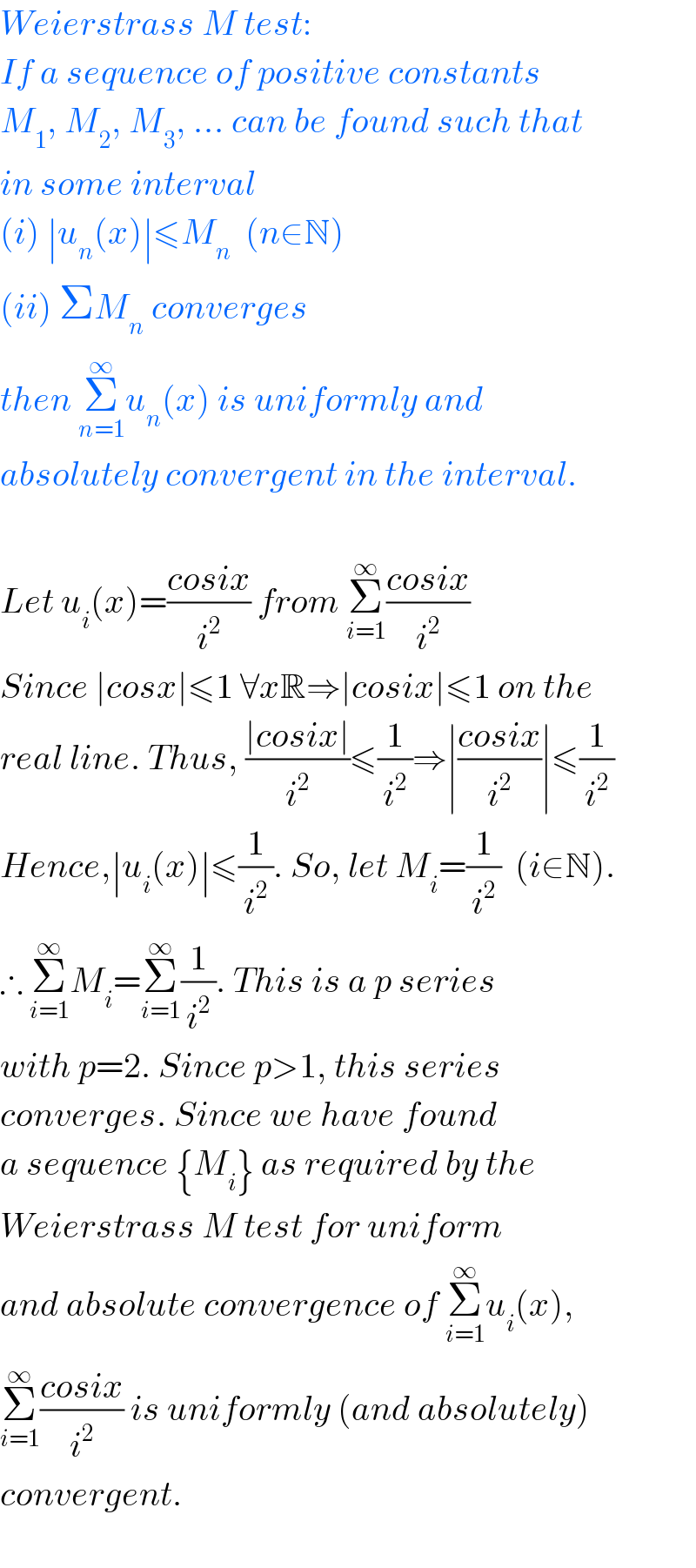
$${Weierstrass}\:{M}\:{test}: \\ $$$${If}\:{a}\:{sequence}\:{of}\:{positive}\:{constants} \\ $$$${M}_{\mathrm{1}} ,\:{M}_{\mathrm{2}} ,\:{M}_{\mathrm{3}} ,\:…\:{can}\:{be}\:{found}\:{such}\:{that} \\ $$$${in}\:{some}\:{interval} \\ $$$$\left({i}\right)\:\mid{u}_{{n}} \left({x}\right)\mid\leqslant{M}_{{n}} \:\:\left({n}\in\mathbb{N}\right) \\ $$$$\left({ii}\right)\:\Sigma{M}_{{n}} \:{converges} \\ $$$${then}\:\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}{u}_{{n}} \left({x}\right)\:{is}\:{uniformly}\:{and} \\ $$$${absolutely}\:{convergent}\:{in}\:{the}\:{interval}. \\ $$$$ \\ $$$${Let}\:{u}_{{i}} \left({x}\right)=\frac{{cosix}}{{i}^{\mathrm{2}} }\:{from}\:\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{cosix}}{{i}^{\mathrm{2}} } \\ $$$${Since}\:\mid{cosx}\mid\leqslant\mathrm{1}\:\forall{x}\mathbb{R}\Rightarrow\mid{cosix}\mid\leqslant\mathrm{1}\:{on}\:{the} \\ $$$${real}\:{line}.\:{Thus},\:\frac{\mid{cosix}\mid}{{i}^{\mathrm{2}} }\leqslant\frac{\mathrm{1}}{{i}^{\mathrm{2}} }\Rightarrow\mid\frac{{cosix}}{{i}^{\mathrm{2}} }\mid\leqslant\frac{\mathrm{1}}{{i}^{\mathrm{2}} } \\ $$$${Hence},\mid{u}_{{i}} \left({x}\right)\mid\leqslant\frac{\mathrm{1}}{{i}^{\mathrm{2}} }.\:{So},\:{let}\:{M}_{{i}} =\frac{\mathrm{1}}{{i}^{\mathrm{2}} }\:\:\left({i}\in\mathbb{N}\right). \\ $$$$\therefore\:\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}{M}_{{i}} =\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{i}^{\mathrm{2}} }.\:{This}\:{is}\:{a}\:{p}\:{series} \\ $$$${with}\:{p}=\mathrm{2}.\:{Since}\:{p}>\mathrm{1},\:{this}\:{series} \\ $$$${converges}.\:{Since}\:{we}\:{have}\:{found}\: \\ $$$${a}\:{sequence}\:\left\{{M}_{{i}} \right\}\:{as}\:{required}\:{by}\:{the}\: \\ $$$${Weierstrass}\:{M}\:{test}\:{for}\:{uniform} \\ $$$${and}\:{absolute}\:{convergence}\:{of}\:\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}{u}_{{i}} \left({x}\right), \\ $$$$\underset{{i}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{cosix}}{{i}^{\mathrm{2}} }\:{is}\:{uniformly}\:\left({and}\:{absolutely}\right) \\ $$$${convergent}. \\ $$$$ \\ $$
