Question Number 161482 by amin96 last updated on 18/Dec/21

Commented by amin96 last updated on 18/Dec/21

$${circles}\:{area}=? \\ $$
Answered by FongXD last updated on 18/Dec/21

Commented by Tawa11 last updated on 18/Dec/21

$$\mathrm{Great}\:\mathrm{sir}. \\ $$
Answered by FongXD last updated on 18/Dec/21
Commented by amin96 last updated on 18/Dec/21

$${great}\:{sir}\:{weldone} \\ $$
Commented by mr W last updated on 18/Dec/21

$${nice}\:{job}! \\ $$
Answered by mr W last updated on 18/Dec/21

Commented by mr W last updated on 18/Dec/21
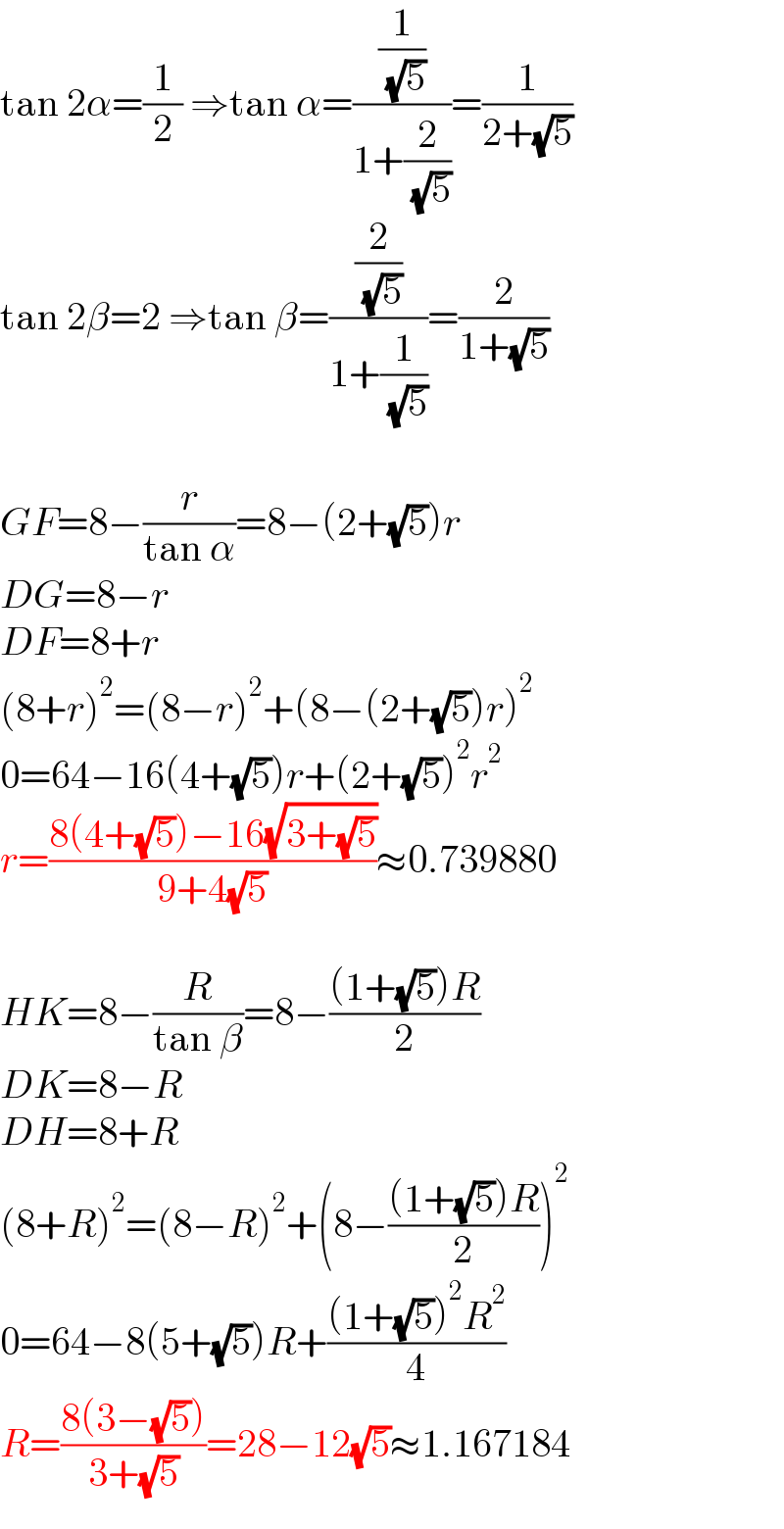
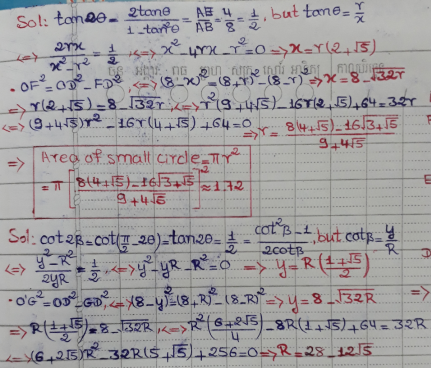
$$\mathrm{tan}\:\mathrm{2}\alpha=\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow\mathrm{tan}\:\alpha=\frac{\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}}{\mathrm{1}+\frac{\mathrm{2}}{\:\sqrt{\mathrm{5}}}}=\frac{\mathrm{1}}{\mathrm{2}+\sqrt{\mathrm{5}}} \\ $$$$\mathrm{tan}\:\mathrm{2}\beta=\mathrm{2}\:\Rightarrow\mathrm{tan}\:\beta=\frac{\frac{\mathrm{2}}{\:\sqrt{\mathrm{5}}}}{\mathrm{1}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{5}}}}=\frac{\mathrm{2}}{\mathrm{1}+\sqrt{\mathrm{5}}} \\ $$$$ \\ $$$${GF}=\mathrm{8}−\frac{{r}}{\mathrm{tan}\:\alpha}=\mathrm{8}−\left(\mathrm{2}+\sqrt{\mathrm{5}}\right){r} \\ $$$${DG}=\mathrm{8}−{r} \\ $$$${DF}=\mathrm{8}+{r} \\ $$$$\left(\mathrm{8}+{r}\right)^{\mathrm{2}} =\left(\mathrm{8}−{r}\right)^{\mathrm{2}} +\left(\mathrm{8}−\left(\mathrm{2}+\sqrt{\mathrm{5}}\right){r}\right)^{\mathrm{2}} \\ $$$$\mathrm{0}=\mathrm{64}−\mathrm{16}\left(\mathrm{4}+\sqrt{\mathrm{5}}\right){r}+\left(\mathrm{2}+\sqrt{\mathrm{5}}\right)^{\mathrm{2}} {r}^{\mathrm{2}} \\ $$$${r}=\frac{\mathrm{8}\left(\mathrm{4}+\sqrt{\mathrm{5}}\right)−\mathrm{16}\sqrt{\mathrm{3}+\sqrt{\mathrm{5}}}}{\mathrm{9}+\mathrm{4}\sqrt{\mathrm{5}}}\approx\mathrm{0}.\mathrm{739880} \\ $$$$ \\ $$$${HK}=\mathrm{8}−\frac{{R}}{\mathrm{tan}\:\beta}=\mathrm{8}−\frac{\left(\mathrm{1}+\sqrt{\mathrm{5}}\right){R}}{\mathrm{2}} \\ $$$${DK}=\mathrm{8}−{R} \\ $$$${DH}=\mathrm{8}+{R} \\ $$$$\left(\mathrm{8}+{R}\right)^{\mathrm{2}} =\left(\mathrm{8}−{R}\right)^{\mathrm{2}} +\left(\mathrm{8}−\frac{\left(\mathrm{1}+\sqrt{\mathrm{5}}\right){R}}{\mathrm{2}}\right)^{\mathrm{2}} \\ $$$$\mathrm{0}=\mathrm{64}−\mathrm{8}\left(\mathrm{5}+\sqrt{\mathrm{5}}\right){R}+\frac{\left(\mathrm{1}+\sqrt{\mathrm{5}}\right)^{\mathrm{2}} {R}^{\mathrm{2}} }{\mathrm{4}} \\ $$$${R}=\frac{\mathrm{8}\left(\mathrm{3}−\sqrt{\mathrm{5}}\right)}{\mathrm{3}+\sqrt{\mathrm{5}}}=\mathrm{28}−\mathrm{12}\sqrt{\mathrm{5}}\approx\mathrm{1}.\mathrm{167184} \\ $$
Commented by Ar Brandon last updated on 19/Dec/21
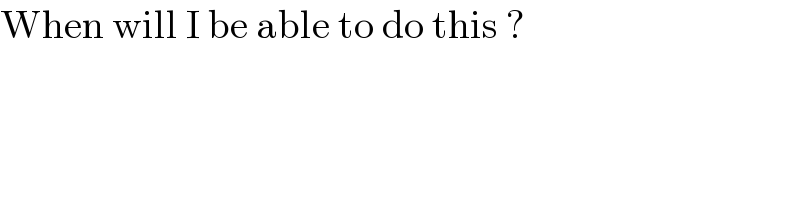
$$\mathrm{When}\:\mathrm{will}\:\mathrm{I}\:\mathrm{be}\:\mathrm{able}\:\mathrm{to}\:\mathrm{do}\:\mathrm{this}\:? \\ $$
Commented by mr W last updated on 19/Dec/21
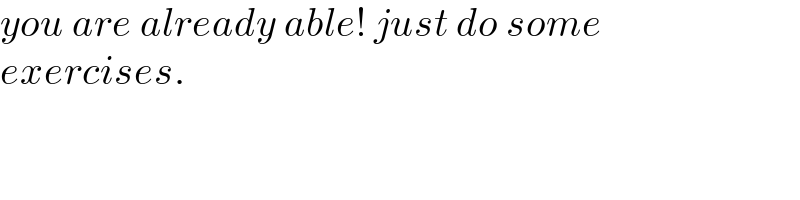
$${you}\:{are}\:{already}\:{able}!\:{just}\:{do}\:{some} \\ $$$${exercises}. \\ $$
Commented by peter frank last updated on 19/Dec/21
$$\mathrm{may}\:\mathrm{be}\:\mathrm{we}\:\mathrm{should}\:\mathrm{ask}\:\mathrm{him}\:\mathrm{how} \\ $$$$\mathrm{he}\:\mathrm{managed}? \\ $$
Commented by peter frank last updated on 19/Dec/21

$$\mathrm{To}\:\mathrm{mrW}.\mathrm{we}\:\mathrm{thank}\:\mathrm{you}\:\mathrm{very}\:\mathrm{much} \\ $$$$\mathrm{for}\:\mathrm{being}\:\mathrm{here}\:\mathrm{in}\:\mathrm{this}\:\mathrm{forum} \\ $$$$\mathrm{we}\:\mathrm{learn}\:\mathrm{alot}\:\mathrm{from}\:\mathrm{you}\left(\mathrm{Physics},\mathrm{Maths}\right) \\ $$
Commented by mr W last updated on 19/Dec/21
$${thanks}\:{for}\:{feedback}!\:{if}\:{i}\:{could}\:{help}\: \\ $$$${you}\:{somehow},\:{i}\:{did}\:{with}\:{pleasure}. \\ $$
Commented by Ar Brandon last updated on 19/Dec/21

$$\mathrm{Alright}\:\mathrm{Sir}.\:\mathrm{But}\:\mathrm{I}\:\mathrm{need}\:\mathrm{some}\:\mathrm{ressources}. \\ $$$$\mathrm{I}\:\mathrm{have}\:\mathrm{none}.\:\mathrm{Documents}\:\mathrm{on}\:\mathrm{this}\:\mathrm{topic}. \\ $$
Commented by mr W last updated on 20/Dec/21
$${try}\:{to}\:{find}\:{the}\:{radii}\:{of}\:{the}\:{three}\:{new} \\ $$$${circles}\:{if}\:{you}\:{want}: \\ $$
Commented by mr W last updated on 20/Dec/21

Commented by Ar Brandon last updated on 20/Dec/21

$$\mathrm{Sir},\:\mathrm{I}\:\mathrm{notice}\:\mathrm{the}\:\mathrm{smallest}\:\mathrm{radius}\:\mathrm{is}\:\frac{\mathrm{R}}{\mathrm{2}} \\ $$$$\mathrm{But}\:\mathrm{have}\:\mathrm{no}\:\mathrm{idea}\:\mathrm{on}\:\mathrm{how}\:\mathrm{to}\:\mathrm{solve}\:\mathrm{the}\:\mathrm{others}. \\ $$
Commented by Ar Brandon last updated on 20/Dec/21

Commented by Ar Brandon last updated on 20/Dec/21

$$\mathrm{Here}\:\mathrm{is}\:\mathrm{my}\:\mathrm{sketch}.\:\mathrm{I}\:\mathrm{don}'\mathrm{t}\:\mathrm{know}\:\mathrm{what}\:\mathrm{to}\:\mathrm{do}\:\mathrm{next}. \\ $$
Commented by mr W last updated on 20/Dec/21

Commented by Ar Brandon last updated on 21/Dec/21
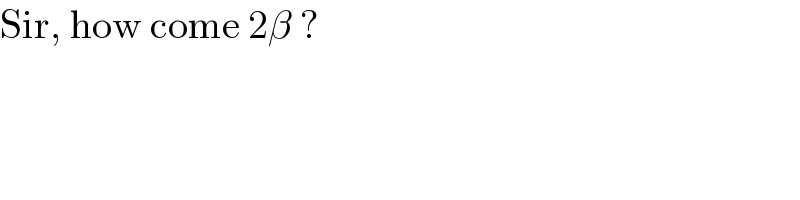
$$\mathrm{Sir},\:\mathrm{how}\:\mathrm{come}\:\mathrm{2}\beta\:? \\ $$
Commented by mr W last updated on 21/Dec/21

Commented by mr W last updated on 21/Dec/21
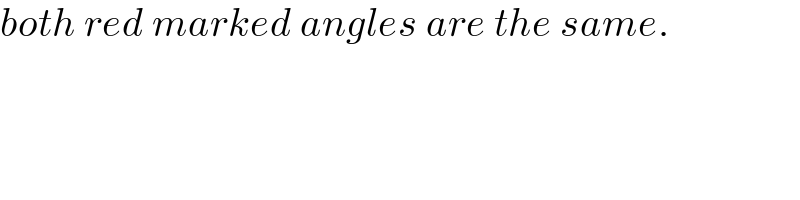
$${both}\:{red}\:{marked}\:{angles}\:{are}\:{the}\:{same}. \\ $$
Commented by Ar Brandon last updated on 21/Dec/21
OK Sir. I see! Thanks ��
