Question Number 30494 by abdo imad last updated on 22/Feb/18
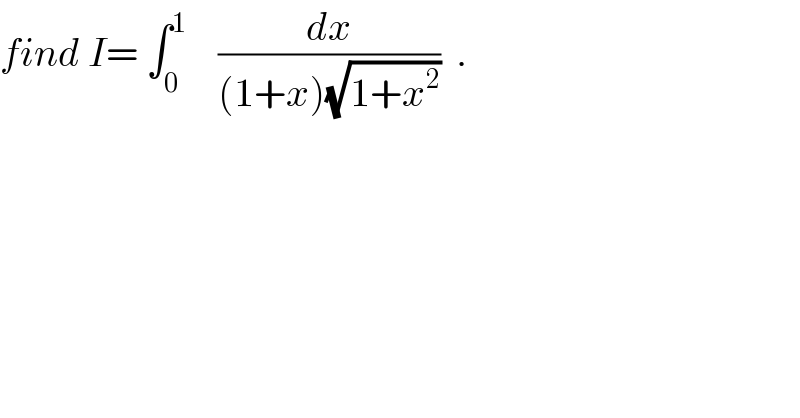
$${find}\:{I}=\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\frac{{dx}}{\left(\mathrm{1}+{x}\right)\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }}\:\:. \\ $$
Answered by sma3l2996 last updated on 24/Feb/18
![x=sinh(t)⇒dx=cosh(t)dt=(√(1+sinh^2 t))dt dt=(dx/( (√(1+x^2 )))) I=∫_0 ^(ln((√2)+1)) (dt/(1+sinh(t)))=∫((e^t dt)/(e^(2t) +e^t −1)) u=e^t ⇒du=e^t dt I=∫_1 ^((√2)+1) (du/(u^2 +u−1))=∫(du/((u+(1/2))^2 −(5/4))) I=(4/5)∫_1 ^((√2)+1) (du/(((2/( (√5)))×((2u+1)/2))^2 −1))=(4/5)∫(du/((((2u+1)/( (√5))))^2 −1)) y=((2u+1)/( (√5)))⇒du=((√5)/2)dy I=((2(√5))/5)∫_(3/(√5)) ^((2(√2)+3)/(√5)) (dy/(y^2 −1))=((2(√5))/5)∫(dy/((y+1)(y−1))) I=((√5)/5)∫_(3/(√5)) ^((2(√2)+3)/(√5)) ((1/(y−1))−(1/(y+1)))dy=((√5)/5)[ln∣((y−1)/(y+1))∣]_(3/(√5)) ^((2(√2)+3)/(√5))](https://www.tinkutara.com/question/Q30676.png)
$${x}={sinh}\left({t}\right)\Rightarrow{dx}={cosh}\left({t}\right){dt}=\sqrt{\mathrm{1}+{sinh}^{\mathrm{2}} {t}}{dt} \\ $$$${dt}=\frac{{dx}}{\:\sqrt{\mathrm{1}+{x}^{\mathrm{2}} }} \\ $$$${I}=\int_{\mathrm{0}} ^{{ln}\left(\sqrt{\mathrm{2}}+\mathrm{1}\right)} \frac{{dt}}{\mathrm{1}+{sinh}\left({t}\right)}=\int\frac{{e}^{{t}} {dt}}{{e}^{\mathrm{2}{t}} +{e}^{{t}} −\mathrm{1}} \\ $$$${u}={e}^{{t}} \Rightarrow{du}={e}^{{t}} {dt} \\ $$$${I}=\int_{\mathrm{1}} ^{\sqrt{\mathrm{2}}+\mathrm{1}} \frac{{du}}{{u}^{\mathrm{2}} +{u}−\mathrm{1}}=\int\frac{{du}}{\left({u}+\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} −\frac{\mathrm{5}}{\mathrm{4}}} \\ $$$${I}=\frac{\mathrm{4}}{\mathrm{5}}\int_{\mathrm{1}} ^{\sqrt{\mathrm{2}}+\mathrm{1}} \frac{{du}}{\left(\frac{\mathrm{2}}{\:\sqrt{\mathrm{5}}}×\frac{\mathrm{2}{u}+\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{2}} −\mathrm{1}}=\frac{\mathrm{4}}{\mathrm{5}}\int\frac{{du}}{\left(\frac{\mathrm{2}{u}+\mathrm{1}}{\:\sqrt{\mathrm{5}}}\right)^{\mathrm{2}} −\mathrm{1}} \\ $$$${y}=\frac{\mathrm{2}{u}+\mathrm{1}}{\:\sqrt{\mathrm{5}}}\Rightarrow{du}=\frac{\sqrt{\mathrm{5}}}{\mathrm{2}}{dy} \\ $$$${I}=\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{5}}\int_{\mathrm{3}/\sqrt{\mathrm{5}}} ^{\left(\mathrm{2}\sqrt{\mathrm{2}}+\mathrm{3}\right)/\sqrt{\mathrm{5}}} \frac{{dy}}{{y}^{\mathrm{2}} −\mathrm{1}}=\frac{\mathrm{2}\sqrt{\mathrm{5}}}{\mathrm{5}}\int\frac{{dy}}{\left({y}+\mathrm{1}\right)\left({y}−\mathrm{1}\right)} \\ $$$${I}=\frac{\sqrt{\mathrm{5}}}{\mathrm{5}}\int_{\mathrm{3}/\sqrt{\mathrm{5}}} ^{\left(\mathrm{2}\sqrt{\mathrm{2}}+\mathrm{3}\right)/\sqrt{\mathrm{5}}} \left(\frac{\mathrm{1}}{{y}−\mathrm{1}}−\frac{\mathrm{1}}{{y}+\mathrm{1}}\right){dy}=\frac{\sqrt{\mathrm{5}}}{\mathrm{5}}\left[{ln}\mid\frac{{y}−\mathrm{1}}{{y}+\mathrm{1}}\mid\right]_{\mathrm{3}/\sqrt{\mathrm{5}}} ^{\left(\mathrm{2}\sqrt{\mathrm{2}}+\mathrm{3}\right)/\sqrt{\mathrm{5}}} \\ $$
