Menu Close

let-p-x-x-2n-2cos-x-n-1-1-find-roots-lf-p-x-2-factorize-p-x-inside-C-x-3-factorize-p-x-inside-R-x-

Tinku Tara 0 Comments
Pin




Question Number 30592 by abdo imad last updated on 23/Feb/18
let p(x)=x^(2n) −2cosα x^n +1 1) find roots lf p(x) 2)factorize p(x) inside C[x] 3)factorize p(x) inside R[x].
$${let}\:{p}\left({x}\right)={x}^{\mathrm{2}{n}} \:−\mathrm{2}{cos}\alpha\:{x}^{{n}} \:+\mathrm{1} \\ $$$$\left.\mathrm{1}\right)\:{find}\:{roots}\:{lf}\:{p}\left({x}\right) \\ $$$$\left.\mathrm{2}\right){factorize}\:{p}\left({x}\right)\:{inside}\:{C}\left[{x}\right] \\ $$$$\left.\mathrm{3}\right){factorize}\:{p}\left({x}\right)\:{inside}\:{R}\left[{x}\right]. \\ $$
Answered by sma3l2996 last updated on 23/Feb/18
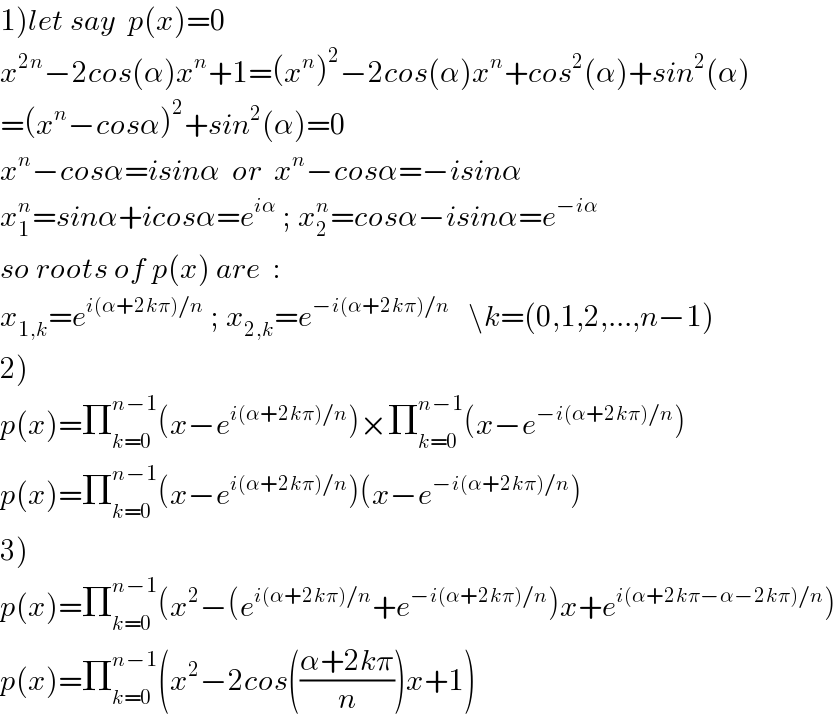
1)let say p(x)=0 x^(2n) −2cos(α)x^n +1=(x^n )^2 −2cos(α)x^n +cos^2 (α)+sin^2 (α) =(x^n −cosα)^2 +sin^2 (α)=0 x^n −cosα=isinα or x^n −cosα=−isinα x_1 ^n =sinα+icosα=e^(iα) ; x_2 ^n =cosα−isinα=e^(−iα) so roots of p(x) are : x_(1,k) =e^(i(α+2kπ)/n) ; x_(2,k) =e^(−i(α+2kπ)/n) \k=(0,1,2,...,n−1) 2) p(x)=Π_(k=0) ^(n−1) (x−e^(i(α+2kπ)/n) )×Π_(k=0) ^(n−1) (x−e^(−i(α+2kπ)/n) ) p(x)=Π_(k=0) ^(n−1) (x−e^(i(α+2kπ)/n) )(x−e^(−i(α+2kπ)/n) ) 3) p(x)=Π_(k=0) ^(n−1) (x^2 −(e^(i(α+2kπ)/n) +e^(−i(α+2kπ)/n) )x+e^(i(α+2kπ−α−2kπ)/n) ) p(x)=Π_(k=0) ^(n−1) (x^2 −2cos(((α+2kπ)/n))x+1)
$$\left.\mathrm{1}\right){let}\:{say}\:\:{p}\left({x}\right)=\mathrm{0} \\ $$$${x}^{\mathrm{2}{n}} −\mathrm{2}{cos}\left(\alpha\right){x}^{{n}} +\mathrm{1}=\left({x}^{{n}} \right)^{\mathrm{2}} −\mathrm{2}{cos}\left(\alpha\right){x}^{{n}} +{cos}^{\mathrm{2}} \left(\alpha\right)+{sin}^{\mathrm{2}} \left(\alpha\right) \\ $$$$=\left({x}^{{n}} −{cos}\alpha\right)^{\mathrm{2}} +{sin}^{\mathrm{2}} \left(\alpha\right)=\mathrm{0} \\ $$$${x}^{{n}} −{cos}\alpha={isin}\alpha\:\:{or}\:\:{x}^{{n}} −{cos}\alpha=−{isin}\alpha \\ $$$${x}_{\mathrm{1}} ^{{n}} ={sin}\alpha+{icos}\alpha={e}^{{i}\alpha} \:;\:{x}_{\mathrm{2}} ^{{n}} ={cos}\alpha−{isin}\alpha={e}^{−{i}\alpha} \\ $$$${so}\:{roots}\:{of}\:{p}\left({x}\right)\:{are}\:\:: \\ $$$${x}_{\mathrm{1},{k}} ={e}^{{i}\left(\alpha+\mathrm{2}{k}\pi\right)/{n}} \:;\:{x}_{\mathrm{2},{k}} ={e}^{−{i}\left(\alpha+\mathrm{2}{k}\pi\right)/{n}} \:\:\:\backslash{k}=\left(\mathrm{0},\mathrm{1},\mathrm{2},…,{n}−\mathrm{1}\right) \\ $$$$\left.\mathrm{2}\right) \\ $$$${p}\left({x}\right)=\prod_{{k}=\mathrm{0}} ^{{n}−\mathrm{1}} \left({x}−{e}^{{i}\left(\alpha+\mathrm{2}{k}\pi\right)/{n}} \right)×\prod_{{k}=\mathrm{0}} ^{{n}−\mathrm{1}} \left({x}−{e}^{−{i}\left(\alpha+\mathrm{2}{k}\pi\right)/{n}} \right) \\ $$$${p}\left({x}\right)=\prod_{{k}=\mathrm{0}} ^{{n}−\mathrm{1}} \left({x}−{e}^{{i}\left(\alpha+\mathrm{2}{k}\pi\right)/{n}} \right)\left({x}−{e}^{−{i}\left(\alpha+\mathrm{2}{k}\pi\right)/{n}} \right) \\ $$$$\left.\mathrm{3}\right) \\ $$$${p}\left({x}\right)=\prod_{{k}=\mathrm{0}} ^{{n}−\mathrm{1}} \left({x}^{\mathrm{2}} −\left({e}^{{i}\left(\alpha+\mathrm{2}{k}\pi\right)/{n}} +{e}^{−{i}\left(\alpha+\mathrm{2}{k}\pi\right)/{n}} \right){x}+{e}^{{i}\left(\alpha+\mathrm{2}{k}\pi−\alpha−\mathrm{2}{k}\pi\right)/{n}} \right) \\ $$$${p}\left({x}\right)=\prod_{{k}=\mathrm{0}} ^{{n}−\mathrm{1}} \left({x}^{\mathrm{2}} −\mathrm{2}{cos}\left(\frac{\alpha+\mathrm{2}{k}\pi}{{n}}\right){x}+\mathrm{1}\right) \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *