Question Number 134002 by Dwaipayan Shikari last updated on 26/Feb/21

$${What}\:{will}\:{be}\:{the}\:{minimum}\:{area}\:{of}\:{a}\:{heptagon}\:{inscribed}\:{in} \\ $$$${an}\:{unit}\:{square}? \\ $$
Commented by Dwaipayan Shikari last updated on 26/Feb/21

Answered by mr W last updated on 26/Feb/21
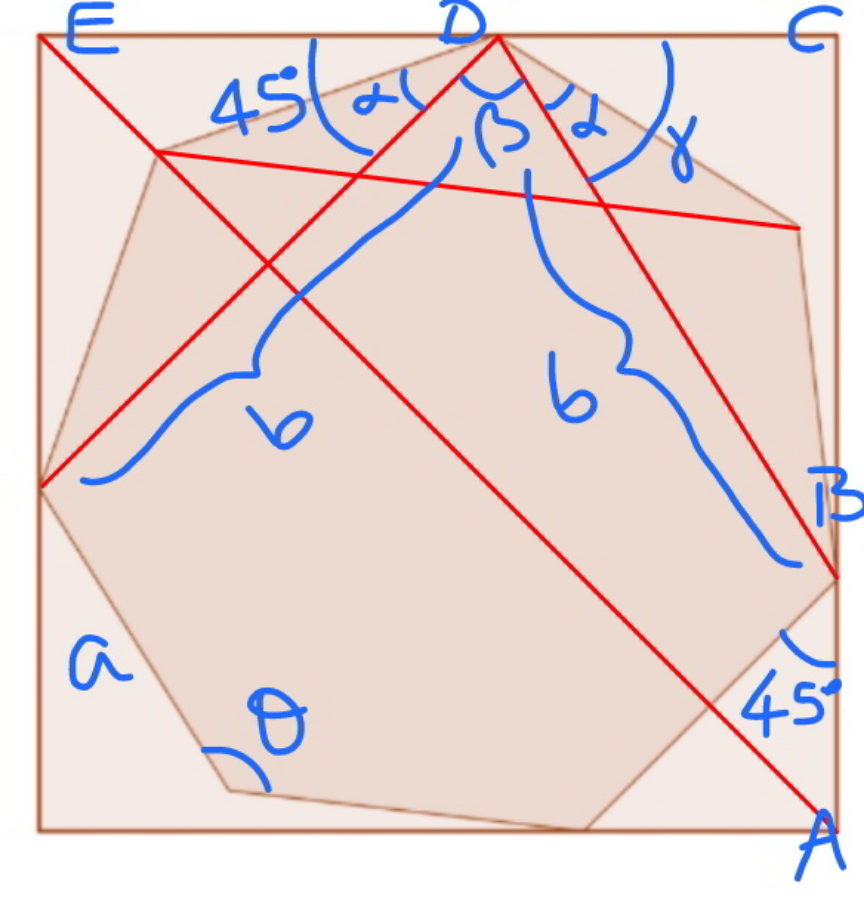
Commented by mr W last updated on 26/Feb/21

$${side}\:{length}\:{of}\:{heptagon}\:=\:{a} \\ $$$$\theta=\frac{\left(\mathrm{7}−\mathrm{2}\right)×\mathrm{180}°}{\mathrm{7}}=\frac{\mathrm{900}°}{\mathrm{7}} \\ $$$$\alpha=\mathrm{90}°−\frac{\theta}{\mathrm{2}}=\frac{\mathrm{180}°}{\mathrm{7}} \\ $$$$\beta=\theta−\mathrm{2}\alpha=\frac{\mathrm{540}°}{\mathrm{7}} \\ $$$$\gamma=\mathrm{180}°−\mathrm{45}°−\beta=\frac{\mathrm{405}°}{\mathrm{7}} \\ $$$${DB}={b}=\mathrm{2}{a}\:\mathrm{cos}\:\alpha=\mathrm{2}{a}\:\mathrm{cos}\:\frac{\mathrm{180}°}{\mathrm{7}} \\ $$$${AB}=\frac{{a}}{\:\sqrt{\mathrm{2}}} \\ $$$${BC}={b}\:\mathrm{sin}\:\gamma=\mathrm{2}{a}\:\mathrm{cos}\:\frac{\mathrm{180}°}{\mathrm{7}}\:\mathrm{sin}\:\frac{\mathrm{405}°}{\mathrm{7}} \\ $$$${AC}=\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}+\mathrm{2}\:\mathrm{cos}\:\frac{\mathrm{180}°}{\mathrm{7}}\:\mathrm{sin}\:\frac{\mathrm{405}°}{\mathrm{7}}\right){a}=\mathrm{1} \\ $$$$\Rightarrow{a}=\frac{\mathrm{1}}{\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}+\mathrm{2}\:\mathrm{cos}\:\frac{\mathrm{180}°}{\mathrm{7}}\:\mathrm{sin}\:\frac{\mathrm{405}°}{\mathrm{7}}} \\ $$$${area}\:{of}\:{heptagon} \\ $$$${A}=\frac{\mathrm{7}{a}^{\mathrm{2}} }{\mathrm{4}\:\mathrm{tan}\:\frac{\mathrm{180}°}{\mathrm{7}}} \\ $$$$=\frac{\mathrm{7}}{\mathrm{4}\:\mathrm{tan}\:\frac{\mathrm{180}°}{\mathrm{7}}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}+\mathrm{2}\:\mathrm{cos}\:\frac{\mathrm{180}°}{\mathrm{7}}\:\mathrm{sin}\:\frac{\mathrm{405}°}{\mathrm{7}}\right)^{\mathrm{2}} } \\ $$$$\approx\mathrm{0}.\mathrm{728}\:\mathrm{878}\:\mathrm{175} \\ $$$$ \\ $$$${check}: \\ $$$${ED}=\frac{{b}}{\:\sqrt{\mathrm{2}}} \\ $$$${DC}={b}\:\mathrm{cos}\:\gamma \\ $$$${EC}={b}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}+\mathrm{cos}\:\gamma\right)=\mathrm{2}{a}\:\mathrm{cos}\:\frac{\mathrm{180}°}{\mathrm{7}}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}+\mathrm{cos}\:\frac{\mathrm{405}°}{\mathrm{7}}\right) \\ $$$$=\mathrm{2}×\frac{\mathrm{1}}{\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}+\mathrm{2}\:\mathrm{cos}\:\frac{\mathrm{180}°}{\mathrm{7}}\:\mathrm{sin}\:\frac{\mathrm{405}°}{\mathrm{7}}}×\mathrm{cos}\:\frac{\mathrm{180}°}{\mathrm{7}}\left(\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}}}+\mathrm{cos}\:\frac{\mathrm{405}°}{\mathrm{7}}\right) \\ $$$$=\mathrm{1} \\ $$
Commented by Dwaipayan Shikari last updated on 26/Feb/21

$${Great}\:{sir}! \\ $$
