Menu Close

Prove-that-ln-sec-x-tan-x-tanh-1-sin-x-

Tinku Tara 0 Comments
Pin




Question Number 96729 by qwertasdf last updated on 04/Jun/20
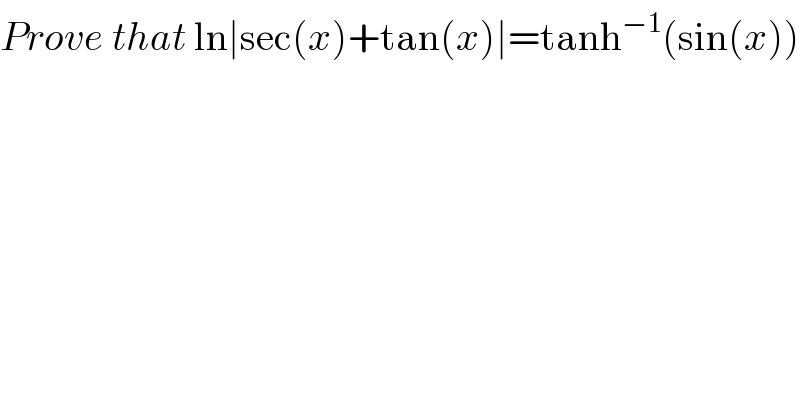
Prove that ln∣sec(x)+tan(x)∣=tanh^(−1) (sin(x))
$${Prove}\:{that}\:\mathrm{ln}\mid\mathrm{sec}\left({x}\right)+\mathrm{tan}\left({x}\right)\mid=\mathrm{tanh}^{−\mathrm{1}} \left(\mathrm{sin}\left({x}\right)\right) \\ $$
Commented by prakash jain last updated on 04/Jun/20
tanh^(−1) (x)=ln (((1+x)/(1−x)))^(1/2) tanh^(−1) (sin (x))=ln ((1+sin x)/(1−sin x)) When sin x≠1 ln( ((1+sin x)/(1−sin x))×((1+sin x)/(1+sin x)))^(1/2) ln (((1+2sin x+sin^2 x)/(1−sin^2 x)))^(1/2) =ln ((1/(cos^2 x))+2tan xsec x+tan^2 x)^(1/2) =ln ((sec x+tan x)^2 )^(1/2) =ln ∣sec x+tan x∣ equality only valid when sin x≠1
$$\mathrm{tanh}^{−\mathrm{1}} \left({x}\right)=\mathrm{ln}\:\left(\frac{\mathrm{1}+{x}}{\mathrm{1}−{x}}\right)^{\mathrm{1}/\mathrm{2}} \\ $$$$\mathrm{tanh}^{−\mathrm{1}} \left(\mathrm{sin}\:\left({x}\right)\right)=\mathrm{ln}\:\frac{\mathrm{1}+\mathrm{sin}\:{x}}{\mathrm{1}−\mathrm{sin}\:{x}} \\ $$$$\mathrm{When}\:\mathrm{sin}\:{x}\neq\mathrm{1} \\ $$$$\mathrm{ln}\left(\:\frac{\mathrm{1}+\mathrm{sin}\:{x}}{\mathrm{1}−\mathrm{sin}\:{x}}×\frac{\mathrm{1}+\mathrm{sin}\:{x}}{\mathrm{1}+\mathrm{sin}\:{x}}\right)^{\mathrm{1}/\mathrm{2}} \\ $$$$\mathrm{ln}\:\left(\frac{\mathrm{1}+\mathrm{2sin}\:{x}+\mathrm{sin}^{\mathrm{2}} {x}}{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} {x}}\right)^{\mathrm{1}/\mathrm{2}} \\ $$$$=\mathrm{ln}\:\left(\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} {x}}+\mathrm{2tan}\:{x}\mathrm{sec}\:{x}+\mathrm{tan}^{\mathrm{2}} {x}\right)^{\mathrm{1}/\mathrm{2}} \\ $$$$=\mathrm{ln}\:\left(\left(\mathrm{sec}\:{x}+\mathrm{tan}\:{x}\right)^{\mathrm{2}} \right)^{\mathrm{1}/\mathrm{2}} \\ $$$$=\mathrm{ln}\:\mid\mathrm{sec}\:{x}+\mathrm{tan}\:{x}\mid \\ $$$$\mathrm{equality}\:\mathrm{only}\:\mathrm{valid}\:\mathrm{when}\:\mathrm{sin}\:{x}\neq\mathrm{1} \\ $$
Answered by Ar Brandon last updated on 04/Jun/20
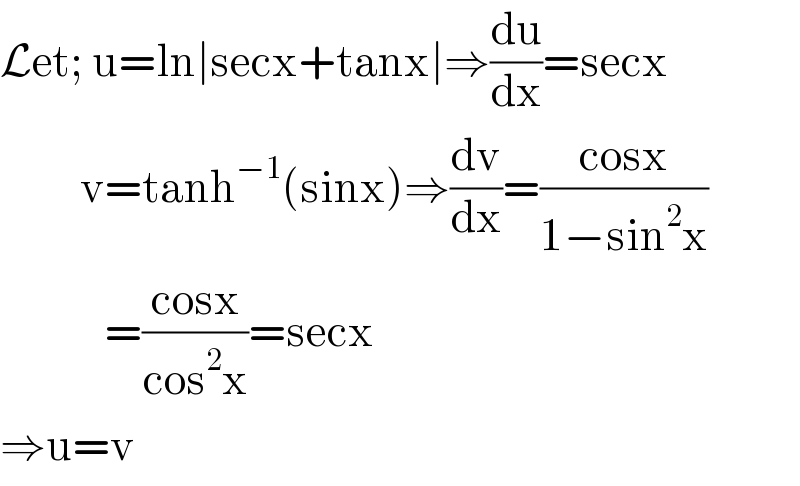
Let; u=ln∣secx+tanx∣⇒(du/dx)=secx v=tanh^(−1) (sinx)⇒(dv/dx)=((cosx)/(1−sin^2 x)) =((cosx)/(cos^2 x))=secx ⇒u=v
$$\mathcal{L}\mathrm{et};\:\mathrm{u}=\mathrm{ln}\mid\mathrm{secx}+\mathrm{tanx}\mid\Rightarrow\frac{\mathrm{du}}{\mathrm{dx}}=\mathrm{secx} \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{v}=\mathrm{tanh}^{−\mathrm{1}} \left(\mathrm{sinx}\right)\Rightarrow\frac{\mathrm{dv}}{\mathrm{dx}}=\frac{\mathrm{cosx}}{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \mathrm{x}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{cosx}}{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}=\mathrm{secx} \\ $$$$\Rightarrow\mathrm{u}=\mathrm{v} \\ $$
Commented by Ar Brandon last updated on 04/Jun/20
But in this case the constants are all 0 I feel that makes a difference. What do you think Sir?
$$\mathrm{But}\:\mathrm{in}\:\mathrm{this}\:\mathrm{case}\:\mathrm{the}\:\mathrm{constants}\:\mathrm{are}\:\mathrm{all}\:\mathrm{0} \\ $$$$\mathcal{I}\:\mathrm{feel}\:\mathrm{that}\:\mathrm{makes}\:\mathrm{a}\:\mathrm{difference}.\:\mathrm{What}\:\mathrm{do} \\ $$$$\mathrm{you}\:\mathrm{think}\:\mathrm{Sir}? \\ $$
Commented by prakash jain last updated on 04/Jun/20
(du/dx)=(dv/dx)⇏u=v ex: u=x^2 +c v=x^2
$$\frac{{du}}{{dx}}=\frac{{dv}}{{dx}}\nRightarrow{u}={v} \\ $$$${ex}: \\ $$$${u}={x}^{\mathrm{2}} +{c} \\ $$$${v}={x}^{\mathrm{2}} \\ $$
Commented by prakash jain last updated on 04/Jun/20
But then you will need a formal proof that there is no constant term. For example function like cosx,e^x have terms independent of x.
$$\mathrm{But}\:\mathrm{then}\:\mathrm{you}\:\mathrm{will}\:\mathrm{need}\:\mathrm{a}\:\mathrm{formal} \\ $$$$\mathrm{proof}\:\mathrm{that}\:\mathrm{there}\:\mathrm{is}\:\mathrm{no}\:\mathrm{constant}\:\mathrm{term}. \\ $$$$\mathrm{For}\:\mathrm{example}\:\mathrm{function}\:\mathrm{like}\:\mathrm{cos}{x},{e}^{{x}} \\ $$$$\mathrm{have}\:\mathrm{terms}\:\mathrm{independent}\:\mathrm{of}\:{x}. \\ $$
Commented by prakash jain last updated on 04/Jun/20
Also (sec(x)+tan (x)) has terms independent of x. ln∣sec x+tan x∣ does not have terms independent of x.
$$\mathrm{Also}\:\left(\mathrm{sec}\left({x}\right)+\mathrm{tan}\:\left({x}\right)\right)\:\mathrm{has}\:\mathrm{terms} \\ $$$$\mathrm{independent}\:\mathrm{of}\:{x}.\: \\ $$$$\mathrm{ln}\mid\mathrm{sec}\:{x}+\mathrm{tan}\:{x}\mid\:\:\mathrm{does}\:\mathrm{not}\:\mathrm{have} \\ $$$$\mathrm{terms}\:\mathrm{independent}\:\mathrm{of}\:{x}. \\ $$
Commented by Ar Brandon last updated on 04/Jun/20
OK thanks Sir for your opinion. I tried a different approach.
$$\mathrm{OK}\:\mathrm{thanks}\:\mathrm{Sir}\:\mathrm{for}\:\mathrm{your}\:\mathrm{opinion}.\:\mathrm{I}\:\mathrm{tried}\:\mathrm{a}\:\mathrm{different} \\ $$$$\mathrm{approach}. \\ $$
Commented by 1549442205 last updated on 05/Jun/20
Clearly,by your argument it follows that the equality (du/dx)=(dv/dx) is simple a need condition to u=v but it isn′t a enough condition to u=v
$$\mathrm{Clearly},\mathrm{by}\:\mathrm{your}\:\mathrm{argument}\:\mathrm{it}\:\mathrm{follows}\:\mathrm{that}\:\mathrm{the}\:\mathrm{equality} \\ $$$$\frac{\mathrm{du}}{\mathrm{dx}}=\frac{\mathrm{dv}}{\mathrm{dx}}\:\mathrm{is}\:\mathrm{simple}\:\mathrm{a}\:\mathrm{need}\:\mathrm{condition}\:\mathrm{to}\:\mathrm{u}=\mathrm{v}\:\mathrm{but} \\ $$$$\mathrm{it}\:\mathrm{isn}'\mathrm{t}\:\mathrm{a}\:\mathrm{enough}\:\mathrm{condition}\:\mathrm{to}\:\mathrm{u}=\mathrm{v} \\ $$
Answered by Ar Brandon last updated on 04/Jun/20
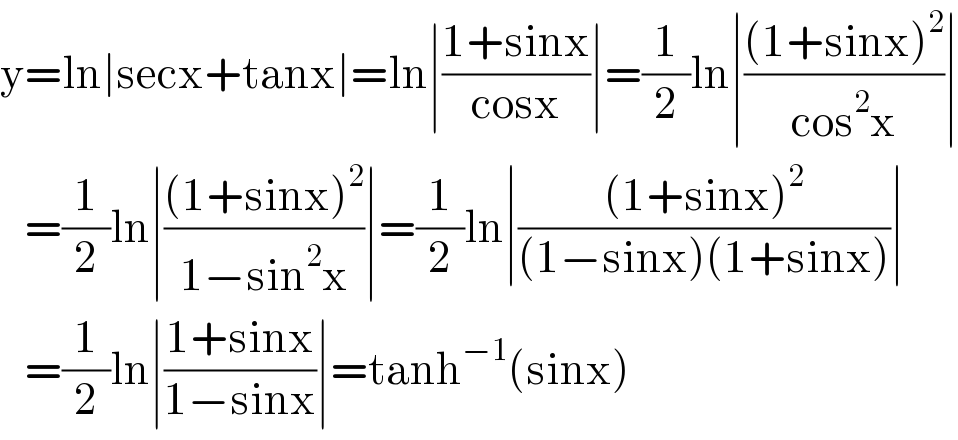
y=ln∣secx+tanx∣=ln∣((1+sinx)/(cosx))∣=(1/2)ln∣(((1+sinx)^2 )/(cos^2 x))∣ =(1/2)ln∣(((1+sinx)^2 )/(1−sin^2 x))∣=(1/2)ln∣(((1+sinx)^2 )/((1−sinx)(1+sinx)))∣ =(1/2)ln∣((1+sinx)/(1−sinx))∣=tanh^(−1) (sinx)
$$\mathrm{y}=\mathrm{ln}\mid\mathrm{secx}+\mathrm{tanx}\mid=\mathrm{ln}\mid\frac{\mathrm{1}+\mathrm{sinx}}{\mathrm{cosx}}\mid=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\frac{\left(\mathrm{1}+\mathrm{sinx}\right)^{\mathrm{2}} }{\mathrm{cos}^{\mathrm{2}} \mathrm{x}}\mid \\ $$$$\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\frac{\left(\mathrm{1}+\mathrm{sinx}\right)^{\mathrm{2}} }{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \mathrm{x}}\mid=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\frac{\left(\mathrm{1}+\mathrm{sinx}\right)^{\mathrm{2}} }{\left(\mathrm{1}−\mathrm{sinx}\right)\left(\mathrm{1}+\mathrm{sinx}\right)}\mid \\ $$$$\:\:\:=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{ln}\mid\frac{\mathrm{1}+\mathrm{sinx}}{\mathrm{1}−\mathrm{sinx}}\mid=\mathrm{tanh}^{−\mathrm{1}} \left(\mathrm{sinx}\right) \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *