Menu Close

proof-that-2-n-1-gt-n-2-sin-n-

Tinku Tara 0 Comments
Pin




Question Number 162305 by mathlove last updated on 28/Dec/21
proof that 2^(n+1) >(n+2)sin n
$${proof}\:{that} \\ $$$$\mathrm{2}^{{n}+\mathrm{1}} >\left({n}+\mathrm{2}\right)\mathrm{sin}\:{n} \\ $$
Answered by aleks041103 last updated on 28/Dec/21
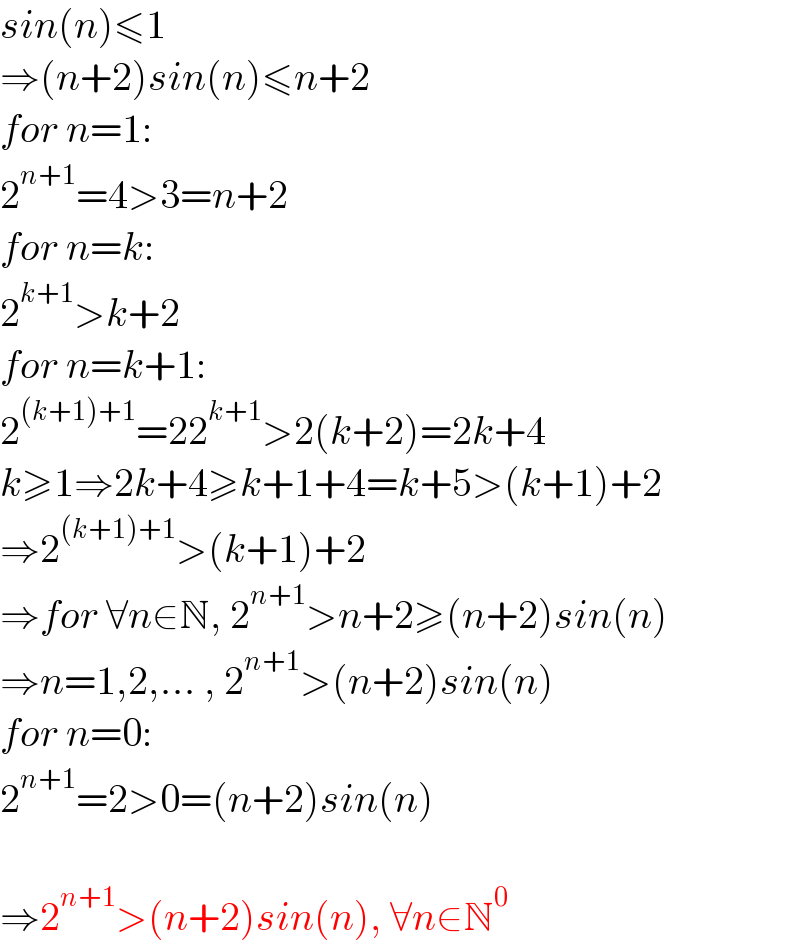
sin(n)≤1 ⇒(n+2)sin(n)≤n+2 for n=1: 2^(n+1) =4>3=n+2 for n=k: 2^(k+1) >k+2 for n=k+1: 2^((k+1)+1) =22^(k+1) >2(k+2)=2k+4 k≥1⇒2k+4≥k+1+4=k+5>(k+1)+2 ⇒2^((k+1)+1) >(k+1)+2 ⇒for ∀n∈N, 2^(n+1) >n+2≥(n+2)sin(n) ⇒n=1,2,... , 2^(n+1) >(n+2)sin(n) for n=0: 2^(n+1) =2>0=(n+2)sin(n) ⇒2^(n+1) >(n+2)sin(n), ∀n∈N^0
$${sin}\left({n}\right)\leqslant\mathrm{1} \\ $$$$\Rightarrow\left({n}+\mathrm{2}\right){sin}\left({n}\right)\leqslant{n}+\mathrm{2} \\ $$$${for}\:{n}=\mathrm{1}: \\ $$$$\mathrm{2}^{{n}+\mathrm{1}} =\mathrm{4}>\mathrm{3}={n}+\mathrm{2} \\ $$$${for}\:{n}={k}: \\ $$$$\mathrm{2}^{{k}+\mathrm{1}} >{k}+\mathrm{2} \\ $$$${for}\:{n}={k}+\mathrm{1}: \\ $$$$\mathrm{2}^{\left({k}+\mathrm{1}\right)+\mathrm{1}} =\mathrm{22}^{{k}+\mathrm{1}} >\mathrm{2}\left({k}+\mathrm{2}\right)=\mathrm{2}{k}+\mathrm{4} \\ $$$${k}\geqslant\mathrm{1}\Rightarrow\mathrm{2}{k}+\mathrm{4}\geqslant{k}+\mathrm{1}+\mathrm{4}={k}+\mathrm{5}>\left({k}+\mathrm{1}\right)+\mathrm{2} \\ $$$$\Rightarrow\mathrm{2}^{\left({k}+\mathrm{1}\right)+\mathrm{1}} >\left({k}+\mathrm{1}\right)+\mathrm{2} \\ $$$$\Rightarrow{for}\:\forall{n}\in\mathbb{N},\:\mathrm{2}^{{n}+\mathrm{1}} >{n}+\mathrm{2}\geqslant\left({n}+\mathrm{2}\right){sin}\left({n}\right) \\ $$$$\Rightarrow{n}=\mathrm{1},\mathrm{2},…\:,\:\mathrm{2}^{{n}+\mathrm{1}} >\left({n}+\mathrm{2}\right){sin}\left({n}\right) \\ $$$${for}\:{n}=\mathrm{0}: \\ $$$$\mathrm{2}^{{n}+\mathrm{1}} =\mathrm{2}>\mathrm{0}=\left({n}+\mathrm{2}\right){sin}\left({n}\right) \\ $$$$ \\ $$$$\Rightarrow\mathrm{2}^{{n}+\mathrm{1}} >\left({n}+\mathrm{2}\right){sin}\left({n}\right),\:\forall{n}\in\mathbb{N}^{\mathrm{0}} \\ $$
Answered by mr W last updated on 28/Dec/21
recall: 1≥sin n for any n∈R (1+a)^n >1+na for a>0 2^(n+1) =(1+1)^(n+1) >1+(n+1)×1=n+2=(n+2)×1 ≥(n+2) sin n ⇒ 2^(n+1) >(n+2) sin n ✓
$${recall}: \\ $$$$\mathrm{1}\geqslant\mathrm{sin}\:{n}\:{for}\:{any}\:{n}\in\mathbb{R} \\ $$$$\left(\mathrm{1}+{a}\right)^{{n}} >\mathrm{1}+{na}\:{for}\:{a}>\mathrm{0} \\ $$$$ \\ $$$$\mathrm{2}^{{n}+\mathrm{1}} =\left(\mathrm{1}+\mathrm{1}\right)^{{n}+\mathrm{1}} \\ $$$$\:\:\:\:\:\:\:\:\:>\mathrm{1}+\left({n}+\mathrm{1}\right)×\mathrm{1}={n}+\mathrm{2}=\left({n}+\mathrm{2}\right)×\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\geqslant\left({n}+\mathrm{2}\right)\:\mathrm{sin}\:{n} \\ $$$$\Rightarrow\:\mathrm{2}^{{n}+\mathrm{1}} >\left({n}+\mathrm{2}\right)\:\mathrm{sin}\:{n}\:\checkmark \\ $$
Commented by aleks041103 last updated on 28/Dec/21
It is worth noting that (n+2)≥(n+2)sin(n) is true for n≥−2
$${It}\:{is}\:{worth}\:{noting}\:{that} \\ $$$$\left({n}+\mathrm{2}\right)\geqslant\left({n}+\mathrm{2}\right){sin}\left({n}\right) \\ $$$${is}\:{true}\:{for}\:{n}\geqslant−\mathrm{2} \\ $$
Commented by mr W last updated on 29/Dec/21
i assumed n∈N what the questioner also meant, i think. if n∈Z, then 2^(n+1) >(n+2) sin n is true for n≥−6.
$${i}\:{assumed}\:{n}\in\mathbb{N}\:{what}\:{the}\:{questioner} \\ $$$${also}\:{meant},\:{i}\:{think}. \\ $$$${if}\:{n}\in\mathbb{Z},\:{then}\:\mathrm{2}^{{n}+\mathrm{1}} >\left({n}+\mathrm{2}\right)\:\mathrm{sin}\:{n}\:{is} \\ $$$${true}\:{for}\:{n}\geqslant−\mathrm{6}. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *