Question Number 31382 by Tinkutara last updated on 07/Mar/18

Answered by mrW2 last updated on 07/Mar/18

Commented by mrW2 last updated on 07/Mar/18
![x_A =s y_A =0 v_(Ax) =(ds/dt)=v_A tan θ=(h/s) (1/(cos^2 θ))×(dθ/dt)=−((hv_A )/s^2 ) ⇒ω=(dθ/dt)=−((hv_A cos^2 θ)/s^2 )=−((hv_A )/(s^2 +h^2 )) x_B =x_A −l cos θ y_B =l sin θ v_(Bx) =(dx_B /dt)=v_A +l sin θ (dθ/dt)=v_A [1−((lh^2 )/((s^2 +h^2 )^(3/2) ))] v_(By) =(dy_B /dt)=l cos θ (dθ/dt)=−((lhv_A s)/((s^2 +h^2 )^(3/2) )) at s=h: v_(Bx) =v_A [1−(l/(2(√2)h))] v_(By) =−((lv_A )/(2(√2)h)) v_B =(v_A /(2(√2)h))(√(l^2 +(2(√2)h−l)^2 ))=(v_A /(2h))(√(l^2 +4h^2 −2(√2)hl)) ω=−(v_A /(2h))](https://www.tinkutara.com/question/Q31393.png)
$${x}_{{A}} ={s} \\ $$$${y}_{{A}} =\mathrm{0} \\ $$$${v}_{{Ax}} =\frac{{ds}}{{dt}}={v}_{{A}} \\ $$$$\mathrm{tan}\:\theta=\frac{{h}}{{s}} \\ $$$$\frac{\mathrm{1}}{\mathrm{cos}^{\mathrm{2}} \:\theta}×\frac{{d}\theta}{{dt}}=−\frac{{hv}_{{A}} }{{s}^{\mathrm{2}} } \\ $$$$\Rightarrow\omega=\frac{{d}\theta}{{dt}}=−\frac{{hv}_{{A}} \:\mathrm{cos}^{\mathrm{2}} \:\theta}{{s}^{\mathrm{2}} }=−\frac{{hv}_{{A}} }{{s}^{\mathrm{2}} +{h}^{\mathrm{2}} } \\ $$$$ \\ $$$${x}_{{B}} ={x}_{{A}} −{l}\:\mathrm{cos}\:\theta \\ $$$${y}_{{B}} ={l}\:\mathrm{sin}\:\theta \\ $$$${v}_{{Bx}} =\frac{{dx}_{{B}} }{{dt}}={v}_{{A}} +{l}\:\mathrm{sin}\:\theta\:\frac{{d}\theta}{{dt}}={v}_{{A}} \left[\mathrm{1}−\frac{{lh}^{\mathrm{2}} }{\left({s}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} }\right] \\ $$$${v}_{{By}} =\frac{{dy}_{{B}} }{{dt}}={l}\:\mathrm{cos}\:\theta\:\frac{{d}\theta}{{dt}}=−\frac{{lhv}_{{A}} {s}}{\left({s}^{\mathrm{2}} +{h}^{\mathrm{2}} \right)^{\frac{\mathrm{3}}{\mathrm{2}}} } \\ $$$${at}\:{s}={h}: \\ $$$${v}_{{Bx}} ={v}_{{A}} \left[\mathrm{1}−\frac{{l}}{\mathrm{2}\sqrt{\mathrm{2}}{h}}\right] \\ $$$${v}_{{By}} =−\frac{{lv}_{{A}} }{\mathrm{2}\sqrt{\mathrm{2}}{h}} \\ $$$${v}_{{B}} =\frac{{v}_{{A}} }{\mathrm{2}\sqrt{\mathrm{2}}{h}}\sqrt{{l}^{\mathrm{2}} +\left(\mathrm{2}\sqrt{\mathrm{2}}{h}−{l}\right)^{\mathrm{2}} }=\frac{{v}_{{A}} }{\mathrm{2}{h}}\sqrt{{l}^{\mathrm{2}} +\mathrm{4}{h}^{\mathrm{2}} −\mathrm{2}\sqrt{\mathrm{2}}{hl}} \\ $$$$\omega=−\frac{{v}_{{A}} }{\mathrm{2}{h}} \\ $$
Commented by Tinkutara last updated on 08/Mar/18
This method is a bit lengthy. Can it be done by ICOR (instantaneous center of rotation)?
Commented by mrW2 last updated on 08/Mar/18
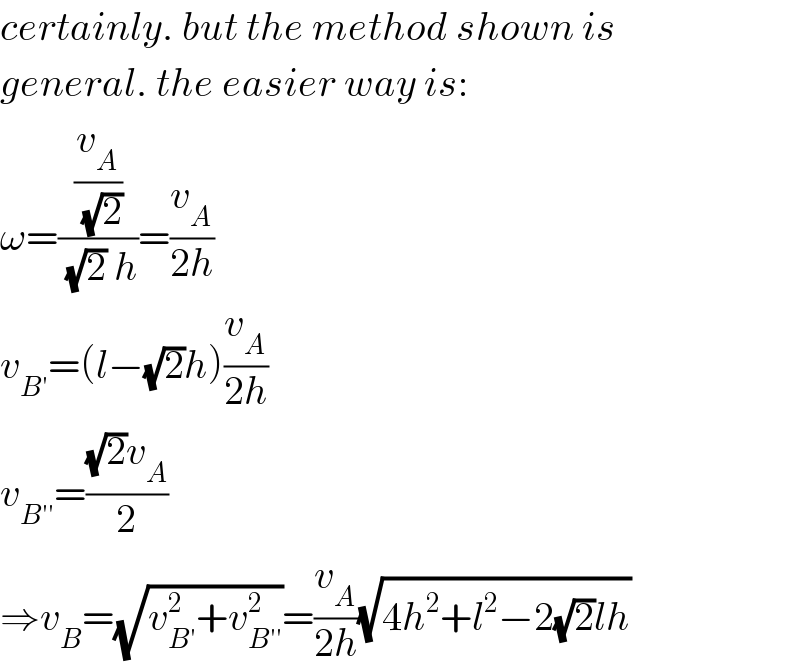
$${certainly}.\:{but}\:{the}\:{method}\:{shown}\:{is} \\ $$$${general}.\:{the}\:{easier}\:{way}\:{is}: \\ $$$$\omega=\frac{\frac{{v}_{{A}} }{\:\sqrt{\mathrm{2}}}}{\:\sqrt{\mathrm{2}}\:{h}}=\frac{{v}_{{A}} }{\mathrm{2}{h}} \\ $$$${v}_{{B}'} =\left({l}−\sqrt{\mathrm{2}}{h}\right)\frac{{v}_{{A}} }{\mathrm{2}{h}} \\ $$$${v}_{{B}''} =\frac{\sqrt{\mathrm{2}}{v}_{{A}} }{\mathrm{2}} \\ $$$$\Rightarrow{v}_{{B}} =\sqrt{{v}_{{B}'} ^{\mathrm{2}} +{v}_{{B}''} ^{\mathrm{2}} }=\frac{{v}_{{A}} }{\mathrm{2}{h}}\sqrt{\mathrm{4}{h}^{\mathrm{2}} +{l}^{\mathrm{2}} −\mathrm{2}\sqrt{\mathrm{2}}{lh}} \\ $$
Commented by Tinkutara last updated on 08/Mar/18
Thanks for the solution ��������
