Question Number 98367 by bobhans last updated on 13/Jun/20
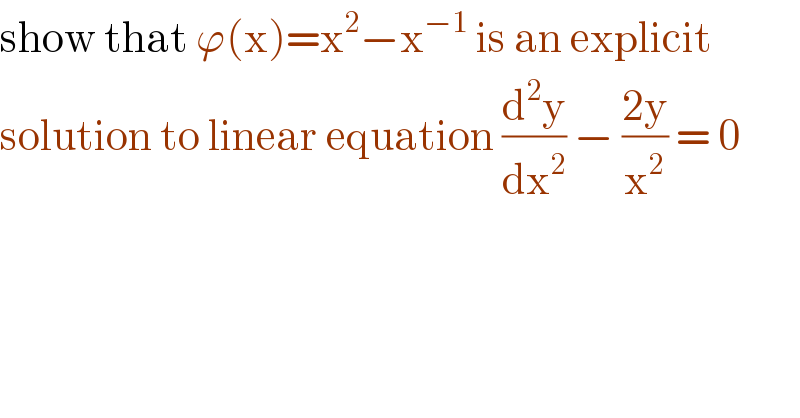
$$\mathrm{show}\:\mathrm{that}\:\varphi\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{2}} −\mathrm{x}^{−\mathrm{1}} \:\mathrm{is}\:\mathrm{an}\:\mathrm{explicit}\: \\ $$$$\mathrm{solution}\:\mathrm{to}\:\mathrm{linear}\:\mathrm{equation}\:\frac{\mathrm{d}^{\mathrm{2}} \mathrm{y}}{\mathrm{dx}^{\mathrm{2}} }\:−\:\frac{\mathrm{2y}}{\mathrm{x}^{\mathrm{2}} }\:=\:\mathrm{0} \\ $$
Commented by john santu last updated on 13/Jun/20

$$\mathrm{the}\:\mathrm{function}\:\varphi\left(\mathrm{x}\right)=\:\mathrm{x}^{\mathrm{2}} −\mathrm{x}^{−\mathrm{1}} \:,\:\varphi^{'} \left(\mathrm{x}\right)=\:\mathrm{2x}+\mathrm{x}^{−\mathrm{2}} \:\mathrm{and} \\ $$$$\varphi''\left(\mathrm{x}\right)=\mathrm{2}−\mathrm{2x}^{−\mathrm{3}} \:\mathrm{are}\:\mathrm{defined}\:\mathrm{for}\:\mathrm{all}\:\mathrm{x}\neq\mathrm{0} \\ $$$$\mathrm{substitution}\:\mathrm{of}\:\varphi\left(\mathrm{x}\right)\:\mathrm{for}\:\mathrm{y}\:\mathrm{in}\:\mathrm{equation} \\ $$$$\mathrm{gives}\:\left(\mathrm{2}−\mathrm{2x}^{−\mathrm{3}} \right)−\frac{\mathrm{2}}{\mathrm{x}^{\mathrm{2}} }\left(\mathrm{x}^{\mathrm{2}} −\mathrm{x}^{−\mathrm{1}} \right)= \\ $$$$\left(\mathrm{2}−\mathrm{2x}^{−\mathrm{3}} \right)−\left(\mathrm{2}−\mathrm{2x}^{−\mathrm{3}} \right)\:=\:\mathrm{0}\:.\:\mathrm{since}\:\mathrm{this} \\ $$$$\mathrm{is}\:\mathrm{valid}\:\mathrm{for}\:\mathrm{any}\:\mathrm{x}\neq\mathrm{0}\:,\:\mathrm{the}\:\mathrm{function} \\ $$$$\varphi\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{2}} −\mathrm{x}^{−\mathrm{1}} \:\mathrm{is}\:\mathrm{an}\:\mathrm{explicit}\:\mathrm{solution} \\ $$$$\mathrm{for}\:\frac{\mathrm{d}^{\mathrm{2}} \mathrm{y}}{\mathrm{dx}^{\mathrm{2}} }\:−\frac{\mathrm{2y}}{\mathrm{x}^{\mathrm{2}} }\:=\:\mathrm{0}\:\mathrm{on}\:\left(−\infty,\mathrm{0}\right)\:\cup\left(\mathrm{0},\infty\right) \\ $$
Commented by bobhans last updated on 13/Jun/20

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{both} \\ $$
Answered by abdomathmax last updated on 13/Jun/20
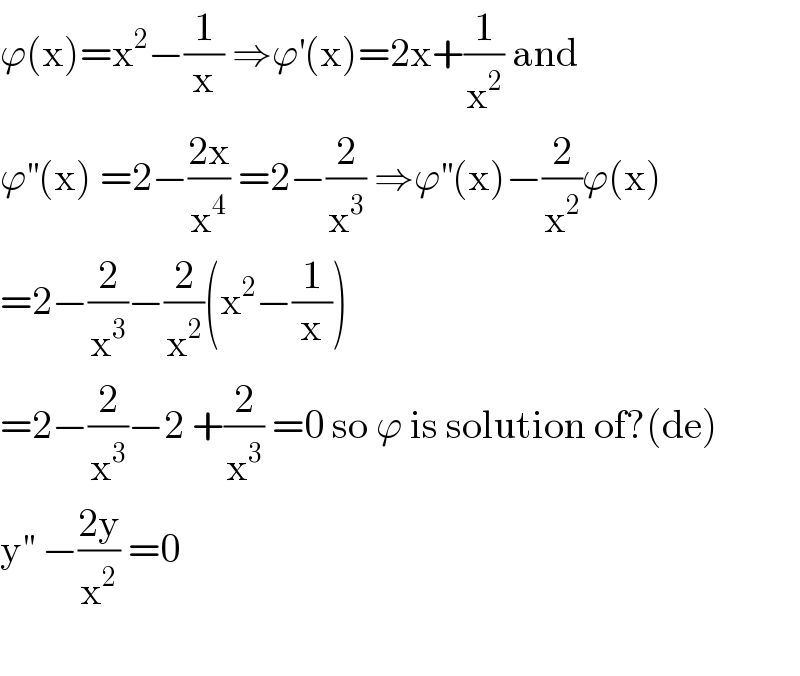
$$\varphi\left(\mathrm{x}\right)=\mathrm{x}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{x}}\:\Rightarrow\varphi^{'} \left(\mathrm{x}\right)=\mathrm{2x}+\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }\:\mathrm{and} \\ $$$$\varphi^{''} \left(\mathrm{x}\right)\:=\mathrm{2}−\frac{\mathrm{2x}}{\mathrm{x}^{\mathrm{4}} }\:=\mathrm{2}−\frac{\mathrm{2}}{\mathrm{x}^{\mathrm{3}} }\:\Rightarrow\varphi^{''} \left(\mathrm{x}\right)−\frac{\mathrm{2}}{\mathrm{x}^{\mathrm{2}} }\varphi\left(\mathrm{x}\right) \\ $$$$=\mathrm{2}−\frac{\mathrm{2}}{\mathrm{x}^{\mathrm{3}} }−\frac{\mathrm{2}}{\mathrm{x}^{\mathrm{2}} }\left(\mathrm{x}^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{x}}\right) \\ $$$$=\mathrm{2}−\frac{\mathrm{2}}{\mathrm{x}^{\mathrm{3}} }−\mathrm{2}\:+\frac{\mathrm{2}}{\mathrm{x}^{\mathrm{3}} }\:=\mathrm{0}\:\mathrm{so}\:\varphi\:\mathrm{is}\:\mathrm{solution}\:\mathrm{of}?\left(\mathrm{de}\right) \\ $$$$\mathrm{y}^{''} \:−\frac{\mathrm{2y}}{\mathrm{x}^{\mathrm{2}} }\:=\mathrm{0} \\ $$$$ \\ $$
