Menu Close

let-f-x-1-1-x-2-1-calculate-f-n-x-2-find-f-n-0-3-developp-f-x-at-integr-serie-

Tinku Tara 0 Comments
Pin




Question Number 33256 by prof Abdo imad last updated on 14/Apr/18
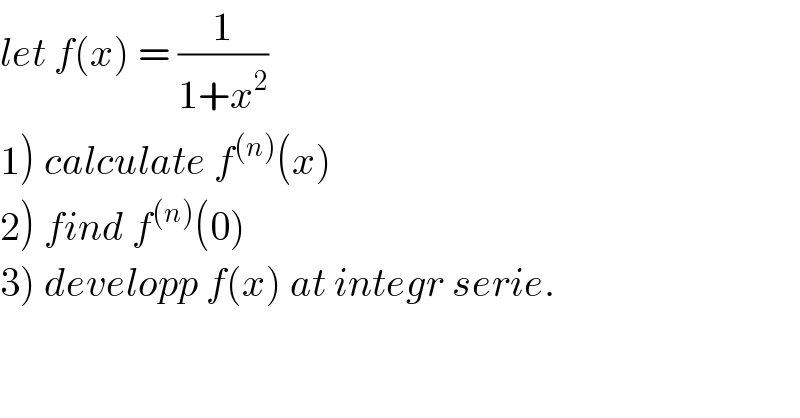
let f(x) = (1/(1+x^2 )) 1) calculate f^((n)) (x) 2) find f^((n)) (0) 3) developp f(x) at integr serie.
$${let}\:{f}\left({x}\right)\:=\:\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} } \\ $$$$\left.\mathrm{1}\right)\:{calculate}\:{f}^{\left({n}\right)} \left({x}\right) \\ $$$$\left.\mathrm{2}\right)\:{find}\:{f}^{\left({n}\right)} \left(\mathrm{0}\right) \\ $$$$\left.\mathrm{3}\right)\:{developp}\:{f}\left({x}\right)\:{at}\:{integr}\:{serie}. \\ $$$$ \\ $$
Commented by prof Abdo imad last updated on 15/Apr/18
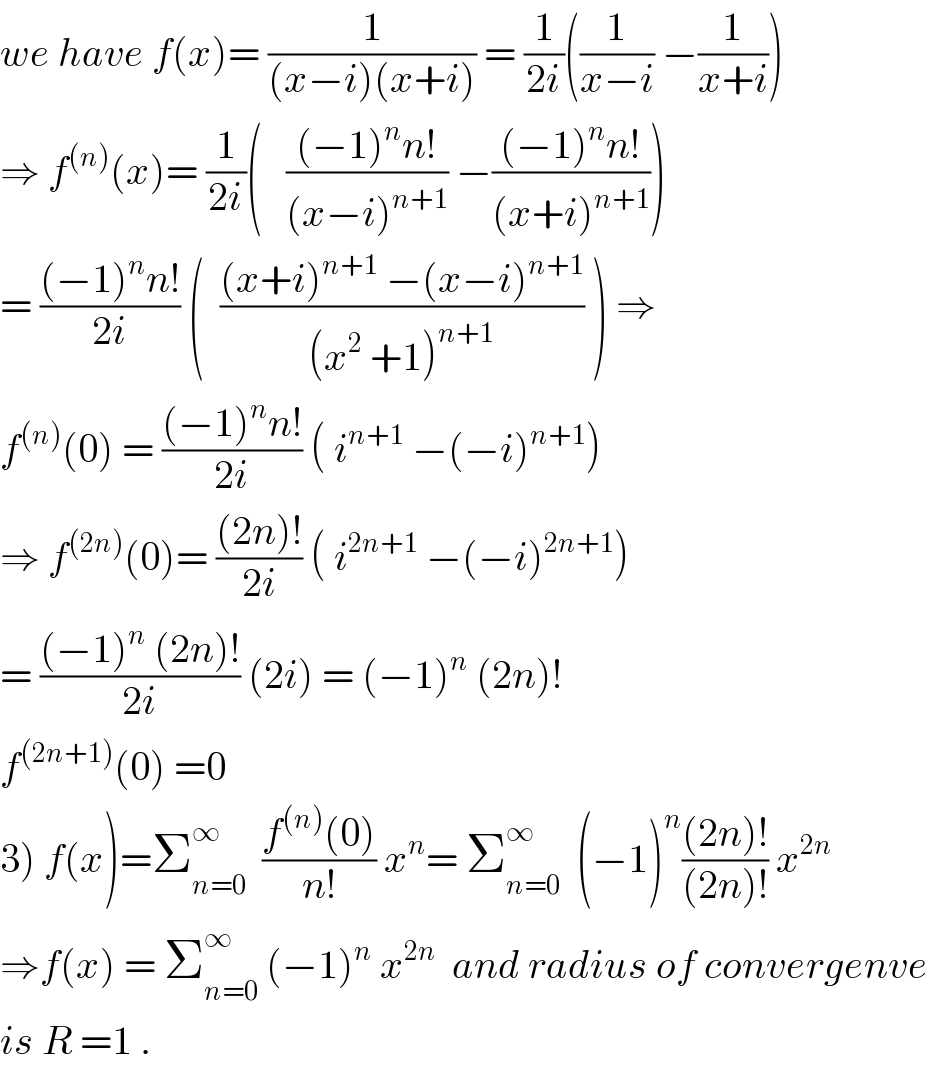
we have f(x)= (1/((x−i)(x+i))) = (1/(2i))((1/(x−i)) −(1/(x+i))) ⇒ f^((n)) (x)= (1/(2i))( (((−1)^n n!)/((x−i)^(n+1) )) −(((−1)^n n!)/((x+i)^(n+1) ))) = (((−1)^n n!)/(2i)) ( (((x+i)^(n+1) −(x−i)^(n+1) )/((x^2 +1)^(n+1) )) ) ⇒ f^((n)) (0) = (((−1)^n n!)/(2i)) ( i^(n+1) −(−i)^(n+1) ) ⇒ f^((2n)) (0)= (((2n)!)/(2i)) ( i^(2n+1) −(−i)^(2n+1) ) = (((−1)^n (2n)!)/(2i)) (2i) = (−1)^n (2n)! f^((2n+1)) (0) =0 3) f(x)=Σ_(n=0) ^∞ ((f^((n)) (0))/(n!)) x^n = Σ_(n=0) ^∞ (−1)^n (((2n)!)/((2n)!)) x^(2n) ⇒f(x) = Σ_(n=0) ^∞ (−1)^n x^(2n) and radius of convergenve is R =1 .
$${we}\:{have}\:{f}\left({x}\right)=\:\frac{\mathrm{1}}{\left({x}−{i}\right)\left({x}+{i}\right)}\:=\:\frac{\mathrm{1}}{\mathrm{2}{i}}\left(\frac{\mathrm{1}}{{x}−{i}}\:−\frac{\mathrm{1}}{{x}+{i}}\right) \\ $$$$\Rightarrow\:{f}^{\left({n}\right)} \left({x}\right)=\:\frac{\mathrm{1}}{\mathrm{2}{i}}\left(\:\:\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\left({x}−{i}\right)^{{n}+\mathrm{1}} }\:−\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\left({x}+{i}\right)^{{n}+\mathrm{1}} }\right) \\ $$$$=\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{2}{i}}\:\left(\:\:\frac{\left({x}+{i}\right)^{{n}+\mathrm{1}} \:−\left({x}−{i}\right)^{{n}+\mathrm{1}} }{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)^{{n}+\mathrm{1}} }\:\right)\:\Rightarrow \\ $$$${f}^{\left({n}\right)} \left(\mathrm{0}\right)\:=\:\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\mathrm{2}{i}}\:\left(\:{i}^{{n}+\mathrm{1}} \:−\left(−{i}\right)^{{n}+\mathrm{1}} \right) \\ $$$$\Rightarrow\:{f}^{\left(\mathrm{2}{n}\right)} \left(\mathrm{0}\right)=\:\frac{\left(\mathrm{2}{n}\right)!}{\mathrm{2}{i}}\:\left(\:{i}^{\mathrm{2}{n}+\mathrm{1}} \:−\left(−{i}\right)^{\mathrm{2}{n}+\mathrm{1}} \right) \\ $$$$=\:\frac{\left(−\mathrm{1}\right)^{{n}} \:\left(\mathrm{2}{n}\right)!}{\mathrm{2}{i}}\:\left(\mathrm{2}{i}\right)\:=\:\left(−\mathrm{1}\right)^{{n}} \:\left(\mathrm{2}{n}\right)! \\ $$$${f}^{\left(\mathrm{2}{n}+\mathrm{1}\right)} \left(\mathrm{0}\right)\:=\mathrm{0} \\ $$$$\left.\mathrm{3}\right)\:{f}\left({x}\right)=\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\frac{{f}^{\left({n}\right)} \left(\mathrm{0}\right)}{{n}!}\:{x}^{{n}} =\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\left(−\mathrm{1}\right)^{{n}} \frac{\left(\mathrm{2}{n}\right)!}{\left(\mathrm{2}{n}\right)!}\:{x}^{\mathrm{2}{n}} \\ $$$$\Rightarrow{f}\left({x}\right)\:=\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\left(−\mathrm{1}\right)^{{n}} \:{x}^{\mathrm{2}{n}} \:\:{and}\:{radius}\:{of}\:{convergenve} \\ $$$${is}\:{R}\:=\mathrm{1}\:. \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *