Question Number 99261 by Ar Brandon last updated on 19/Jun/20
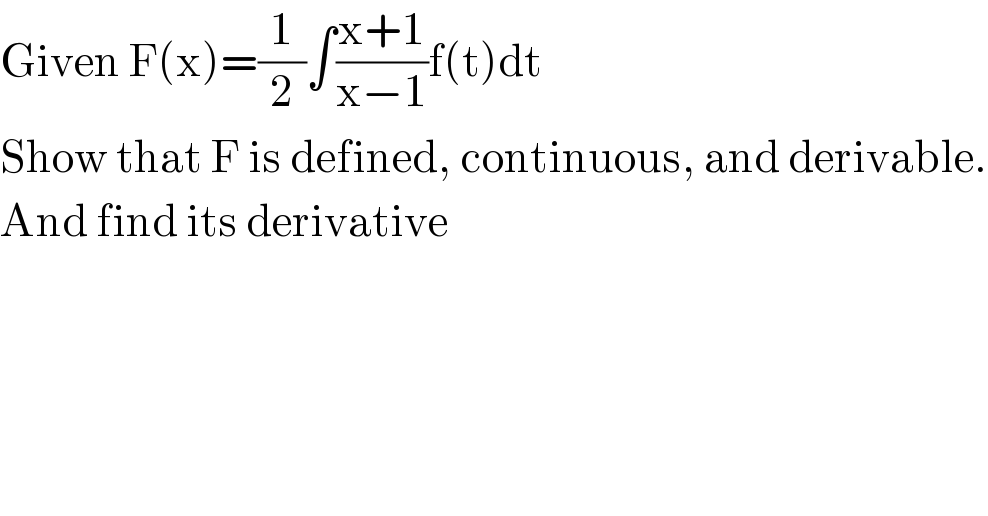
$$\mathrm{Given}\:\mathrm{F}\left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{2}}\int\frac{\mathrm{x}+\mathrm{1}}{\mathrm{x}−\mathrm{1}}\mathrm{f}\left(\mathrm{t}\right)\mathrm{dt} \\ $$$$\mathrm{Show}\:\mathrm{that}\:\mathrm{F}\:\mathrm{is}\:\mathrm{defined},\:\mathrm{continuous},\:\mathrm{and}\:\mathrm{derivable}. \\ $$$$\mathrm{And}\:\mathrm{find}\:\mathrm{its}\:\mathrm{derivative} \\ $$
Answered by abdomathmax last updated on 19/Jun/20
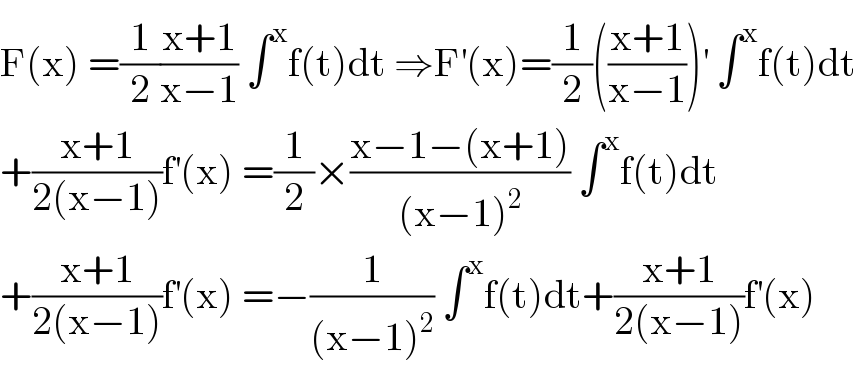
$$\mathrm{F}\left(\mathrm{x}\right)\:=\frac{\mathrm{1}}{\mathrm{2}}\frac{\mathrm{x}+\mathrm{1}}{\mathrm{x}−\mathrm{1}}\:\int^{\mathrm{x}} \mathrm{f}\left(\mathrm{t}\right)\mathrm{dt}\:\Rightarrow\mathrm{F}^{'} \left(\mathrm{x}\right)=\frac{\mathrm{1}}{\mathrm{2}}\left(\frac{\mathrm{x}+\mathrm{1}}{\mathrm{x}−\mathrm{1}}\right)^{'} \:\int^{\mathrm{x}} \mathrm{f}\left(\mathrm{t}\right)\mathrm{dt} \\ $$$$+\frac{\mathrm{x}+\mathrm{1}}{\mathrm{2}\left(\mathrm{x}−\mathrm{1}\right)}\mathrm{f}^{'} \left(\mathrm{x}\right)\:=\frac{\mathrm{1}}{\mathrm{2}}×\frac{\mathrm{x}−\mathrm{1}−\left(\mathrm{x}+\mathrm{1}\right)}{\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} }\:\int^{\mathrm{x}} \mathrm{f}\left(\mathrm{t}\right)\mathrm{dt} \\ $$$$+\frac{\mathrm{x}+\mathrm{1}}{\mathrm{2}\left(\mathrm{x}−\mathrm{1}\right)}\mathrm{f}^{'} \left(\mathrm{x}\right)\:=−\frac{\mathrm{1}}{\left(\mathrm{x}−\mathrm{1}\right)^{\mathrm{2}} }\:\int^{\mathrm{x}} \mathrm{f}\left(\mathrm{t}\right)\mathrm{dt}+\frac{\mathrm{x}+\mathrm{1}}{\mathrm{2}\left(\mathrm{x}−\mathrm{1}\right)}\mathrm{f}^{'} \left(\mathrm{x}\right) \\ $$
