Question Number 99278 by Rio Michael last updated on 19/Jun/20
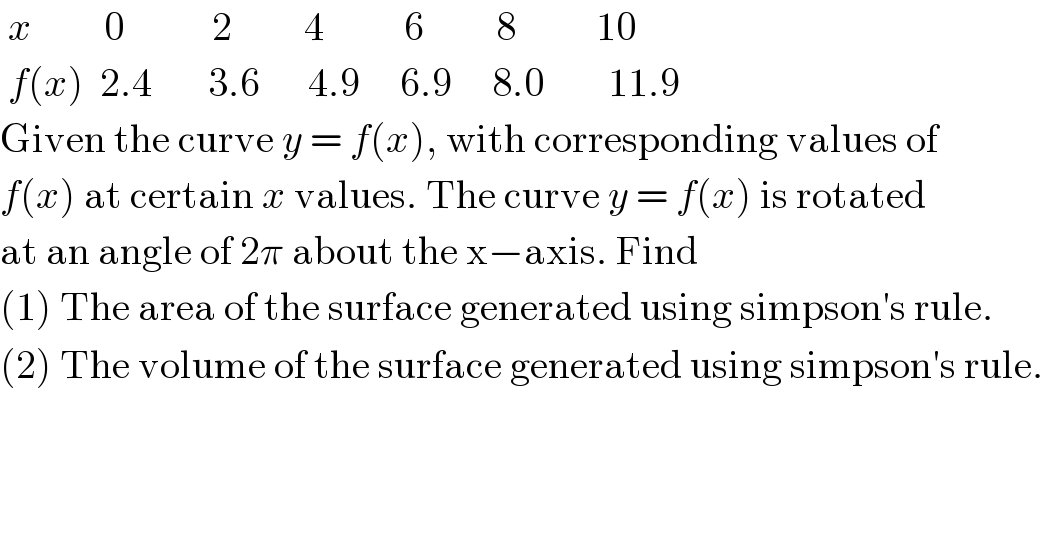
$$\:{x}\:\:\:\:\:\:\:\:\:\mathrm{0}\:\:\:\:\:\:\:\:\:\:\:\mathrm{2}\:\:\:\:\:\:\:\:\:\mathrm{4}\:\:\:\:\:\:\:\:\:\:\mathrm{6}\:\:\:\:\:\:\:\:\:\mathrm{8}\:\:\:\:\:\:\:\:\:\:\mathrm{10} \\ $$$$\:{f}\left({x}\right)\:\:\mathrm{2}.\mathrm{4}\:\:\:\:\:\:\:\mathrm{3}.\mathrm{6}\:\:\:\:\:\:\mathrm{4}.\mathrm{9}\:\:\:\:\:\mathrm{6}.\mathrm{9}\:\:\:\:\:\mathrm{8}.\mathrm{0}\:\:\:\:\:\:\:\:\mathrm{11}.\mathrm{9} \\ $$$$\mathrm{Given}\:\mathrm{the}\:\mathrm{curve}\:{y}\:=\:{f}\left({x}\right),\:\mathrm{with}\:\mathrm{corresponding}\:\mathrm{values}\:\mathrm{of} \\ $$$${f}\left({x}\right)\:\mathrm{at}\:\mathrm{certain}\:{x}\:\mathrm{values}.\:\mathrm{The}\:\mathrm{curve}\:{y}\:=\:{f}\left({x}\right)\:\mathrm{is}\:\mathrm{rotated} \\ $$$$\mathrm{at}\:\mathrm{an}\:\mathrm{angle}\:\mathrm{of}\:\mathrm{2}\pi\:\mathrm{about}\:\mathrm{the}\:\mathrm{x}−\mathrm{axis}.\:\mathrm{Find}\: \\ $$$$\left(\mathrm{1}\right)\:\mathrm{The}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{surface}\:\mathrm{generated}\:\mathrm{using}\:\mathrm{simpson}'\mathrm{s}\:\mathrm{rule}. \\ $$$$\left(\mathrm{2}\right)\:\mathrm{The}\:\mathrm{volume}\:\mathrm{of}\:\mathrm{the}\:\mathrm{surface}\:\mathrm{generated}\:\mathrm{using}\:\mathrm{simpson}'\mathrm{s}\:\mathrm{rule}. \\ $$
