Question-99516

Question Number 99516 by 175 last updated on 21/Jun/20

Answered by mathmax by abdo last updated on 21/Jun/20

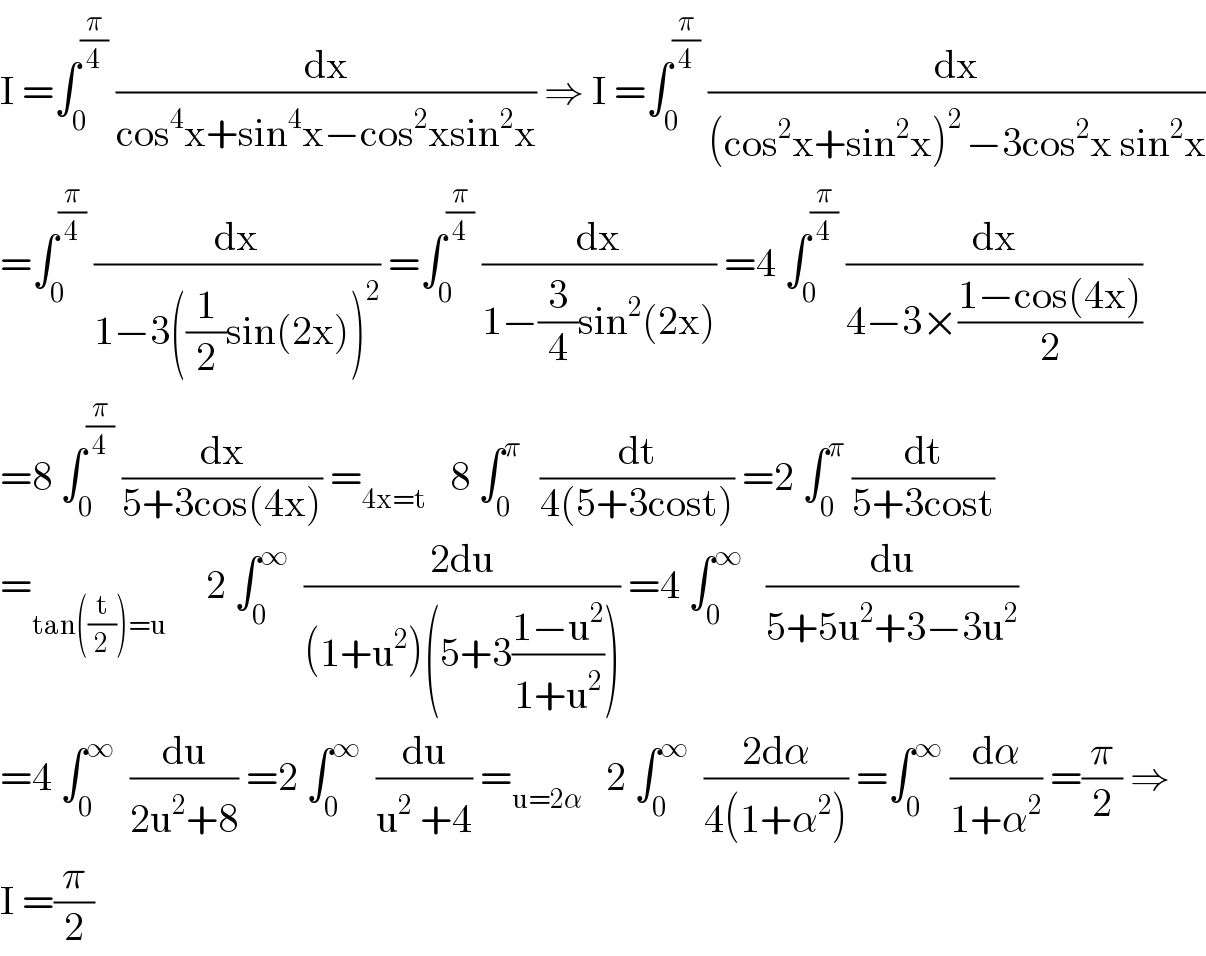
I =∫_0 ^(π/4) (dx/(cos^4 x+sin^4 x−cos^2 xsin^2 x)) ⇒ I =∫_0 ^(π/4) (dx/((cos^2 x+sin^2 x)^(2 ) −3cos^2 x sin^2 x)) =∫_0 ^(π/4) (dx/(1−3((1/2)sin(2x))^2 )) =∫_0 ^(π/4) (dx/(1−(3/4)sin^2 (2x))) =4 ∫_0 ^(π/4) (dx/(4−3×((1−cos(4x))/2))) =8 ∫_0 ^(π/4) (dx/(5+3cos(4x))) =_(4x=t) 8 ∫_0 ^(π ) (dt/(4(5+3cost))) =2 ∫_0 ^π (dt/(5+3cost)) =_(tan((t/2))=u) 2 ∫_0 ^∞ ((2du)/((1+u^2 )(5+3((1−u^2 )/(1+u^2 ))))) =4 ∫_0 ^∞ (du/(5+5u^2 +3−3u^2 )) =4 ∫_0 ^∞ (du/(2u^2 +8)) =2 ∫_0 ^∞ (du/(u^2 +4)) =_(u=2α) 2 ∫_0 ^∞ ((2dα)/(4(1+α^2 ))) =∫_0 ^∞ (dα/(1+α^2 )) =(π/2) ⇒ I =(π/2)

$$\mathrm{I}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{dx}}{\mathrm{cos}^{\mathrm{4}} \mathrm{x}+\mathrm{sin}^{\mathrm{4}} \mathrm{x}−\mathrm{cos}^{\mathrm{2}} \mathrm{xsin}^{\mathrm{2}} \mathrm{x}}\:\Rightarrow\:\mathrm{I}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{dx}}{\left(\mathrm{cos}^{\mathrm{2}} \mathrm{x}+\mathrm{sin}^{\mathrm{2}} \mathrm{x}\right)^{\mathrm{2}\:} −\mathrm{3cos}^{\mathrm{2}} \mathrm{x}\:\mathrm{sin}^{\mathrm{2}} \mathrm{x}} \\ $$$$=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{dx}}{\mathrm{1}−\mathrm{3}\left(\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin}\left(\mathrm{2x}\right)\right)^{\mathrm{2}} }\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{dx}}{\mathrm{1}−\frac{\mathrm{3}}{\mathrm{4}}\mathrm{sin}^{\mathrm{2}} \left(\mathrm{2x}\right)}\:=\mathrm{4}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{dx}}{\mathrm{4}−\mathrm{3}×\frac{\mathrm{1}−\mathrm{cos}\left(\mathrm{4x}\right)}{\mathrm{2}}} \\ $$$$=\mathrm{8}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{dx}}{\mathrm{5}+\mathrm{3cos}\left(\mathrm{4x}\right)}\:=_{\mathrm{4x}=\mathrm{t}} \:\:\:\mathrm{8}\:\int_{\mathrm{0}} ^{\pi\:} \:\:\frac{\mathrm{dt}}{\mathrm{4}\left(\mathrm{5}+\mathrm{3cost}\right)}\:=\mathrm{2}\:\int_{\mathrm{0}} ^{\pi} \:\frac{\mathrm{dt}}{\mathrm{5}+\mathrm{3cost}} \\ $$$$=_{\mathrm{tan}\left(\frac{\mathrm{t}}{\mathrm{2}}\right)=\mathrm{u}} \:\:\:\:\:\mathrm{2}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{2du}}{\left(\mathrm{1}+\mathrm{u}^{\mathrm{2}} \right)\left(\mathrm{5}+\mathrm{3}\frac{\mathrm{1}−\mathrm{u}^{\mathrm{2}} }{\mathrm{1}+\mathrm{u}^{\mathrm{2}} }\right)}\:=\mathrm{4}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{\mathrm{du}}{\mathrm{5}+\mathrm{5u}^{\mathrm{2}} +\mathrm{3}−\mathrm{3u}^{\mathrm{2}} } \\ $$$$=\mathrm{4}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{du}}{\mathrm{2u}^{\mathrm{2}} +\mathrm{8}}\:=\mathrm{2}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{du}}{\mathrm{u}^{\mathrm{2}} \:+\mathrm{4}}\:=_{\mathrm{u}=\mathrm{2}\alpha} \:\:\:\mathrm{2}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{2d}\alpha}{\mathrm{4}\left(\mathrm{1}+\alpha^{\mathrm{2}} \right)}\:=\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{d}\alpha}{\mathrm{1}+\alpha^{\mathrm{2}} }\:=\frac{\pi}{\mathrm{2}}\:\Rightarrow \\ $$$$\mathrm{I}\:=\frac{\pi}{\mathrm{2}} \\ $$

Commented by 175 last updated on 21/Jun/20

thank you sir

Commented by mathmax by abdo last updated on 21/Jun/20

$$\mathrm{you}\:\mathrm{are}\:\mathrm{welcome} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply