Menu Close

lim-x-ln-x-x-You-can-only-use-series-expansion-sandwich-theorem-

Tinku Tara 0 Comments
Pin




Question Number 34843 by rahul 19 last updated on 11/May/18
lim_ _(x→∞) ((ln x)/x) = ? You can only use series expansion / sandwich theorem!
$$\underset{{x}\rightarrow\infty} {\mathrm{lim}_{} }\:\frac{\mathrm{ln}\:{x}}{{x}}\:=\:? \\ $$$${You}\:{can}\:\:{only}\:{use} \\ $$$${series}\:{expansion}\:/\:{sandwich}\:{theorem}! \\ $$
Commented by abdo mathsup 649 cc last updated on 11/May/18
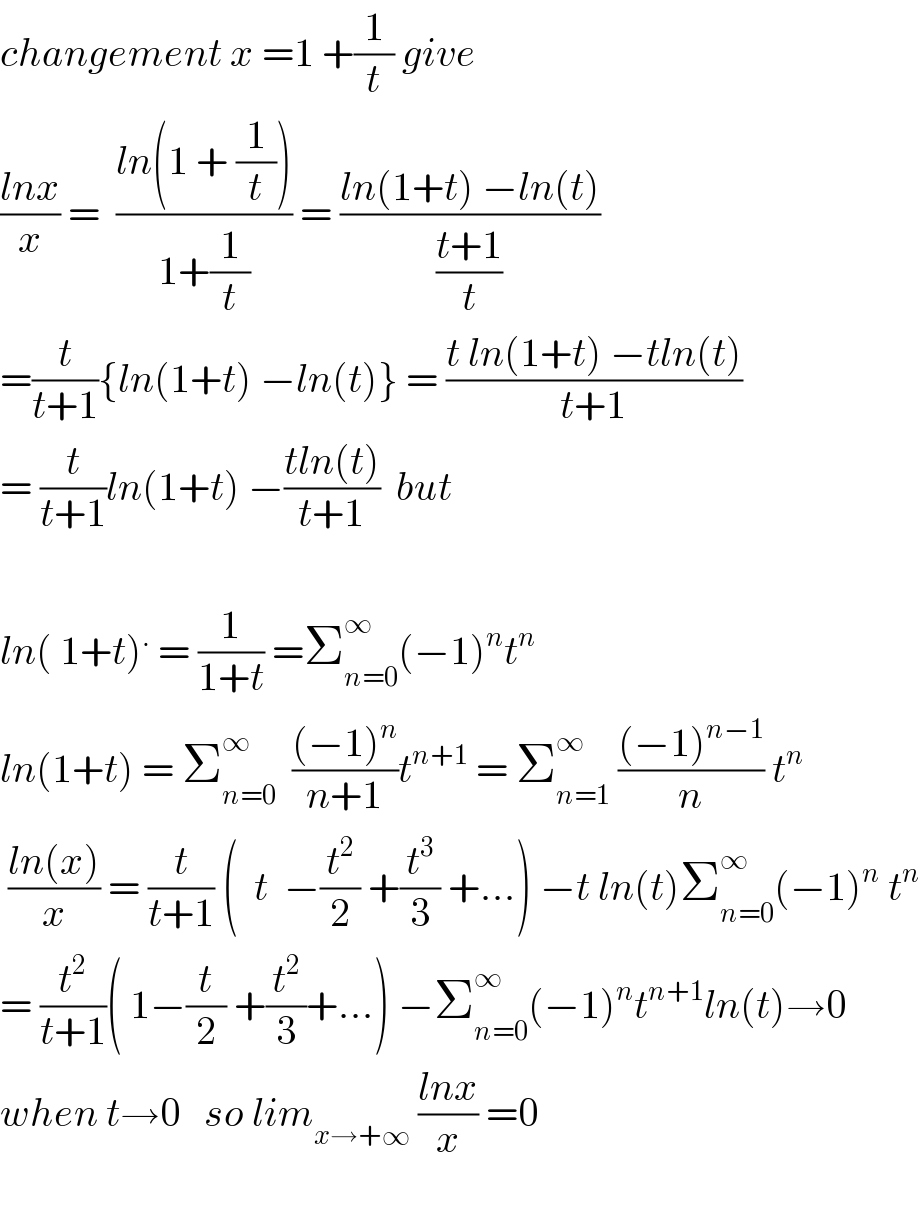
changement x =1 +(1/t) give ((lnx)/x) = ((ln(1 + (1/t)))/(1+(1/t))) = ((ln(1+t) −ln(t))/((t+1)/t)) =(t/(t+1)){ln(1+t) −ln(t)} = ((t ln(1+t) −tln(t))/(t+1)) = (t/(t+1))ln(1+t) −((tln(t))/(t+1)) but ln( 1+t)^. = (1/(1+t)) =Σ_(n=0) ^∞ (−1)^n t^n ln(1+t) = Σ_(n=0) ^∞ (((−1)^n )/(n+1))t^(n+1) = Σ_(n=1) ^∞ (((−1)^(n−1) )/n) t^n ((ln(x))/x) = (t/(t+1)) ( t −(t^2 /2) +(t^3 /3) +...) −t ln(t)Σ_(n=0) ^∞ (−1)^n t^n = (t^2 /(t+1))( 1−(t/2) +(t^2 /3)+...) −Σ_(n=0) ^∞ (−1)^n t^(n+1) ln(t)→0 when t→0 so lim_(x→+∞) ((lnx)/x) =0
$${changement}\:{x}\:=\mathrm{1}\:+\frac{\mathrm{1}}{{t}}\:{give}\:\: \\ $$$$\frac{{lnx}}{{x}}\:=\:\:\frac{{ln}\left(\mathrm{1}\:+\:\frac{\mathrm{1}}{{t}}\right)}{\mathrm{1}+\frac{\mathrm{1}}{{t}}}\:=\:\frac{{ln}\left(\mathrm{1}+{t}\right)\:−{ln}\left({t}\right)}{\frac{{t}+\mathrm{1}}{{t}}} \\ $$$$=\frac{{t}}{{t}+\mathrm{1}}\left\{{ln}\left(\mathrm{1}+{t}\right)\:−{ln}\left({t}\right)\right\}\:=\:\frac{{t}\:{ln}\left(\mathrm{1}+{t}\right)\:−{tln}\left({t}\right)}{{t}+\mathrm{1}} \\ $$$$=\:\frac{{t}}{{t}+\mathrm{1}}{ln}\left(\mathrm{1}+{t}\right)\:−\frac{{tln}\left({t}\right)}{{t}+\mathrm{1}}\:\:{but} \\ $$$$ \\ $$$${ln}\left(\:\mathrm{1}+{t}\right)^{.} \:=\:\frac{\mathrm{1}}{\mathrm{1}+{t}}\:=\sum_{{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{{n}} {t}^{{n}} \\ $$$${ln}\left(\mathrm{1}+{t}\right)\:=\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}+\mathrm{1}}{t}^{{n}+\mathrm{1}} \:=\:\sum_{{n}=\mathrm{1}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}−\mathrm{1}} }{{n}}\:{t}^{{n}} \\ $$$$\:\frac{{ln}\left({x}\right)}{{x}}\:=\:\frac{{t}}{{t}+\mathrm{1}}\:\left(\:\:{t}\:\:−\frac{{t}^{\mathrm{2}} }{\mathrm{2}}\:+\frac{{t}^{\mathrm{3}} }{\mathrm{3}}\:+…\right)\:−{t}\:{ln}\left({t}\right)\sum_{{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{{n}} \:{t}^{{n}} \\ $$$$=\:\frac{{t}^{\mathrm{2}} }{{t}+\mathrm{1}}\left(\:\mathrm{1}−\frac{{t}}{\mathrm{2}}\:+\frac{{t}^{\mathrm{2}} }{\mathrm{3}}+…\right)\:−\sum_{{n}=\mathrm{0}} ^{\infty} \left(−\mathrm{1}\right)^{{n}} {t}^{{n}+\mathrm{1}} {ln}\left({t}\right)\rightarrow\mathrm{0} \\ $$$${when}\:{t}\rightarrow\mathrm{0}\:\:\:{so}\:{lim}_{{x}\rightarrow+\infty} \:\frac{{lnx}}{{x}}\:=\mathrm{0} \\ $$$$ \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *