Question Number 100657 by Cheyboy last updated on 28/Jun/20

$$\int\:\:\frac{\mathrm{3}{x}−\mathrm{1}}{{x}^{\mathrm{2}} +\mathrm{9}}\:{dx} \\ $$
Commented by bobhans last updated on 28/Jun/20
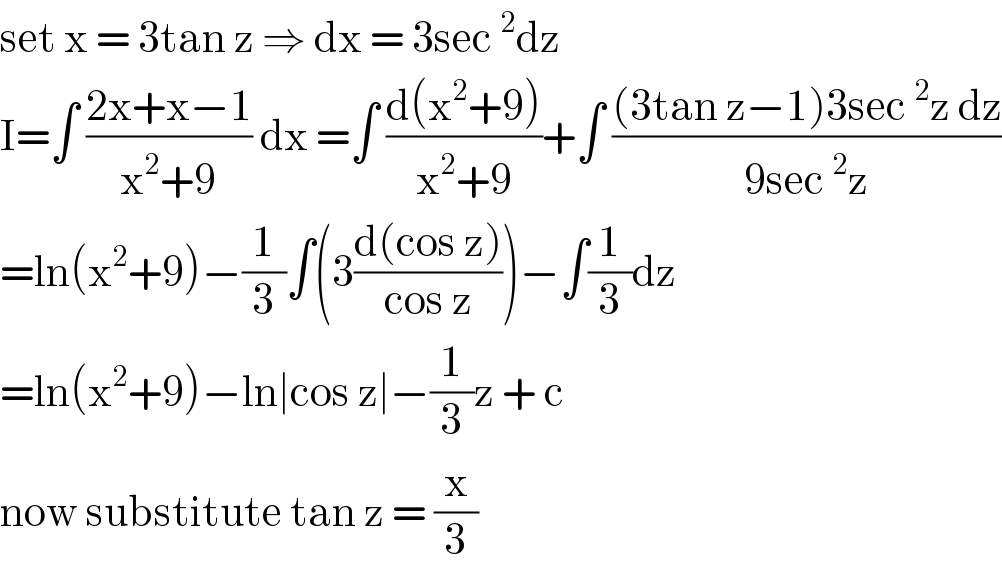
$$\mathrm{set}\:\mathrm{x}\:=\:\mathrm{3tan}\:\mathrm{z}\:\Rightarrow\:\mathrm{dx}\:=\:\mathrm{3sec}\:^{\mathrm{2}} \mathrm{dz} \\ $$$$\mathrm{I}=\int\:\frac{\mathrm{2x}+\mathrm{x}−\mathrm{1}}{\mathrm{x}^{\mathrm{2}} +\mathrm{9}}\:\mathrm{dx}\:=\int\:\frac{\mathrm{d}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{9}\right)}{\mathrm{x}^{\mathrm{2}} +\mathrm{9}}+\int\:\frac{\left(\mathrm{3tan}\:\mathrm{z}−\mathrm{1}\right)\mathrm{3sec}\:^{\mathrm{2}} \mathrm{z}\:\mathrm{dz}}{\mathrm{9sec}\:^{\mathrm{2}} \mathrm{z}} \\ $$$$=\mathrm{ln}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{9}\right)−\frac{\mathrm{1}}{\mathrm{3}}\int\left(\mathrm{3}\frac{\mathrm{d}\left(\mathrm{cos}\:\mathrm{z}\right)}{\mathrm{cos}\:\mathrm{z}}\right)−\int\frac{\mathrm{1}}{\mathrm{3}}\mathrm{dz} \\ $$$$=\mathrm{ln}\left(\mathrm{x}^{\mathrm{2}} +\mathrm{9}\right)−\mathrm{ln}\mid\mathrm{cos}\:\mathrm{z}\mid−\frac{\mathrm{1}}{\mathrm{3}}\mathrm{z}\:+\:\mathrm{c}\: \\ $$$$\mathrm{now}\:\mathrm{substitute}\:\mathrm{tan}\:\mathrm{z}\:=\:\frac{\mathrm{x}}{\mathrm{3}} \\ $$
Commented by Dwaipayan Shikari last updated on 28/Jun/20
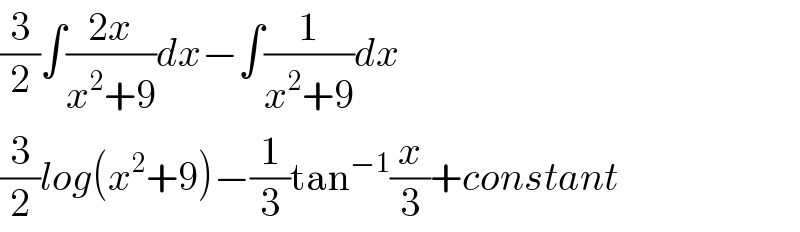
$$\frac{\mathrm{3}}{\mathrm{2}}\int\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\mathrm{9}}{dx}−\int\frac{\mathrm{1}}{{x}^{\mathrm{2}} +\mathrm{9}}{dx} \\ $$$$\frac{\mathrm{3}}{\mathrm{2}}{log}\left({x}^{\mathrm{2}} +\mathrm{9}\right)−\frac{\mathrm{1}}{\mathrm{3}}\mathrm{tan}^{−\mathrm{1}} \frac{{x}}{\mathrm{3}}+{constant} \\ $$
Commented by Cheyboy last updated on 28/Jun/20
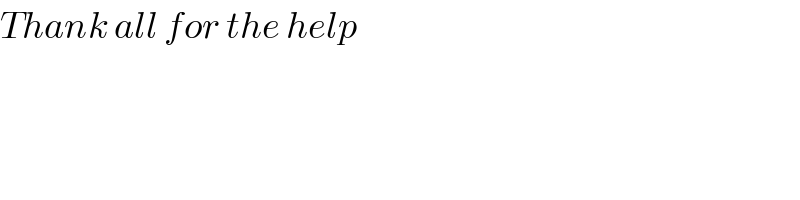
$${Thank}\:{all}\:{for}\:{the}\:{help} \\ $$
Answered by mathmax by abdo last updated on 28/Jun/20
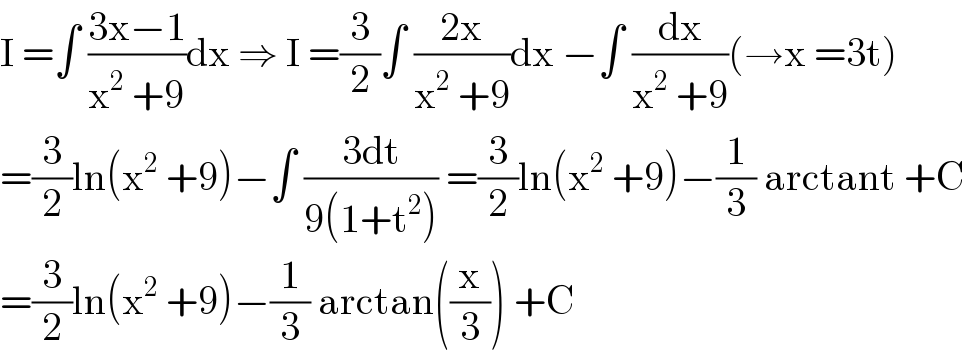
$$\mathrm{I}\:=\int\:\frac{\mathrm{3x}−\mathrm{1}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{9}}\mathrm{dx}\:\Rightarrow\:\mathrm{I}\:=\frac{\mathrm{3}}{\mathrm{2}}\int\:\frac{\mathrm{2x}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{9}}\mathrm{dx}\:−\int\:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{2}} \:+\mathrm{9}}\left(\rightarrow\mathrm{x}\:=\mathrm{3t}\right) \\ $$$$=\frac{\mathrm{3}}{\mathrm{2}}\mathrm{ln}\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{9}\right)−\int\:\frac{\mathrm{3dt}}{\mathrm{9}\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right)}\:=\frac{\mathrm{3}}{\mathrm{2}}\mathrm{ln}\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{9}\right)−\frac{\mathrm{1}}{\mathrm{3}}\:\mathrm{arctant}\:+\mathrm{C} \\ $$$$=\frac{\mathrm{3}}{\mathrm{2}}\mathrm{ln}\left(\mathrm{x}^{\mathrm{2}} \:+\mathrm{9}\right)−\frac{\mathrm{1}}{\mathrm{3}}\:\mathrm{arctan}\left(\frac{\mathrm{x}}{\mathrm{3}}\right)\:+\mathrm{C} \\ $$
