Question Number 100771 by Rio Michael last updated on 28/Jun/20

$$\:\mathrm{sho}\:\mathrm{that}\:\:\left(\mathrm{0},\frac{\mathrm{1}}{\mathrm{2}}\right)\:\mathrm{is}\:\mathrm{a}\:\mathrm{point}\:\mathrm{of}\:\mathrm{symetry}\:\mathrm{for}\:\mathrm{the}\:\mathrm{curve} \\ $$$$\:{f}\left({x}\right)\:=\:{x}\:+\frac{\mathrm{1}}{\mathrm{1}−{e}^{{x}} } \\ $$$$\mathrm{Please}\:\mathrm{make}\:\mathrm{a}\:\mathrm{reference}\:\mathrm{to}\:\mathrm{a}\:\mathrm{book}\:\mathrm{i}\:\mathrm{can}\:\mathrm{understand} \\ $$$$\mathrm{centre}\:\mathrm{of}\:\mathrm{symmetry}\:\mathrm{of}\:\mathrm{rational}\:\mathrm{functions}\:\mathrm{and}\:\mathrm{functions} \\ $$$$\mathrm{like}\:\mathrm{this} \\ $$
Commented by abdomathmax last updated on 28/Jun/20

$$\mathrm{all}\:\mathrm{vuibert}\:\mathrm{and}\:\mathrm{ellipces}\:\mathrm{books}\:\mathrm{are}\:\mathrm{good}… \\ $$
Answered by MJS last updated on 28/Jun/20
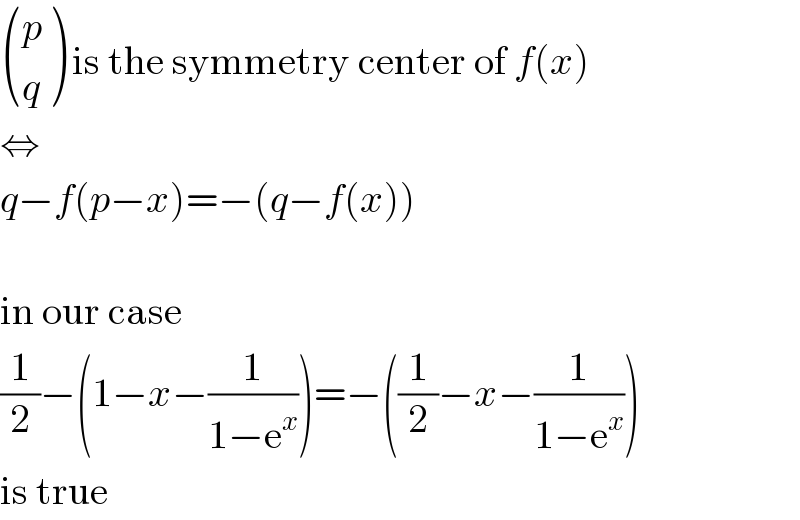
$$\begin{pmatrix}{{p}}\\{{q}}\end{pmatrix}\:\mathrm{is}\:\mathrm{the}\:\mathrm{symmetry}\:\mathrm{center}\:\mathrm{of}\:{f}\left({x}\right) \\ $$$$\Leftrightarrow \\ $$$${q}−{f}\left({p}−{x}\right)=−\left({q}−{f}\left({x}\right)\right) \\ $$$$ \\ $$$$\mathrm{in}\:\mathrm{our}\:\mathrm{case} \\ $$$$\frac{\mathrm{1}}{\mathrm{2}}−\left(\mathrm{1}−{x}−\frac{\mathrm{1}}{\mathrm{1}−\mathrm{e}^{{x}} }\right)=−\left(\frac{\mathrm{1}}{\mathrm{2}}−{x}−\frac{\mathrm{1}}{\mathrm{1}−\mathrm{e}^{{x}} }\right) \\ $$$$\mathrm{is}\:\mathrm{true} \\ $$
Commented by MJS last updated on 28/Jun/20

$$\mathrm{sorry}\:\mathrm{I}\:\mathrm{know}\:\mathrm{no}\:\mathrm{books}\:\mathrm{on}\:\mathrm{this}\:\mathrm{topic}… \\ $$
Answered by abdomathmax last updated on 28/Jun/20

$$\mathrm{I}\left(\mathrm{a},\mathrm{b}\right)\:\mathrm{is}\:\mathrm{centre}\:\mathrm{of}\:\mathrm{symetry}\:\Leftrightarrow\mathrm{f}\left(\mathrm{2a}−\mathrm{x}\right)=\mathrm{2b}−\mathrm{f}\left(\mathrm{x}\right) \\ $$$$\mathrm{I}\left(\mathrm{0},\frac{\mathrm{1}}{\mathrm{2}}\right)\:\mathrm{we}\:\mathrm{must}\:\mathrm{prove}\:\mathrm{f}\left(\mathrm{2}×\mathrm{0}−\mathrm{x}\right)\:=\mathrm{2}×\frac{\mathrm{1}}{\mathrm{2}}−\mathrm{f}\left(\mathrm{x}\right)\:\Rightarrow \\ $$$$\mathrm{f}\left(−\mathrm{x}\right)\:=\mathrm{1}−\mathrm{f}\left(\mathrm{x}\right)\:\mathrm{we}\:\mathrm{have} \\ $$$$\mathrm{f}\left(−\mathrm{x}\right)\:=−\mathrm{x}\:+\frac{\mathrm{1}}{\mathrm{1}−\mathrm{e}^{−\mathrm{x}} }\:=−\mathrm{x}\:+\frac{\mathrm{e}^{\mathrm{x}} }{\mathrm{e}^{\mathrm{x}} −\mathrm{1}}\:=\frac{\left(\mathrm{1}−\mathrm{x}\right)\mathrm{e}^{\mathrm{x}} +\mathrm{x}}{\mathrm{e}^{\mathrm{x}} −\mathrm{1}} \\ $$$$\mathrm{1}−\mathrm{f}\left(\mathrm{x}\right)\:=\mathrm{1}−\mathrm{x}−\frac{\mathrm{1}}{\mathrm{1}−\mathrm{e}^{\mathrm{x}} }\:=\frac{\mathrm{1}−\mathrm{e}^{\mathrm{x}} −\mathrm{x}+\mathrm{xe}^{\mathrm{x}} −\mathrm{1}}{\mathrm{1}−\mathrm{e}^{\mathrm{x}} } \\ $$$$=\frac{−\mathrm{x}\:+\left({x}−\mathrm{1}\right){e}^{{x}} }{\mathrm{1}−{e}^{{x}} }\:=\frac{{x}+\left(\mathrm{1}−{x}\right){e}^{{x}} }{{e}^{{x}} −\mathrm{1}} \\ $$$${tbe}\:{equality}\:{is}\:{proved}.. \\ $$
Commented by Rio Michael last updated on 28/Jun/20

$$\mathrm{thank}\:\mathrm{you}\:\mathrm{all} \\ $$
