Menu Close

Find-limit-lim-x-x-x-2-1-x-and-lim-x-x-x-2-1-x-

Tinku Tara 0 Comments
Pin




Question Number 100920 by ajfour last updated on 29/Jun/20
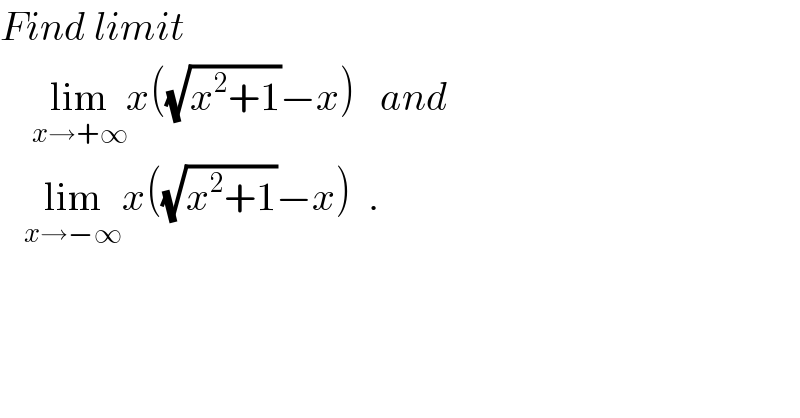
Find limit lim_(x→+∞) x((√(x^2 +1))−x) and lim_(x→−∞) x((√(x^2 +1))−x) .
$${Find}\:{limit} \\ $$$$\:\:\:\:\underset{{x}\rightarrow+\infty} {\mathrm{lim}}{x}\left(\sqrt{{x}^{\mathrm{2}} +\mathrm{1}}−{x}\right)\:\:\:{and} \\ $$$$\:\:\:\underset{{x}\rightarrow−\infty} {\mathrm{lim}}{x}\left(\sqrt{{x}^{\mathrm{2}} +\mathrm{1}}−{x}\right)\:\:. \\ $$
Commented by bramlex last updated on 29/Jun/20
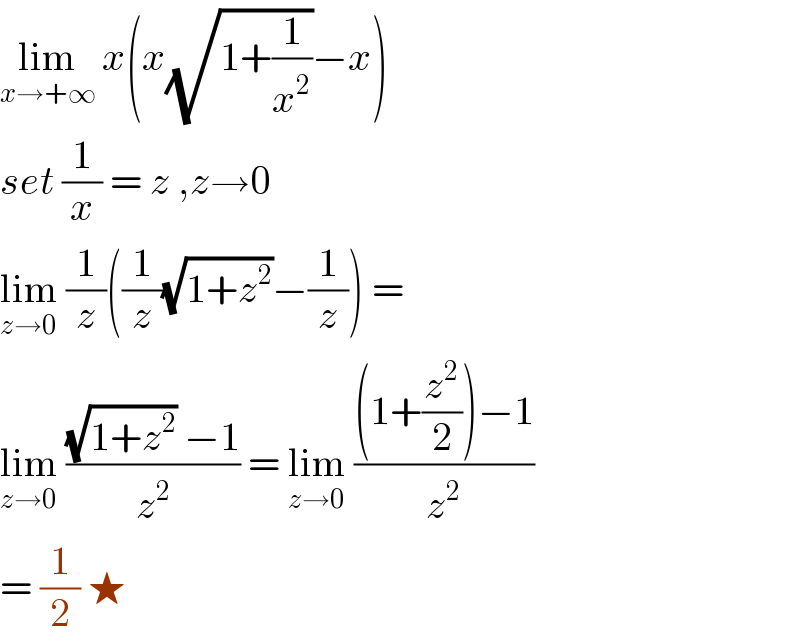
lim_(x→+∞) x(x(√(1+(1/x^2 )))−x) set (1/x) = z ,z→0 lim_(z→0) (1/z)((1/z)(√(1+z^2 ))−(1/z)) = lim_(z→0) (((√(1+z^2 )) −1)/z^2 ) = lim_(z→0) (((1+(z^2 /2))−1)/z^2 ) = (1/2) ★
$$\underset{{x}\rightarrow+\infty} {\mathrm{lim}}\:{x}\left({x}\sqrt{\mathrm{1}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }}−{x}\right)\: \\ $$$${set}\:\frac{\mathrm{1}}{{x}}\:=\:{z}\:,{z}\rightarrow\mathrm{0} \\ $$$$\underset{{z}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{1}}{{z}}\left(\frac{\mathrm{1}}{{z}}\sqrt{\mathrm{1}+{z}^{\mathrm{2}} }−\frac{\mathrm{1}}{{z}}\right)\:= \\ $$$$\underset{{z}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\sqrt{\mathrm{1}+{z}^{\mathrm{2}} }\:−\mathrm{1}}{{z}^{\mathrm{2}} }\:=\:\underset{{z}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\left(\mathrm{1}+\frac{{z}^{\mathrm{2}} }{\mathrm{2}}\right)−\mathrm{1}}{{z}^{\mathrm{2}} } \\ $$$$=\:\frac{\mathrm{1}}{\mathrm{2}}\:\bigstar \\ $$
Commented by bramlex last updated on 29/Jun/20
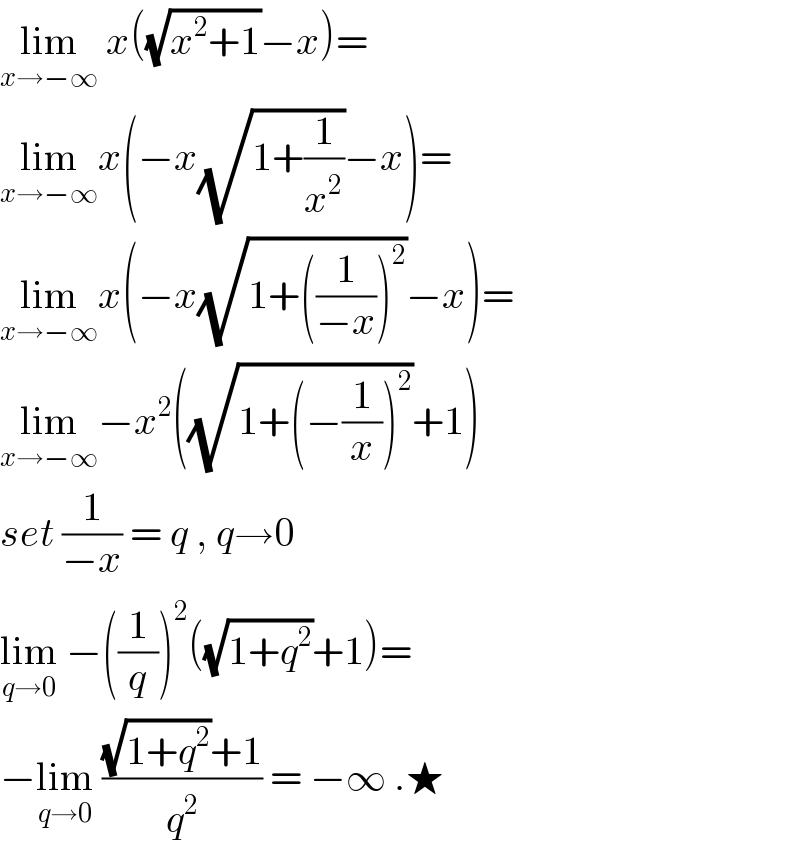
lim_(x→−∞) x((√(x^2 +1))−x)= lim_(x→−∞) x(−x(√(1+(1/x^2 )))−x)= lim_(x→−∞) x(−x(√(1+((1/(−x)))^2 ))−x)= lim_(x→−∞) −x^2 ((√(1+(−(1/x))^2 ))+1) set (1/(−x)) = q , q→0 lim_(q→0) −((1/q))^2 ((√(1+q^2 ))+1)= −lim_(q→0) (((√(1+q^2 ))+1)/q^2 ) = −∞ .★
$$\underset{{x}\rightarrow−\infty} {\mathrm{lim}}\:{x}\left(\sqrt{{x}^{\mathrm{2}} +\mathrm{1}}−{x}\right)= \\ $$$$\underset{{x}\rightarrow−\infty} {\mathrm{lim}}{x}\left(−{x}\sqrt{\mathrm{1}+\frac{\mathrm{1}}{{x}^{\mathrm{2}} }}−{x}\right)= \\ $$$$\underset{{x}\rightarrow−\infty} {\mathrm{lim}}{x}\left(−{x}\sqrt{\mathrm{1}+\left(\frac{\mathrm{1}}{−{x}}\right)^{\mathrm{2}} }−{x}\right)= \\ $$$$\underset{{x}\rightarrow−\infty} {\mathrm{lim}}−{x}^{\mathrm{2}} \left(\sqrt{\mathrm{1}+\left(−\frac{\mathrm{1}}{{x}}\right)^{\mathrm{2}} }+\mathrm{1}\right) \\ $$$${set}\:\frac{\mathrm{1}}{−{x}}\:=\:{q}\:,\:{q}\rightarrow\mathrm{0} \\ $$$$\underset{{q}\rightarrow\mathrm{0}} {\mathrm{lim}}\:−\left(\frac{\mathrm{1}}{{q}}\right)^{\mathrm{2}} \left(\sqrt{\mathrm{1}+{q}^{\mathrm{2}} }+\mathrm{1}\right)= \\ $$$$−\underset{{q}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\sqrt{\mathrm{1}+{q}^{\mathrm{2}} }+\mathrm{1}}{{q}^{\mathrm{2}} }\:=\:−\infty\:.\bigstar \\ $$
Answered by mathmax by abdo last updated on 29/Jun/20
for x>0 we have x((√(x^2 +1))−x) =x(x(√(1+(1/x^2 )))−x)∼x(x(1+(1/(2x^2 )))−x) =x( (1/(2x))) =(1/2) ⇒lim_(x→+∞) x((√(1+x^2 ))−x) =(1/2) for x<0 we have x((√(x^2 +1))−x) =x{ −x(√(1+(1/x^2 )))−x} ∼x{−x(1+(1/(2x^2 )))−x} =x{−2x−(1/(2x))} =−2x^2 −(x/2) →−∞
$$\mathrm{for}\:\mathrm{x}>\mathrm{0}\:\mathrm{we}\:\mathrm{have}\:\mathrm{x}\left(\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}−\mathrm{x}\right)\:=\mathrm{x}\left(\mathrm{x}\sqrt{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }}−\mathrm{x}\right)\sim\mathrm{x}\left(\mathrm{x}\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2x}^{\mathrm{2}} }\right)−\mathrm{x}\right) \\ $$$$=\mathrm{x}\left(\:\frac{\mathrm{1}}{\mathrm{2x}}\right)\:=\frac{\mathrm{1}}{\mathrm{2}}\:\Rightarrow\mathrm{lim}_{\mathrm{x}\rightarrow+\infty} \:\mathrm{x}\left(\sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }−\mathrm{x}\right)\:=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\mathrm{for}\:\mathrm{x}<\mathrm{0}\:\:\mathrm{we}\:\mathrm{have}\:\mathrm{x}\left(\sqrt{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}−\mathrm{x}\right)\:=\mathrm{x}\left\{\:−\mathrm{x}\sqrt{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }}−\mathrm{x}\right\} \\ $$$$\sim\mathrm{x}\left\{−\mathrm{x}\left(\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2x}^{\mathrm{2}} }\right)−\mathrm{x}\right\}\:=\mathrm{x}\left\{−\mathrm{2x}−\frac{\mathrm{1}}{\mathrm{2x}}\right\}\:=−\mathrm{2x}^{\mathrm{2}} −\frac{\mathrm{x}}{\mathrm{2}}\:\rightarrow−\infty \\ $$
Commented by ajfour last updated on 29/Jun/20
correct Sir, i mean lim_(x→+∞) f(x)=(1/2) and lim_(x→−∞) f(x)=−∞ . Thanks.
$${correct}\:{Sir},\:\:{i}\:{mean}\:\underset{{x}\rightarrow+\infty} {\mathrm{lim}}{f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{2}} \\ $$$${and}\:\:\underset{{x}\rightarrow−\infty} {\mathrm{lim}}{f}\left({x}\right)=−\infty\:.\:{Thanks}. \\ $$
Commented by mathmax by abdo last updated on 29/Jun/20
you are welcome
$$\mathrm{you}\:\mathrm{are}\:\mathrm{welcome} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *