Menu Close

Question-166917

Tinku Tara 0 Comments
Pin




Question Number 166917 by ajfour last updated on 02/Mar/22
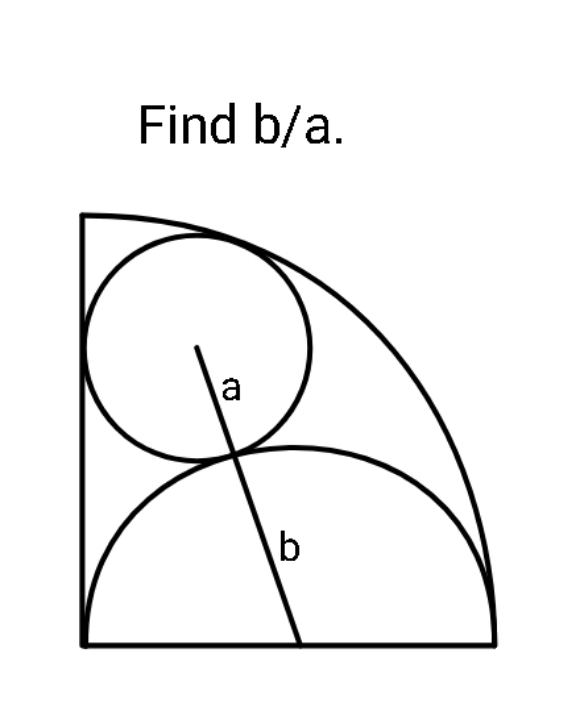
Commented by mr W last updated on 02/Mar/22
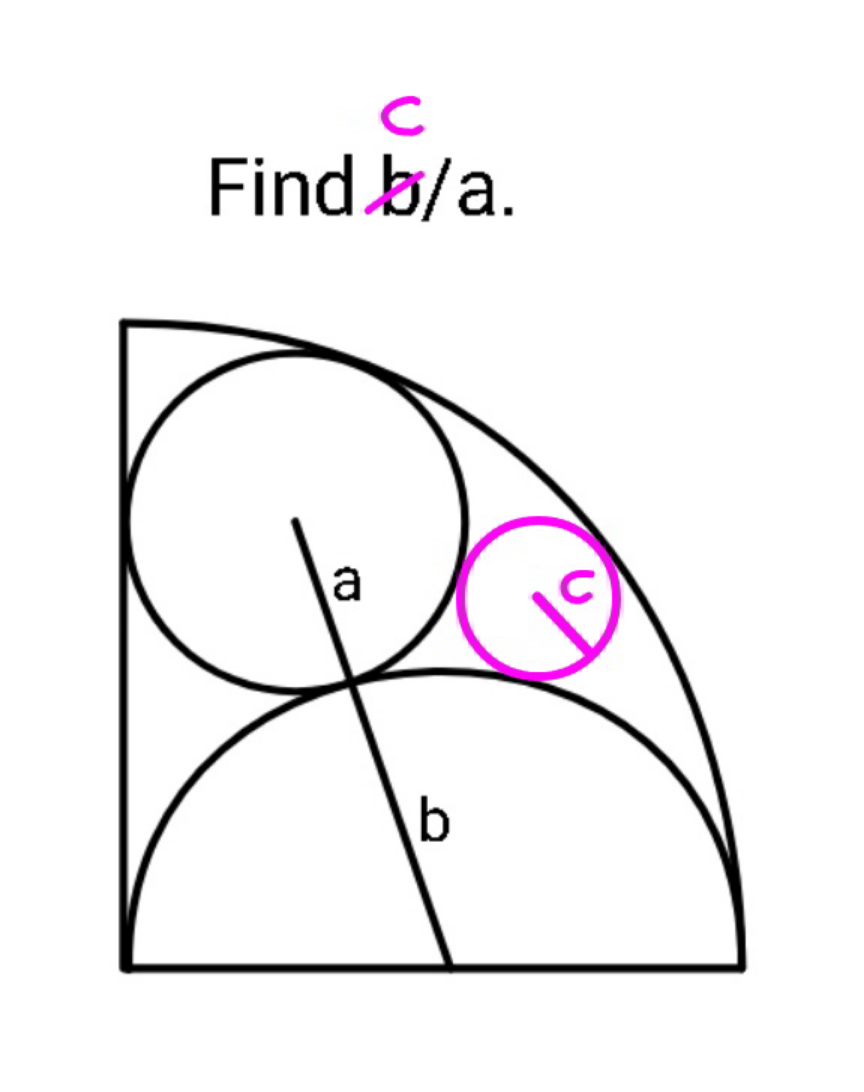
Commented by ajfour last updated on 02/Mar/22
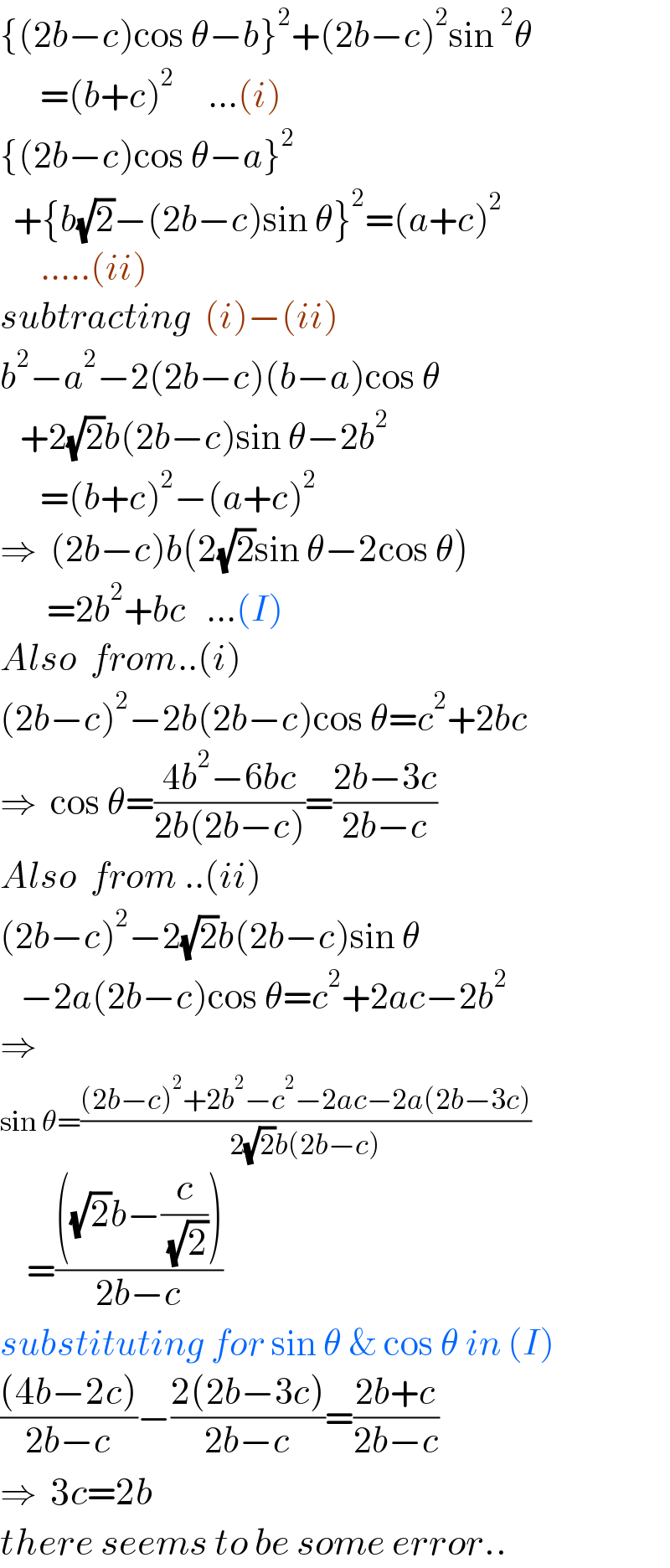
{(2b−c)cos θ−b}^2 +(2b−c)^2 sin^2 θ =(b+c)^2 ...(i) {(2b−c)cos θ−a}^2 +{b(√2)−(2b−c)sin θ}^2 =(a+c)^2 .....(ii) subtracting (i)−(ii) b^2 −a^2 −2(2b−c)(b−a)cos θ +2(√2)b(2b−c)sin θ−2b^2 =(b+c)^2 −(a+c)^2 ⇒ (2b−c)b(2(√2)sin θ−2cos θ) =2b^2 +bc ...(I) Also from..(i) (2b−c)^2 −2b(2b−c)cos θ=c^2 +2bc ⇒ cos θ=((4b^2 −6bc)/(2b(2b−c)))=((2b−3c)/(2b−c)) Also from ..(ii) (2b−c)^2 −2(√2)b(2b−c)sin θ −2a(2b−c)cos θ=c^2 +2ac−2b^2 ⇒ sin θ=(((2b−c)^2 +2b^2 −c^2 −2ac−2a(2b−3c))/(2(√2)b(2b−c))) =((((√2)b−(c/( (√2)))))/(2b−c)) substituting for sin θ & cos θ in (I) (((4b−2c))/(2b−c))−((2(2b−3c))/(2b−c))=((2b+c)/(2b−c)) ⇒ 3c=2b there seems to be some error..
$$\left\{\left(\mathrm{2}{b}−{c}\right)\mathrm{cos}\:\theta−{b}\right\}^{\mathrm{2}} +\left(\mathrm{2}{b}−{c}\right)^{\mathrm{2}} \mathrm{sin}\:^{\mathrm{2}} \theta \\ $$$$\:\:\:\:\:\:=\left({b}+{c}\right)^{\mathrm{2}} \:\:\:\:\:…\left({i}\right) \\ $$$$\left\{\left(\mathrm{2}{b}−{c}\right)\mathrm{cos}\:\theta−{a}\right\}^{\mathrm{2}} \\ $$$$\:\:+\left\{{b}\sqrt{\mathrm{2}}−\left(\mathrm{2}{b}−{c}\right)\mathrm{sin}\:\theta\right\}^{\mathrm{2}} =\left({a}+{c}\right)^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:…..\left({ii}\right) \\ $$$${subtracting}\:\:\left({i}\right)−\left({ii}\right) \\ $$$${b}^{\mathrm{2}} −{a}^{\mathrm{2}} −\mathrm{2}\left(\mathrm{2}{b}−{c}\right)\left({b}−{a}\right)\mathrm{cos}\:\theta \\ $$$$\:\:\:+\mathrm{2}\sqrt{\mathrm{2}}{b}\left(\mathrm{2}{b}−{c}\right)\mathrm{sin}\:\theta−\mathrm{2}{b}^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:=\left({b}+{c}\right)^{\mathrm{2}} −\left({a}+{c}\right)^{\mathrm{2}} \\ $$$$\Rightarrow\:\:\left(\mathrm{2}{b}−{c}\right){b}\left(\mathrm{2}\sqrt{\mathrm{2}}\mathrm{sin}\:\theta−\mathrm{2cos}\:\theta\right) \\ $$$$\:\:\:\:\:\:\:=\mathrm{2}{b}^{\mathrm{2}} +{bc}\:\:\:…\left({I}\right) \\ $$$${Also}\:\:{from}..\left({i}\right) \\ $$$$\left(\mathrm{2}{b}−{c}\right)^{\mathrm{2}} −\mathrm{2}{b}\left(\mathrm{2}{b}−{c}\right)\mathrm{cos}\:\theta={c}^{\mathrm{2}} +\mathrm{2}{bc} \\ $$$$\Rightarrow\:\:\mathrm{cos}\:\theta=\frac{\mathrm{4}{b}^{\mathrm{2}} −\mathrm{6}{bc}}{\mathrm{2}{b}\left(\mathrm{2}{b}−{c}\right)}=\frac{\mathrm{2}{b}−\mathrm{3}{c}}{\mathrm{2}{b}−{c}} \\ $$$${Also}\:\:{from}\:..\left({ii}\right) \\ $$$$\left(\mathrm{2}{b}−{c}\right)^{\mathrm{2}} −\mathrm{2}\sqrt{\mathrm{2}}{b}\left(\mathrm{2}{b}−{c}\right)\mathrm{sin}\:\theta \\ $$$$\:\:\:−\mathrm{2}{a}\left(\mathrm{2}{b}−{c}\right)\mathrm{cos}\:\theta={c}^{\mathrm{2}} +\mathrm{2}{ac}−\mathrm{2}{b}^{\mathrm{2}} \\ $$$$\Rightarrow\:\: \\ $$$$\mathrm{sin}\:\theta=\frac{\left(\mathrm{2}{b}−{c}\right)^{\mathrm{2}} +\mathrm{2}{b}^{\mathrm{2}} −{c}^{\mathrm{2}} −\mathrm{2}{ac}−\mathrm{2}{a}\left(\mathrm{2}{b}−\mathrm{3}{c}\right)}{\mathrm{2}\sqrt{\mathrm{2}}{b}\left(\mathrm{2}{b}−{c}\right)} \\ $$$$\:\:\:\:=\frac{\left(\sqrt{\mathrm{2}}{b}−\frac{{c}}{\:\sqrt{\mathrm{2}}}\right)}{\mathrm{2}{b}−{c}} \\ $$$${substituting}\:{for}\:\mathrm{sin}\:\theta\:\&\:\mathrm{cos}\:\theta\:{in}\:\left({I}\right) \\ $$$$\frac{\left(\mathrm{4}{b}−\mathrm{2}{c}\right)}{\mathrm{2}{b}−{c}}−\frac{\mathrm{2}\left(\mathrm{2}{b}−\mathrm{3}{c}\right)}{\mathrm{2}{b}−{c}}=\frac{\mathrm{2}{b}+{c}}{\mathrm{2}{b}−{c}} \\ $$$$\Rightarrow\:\:\mathrm{3}{c}=\mathrm{2}{b} \\ $$$${there}\:{seems}\:{to}\:{be}\:{some}\:{error}.. \\ $$
Commented by mr W last updated on 02/Mar/22
something seems wrong indeed sir. i got in an other way (see Q77681): ((1/a)+(1/(2a))+(1/c)−(1/(4a)))^2 =2((1/a^2 )+(1/(4a^2 ))+(1/c^2 )+(1/(16a^2 ))) 17((c/a))^2 −40((c/a))+16=0 ⇒(c/a)=((4(5−2(√2)))/(17))≈0.511
$${something}\:{seems}\:{wrong}\:{indeed}\:{sir}. \\ $$$${i}\:{got}\:{in}\:{an}\:{other}\:{way}\:\left({see}\:{Q}\mathrm{77681}\right): \\ $$$$\left(\frac{\mathrm{1}}{{a}}+\frac{\mathrm{1}}{\mathrm{2}{a}}+\frac{\mathrm{1}}{{c}}−\frac{\mathrm{1}}{\mathrm{4}{a}}\right)^{\mathrm{2}} =\mathrm{2}\left(\frac{\mathrm{1}}{{a}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{4}{a}^{\mathrm{2}} }+\frac{\mathrm{1}}{{c}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{16}{a}^{\mathrm{2}} }\right) \\ $$$$\mathrm{17}\left(\frac{{c}}{{a}}\right)^{\mathrm{2}} −\mathrm{40}\left(\frac{{c}}{{a}}\right)+\mathrm{16}=\mathrm{0} \\ $$$$\Rightarrow\frac{{c}}{{a}}=\frac{\mathrm{4}\left(\mathrm{5}−\mathrm{2}\sqrt{\mathrm{2}}\right)}{\mathrm{17}}\approx\mathrm{0}.\mathrm{511} \\ $$
Commented by Tawa11 last updated on 03/Mar/22
Great sir
$$\mathrm{Great}\:\mathrm{sir} \\ $$
Answered by ajfour last updated on 02/Mar/22
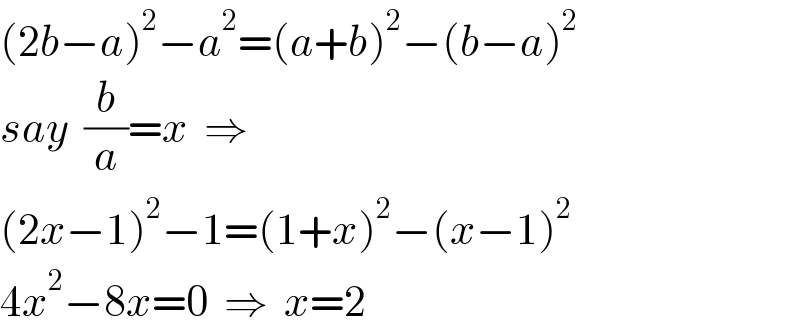
(2b−a)^2 −a^2 =(a+b)^2 −(b−a)^2 say (b/a)=x ⇒ (2x−1)^2 −1=(1+x)^2 −(x−1)^2 4x^2 −8x=0 ⇒ x=2
$$\left(\mathrm{2}{b}−{a}\right)^{\mathrm{2}} −{a}^{\mathrm{2}} =\left({a}+{b}\right)^{\mathrm{2}} −\left({b}−{a}\right)^{\mathrm{2}} \\ $$$${say}\:\:\frac{{b}}{{a}}={x}\:\:\Rightarrow \\ $$$$\left(\mathrm{2}{x}−\mathrm{1}\right)^{\mathrm{2}} −\mathrm{1}=\left(\mathrm{1}+{x}\right)^{\mathrm{2}} −\left({x}−\mathrm{1}\right)^{\mathrm{2}} \\ $$$$\mathrm{4}{x}^{\mathrm{2}} −\mathrm{8}{x}=\mathrm{0}\:\:\Rightarrow\:\:{x}=\mathrm{2} \\ $$
Answered by cherokeesay last updated on 02/Mar/22
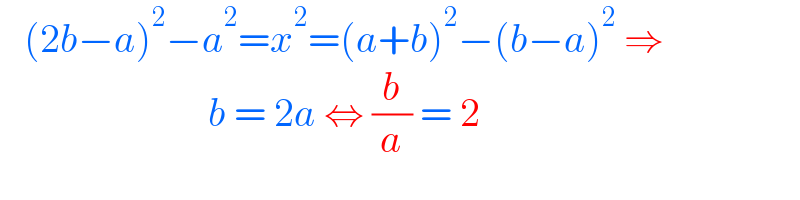
(2b−a)^2 −a^2 =x^2 =(a+b)^2 −(b−a)^2 ⇒ b = 2a ⇔ (b/a) = 2
$$\:\:\:\left(\mathrm{2}{b}−{a}\right)^{\mathrm{2}} −{a}^{\mathrm{2}} ={x}^{\mathrm{2}} =\left({a}+{b}\right)^{\mathrm{2}} −\left({b}−{a}\right)^{\mathrm{2}} \:\Rightarrow \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{b}\:=\:\mathrm{2}{a}\:\Leftrightarrow\:\frac{{b}}{{a}}\:=\:\mathrm{2} \\ $$

Pin

Leave a Reply

Your email address will not be published. Required fields are marked *