Question-36024

Question Number 36024 by bshahid010@gmail.com last updated on 27/May/18

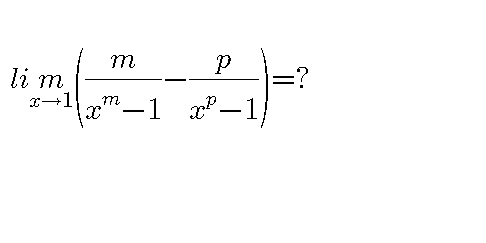
Commented by abdo mathsup 649 cc last updated on 27/May/18

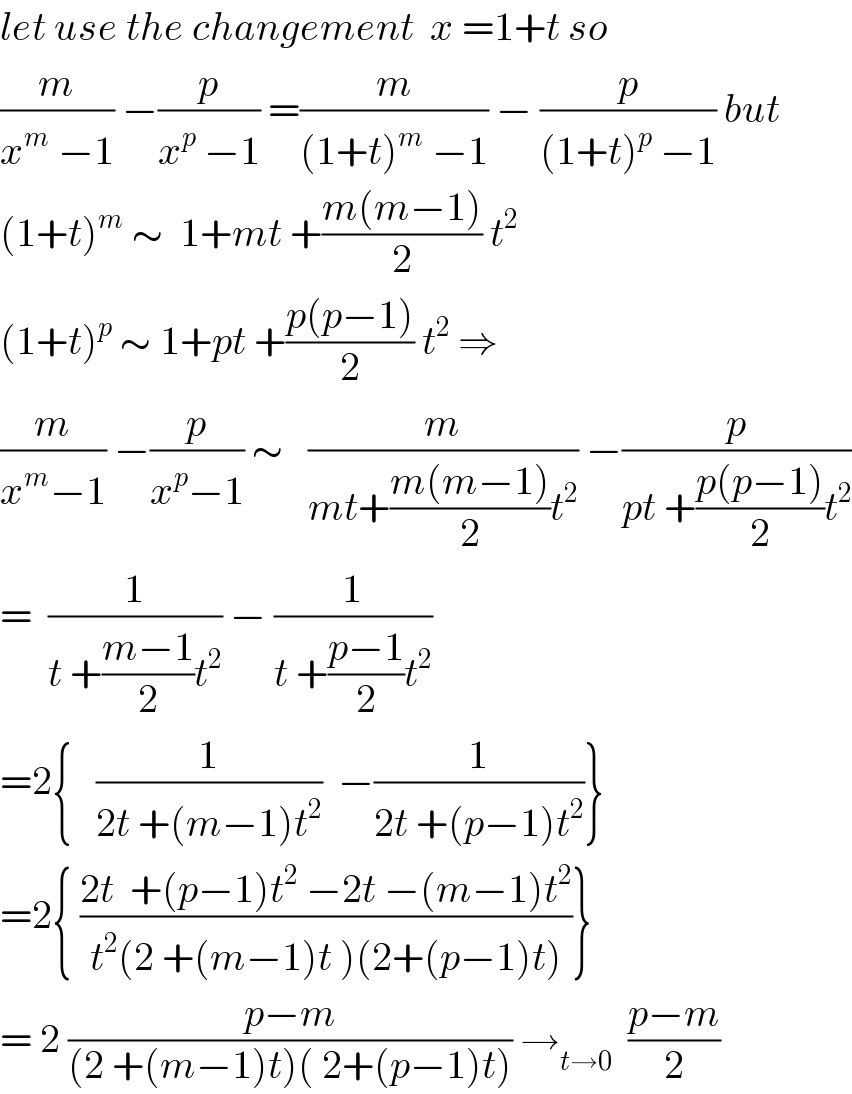
let use the changement x =1+t so (m/(x^m −1)) −(p/(x^p −1)) =(m/((1+t)^m −1)) − (p/((1+t)^p −1)) but (1+t)^m ∼ 1+mt +((m(m−1))/2) t^2 (1+t)^p ∼ 1+pt +((p(p−1))/2) t^2 ⇒ (m/(x^m −1)) −(p/(x^p −1)) ∼ (m/(mt+((m(m−1))/2)t^2 )) −(p/(pt +((p(p−1))/2)t^2 )) = (1/(t +((m−1)/2)t^2 )) − (1/(t +((p−1)/2)t^2 )) =2{ (1/(2t +(m−1)t^2 )) −(1/(2t +(p−1)t^2 ))} =2{ ((2t +(p−1)t^2 −2t −(m−1)t^2 )/(t^2 (2 +(m−1)t )(2+(p−1)t)))} = 2 ((p−m)/((2 +(m−1)t)( 2+(p−1)t))) →_(t→0) ((p−m)/2)

$${let}\:{use}\:{the}\:{changement}\:\:{x}\:=\mathrm{1}+{t}\:{so} \\ $$$$\frac{{m}}{{x}^{{m}} \:−\mathrm{1}}\:−\frac{{p}}{{x}^{{p}} \:−\mathrm{1}}\:=\frac{{m}}{\left(\mathrm{1}+{t}\right)^{{m}} \:−\mathrm{1}}\:−\:\frac{{p}}{\left(\mathrm{1}+{t}\right)^{{p}} \:−\mathrm{1}}\:{but} \\ $$$$\left(\mathrm{1}+{t}\right)^{{m}} \:\sim\:\:\mathrm{1}+{mt}\:+\frac{{m}\left({m}−\mathrm{1}\right)}{\mathrm{2}}\:{t}^{\mathrm{2}} \\ $$$$\left(\mathrm{1}+{t}\right)^{{p}} \:\sim\:\mathrm{1}+{pt}\:+\frac{{p}\left({p}−\mathrm{1}\right)}{\mathrm{2}}\:{t}^{\mathrm{2}} \:\Rightarrow \\ $$$$\frac{{m}}{{x}^{{m}} −\mathrm{1}}\:−\frac{{p}}{{x}^{{p}} −\mathrm{1}}\:\sim\:\:\:\frac{{m}}{{mt}+\frac{{m}\left({m}−\mathrm{1}\right)}{\mathrm{2}}{t}^{\mathrm{2}} }\:−\frac{{p}}{{pt}\:+\frac{{p}\left({p}−\mathrm{1}\right)}{\mathrm{2}}{t}^{\mathrm{2}} } \\ $$$$=\:\:\frac{\mathrm{1}}{{t}\:+\frac{{m}−\mathrm{1}}{\mathrm{2}}{t}^{\mathrm{2}} }\:−\:\frac{\mathrm{1}}{{t}\:+\frac{{p}−\mathrm{1}}{\mathrm{2}}{t}^{\mathrm{2}} } \\ $$$$=\mathrm{2}\left\{\:\:\:\frac{\mathrm{1}}{\mathrm{2}{t}\:+\left({m}−\mathrm{1}\right){t}^{\mathrm{2}} }\:\:−\frac{\mathrm{1}}{\mathrm{2}{t}\:+\left({p}−\mathrm{1}\right){t}^{\mathrm{2}} }\right\} \\ $$$$=\mathrm{2}\left\{\:\frac{\mathrm{2}{t}\:\:+\left({p}−\mathrm{1}\right){t}^{\mathrm{2}} \:−\mathrm{2}{t}\:−\left({m}−\mathrm{1}\right){t}^{\mathrm{2}} }{{t}^{\mathrm{2}} \left(\mathrm{2}\:+\left({m}−\mathrm{1}\right){t}\:\right)\left(\mathrm{2}+\left({p}−\mathrm{1}\right){t}\right)}\right\} \\ $$$$=\:\mathrm{2}\:\frac{{p}−{m}}{\left(\mathrm{2}\:+\left({m}−\mathrm{1}\right){t}\right)\left(\:\mathrm{2}+\left({p}−\mathrm{1}\right){t}\right)}\:\rightarrow_{{t}\rightarrow\mathrm{0}} \:\:\frac{{p}−{m}}{\mathrm{2}} \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 27/May/18

$${excellent}… \\ $$

Answered by ajfour last updated on 27/May/18

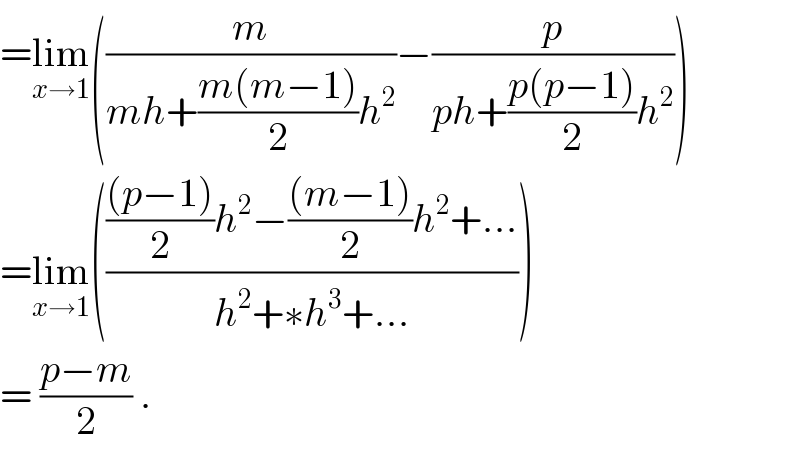
=lim_(x→1) ((m/(mh+((m(m−1))/2)h^2 ))−(p/(ph+((p(p−1))/2)h^2 ))) =lim_(x→1) ((((((p−1))/2)h^2 −(((m−1))/2)h^2 +...)/(h^2 +∗h^3 +...))) = ((p−m)/2) .

$$=\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\left(\frac{{m}}{{mh}+\frac{{m}\left({m}−\mathrm{1}\right)}{\mathrm{2}}{h}^{\mathrm{2}} }−\frac{{p}}{{ph}+\frac{{p}\left({p}−\mathrm{1}\right)}{\mathrm{2}}{h}^{\mathrm{2}} }\right) \\ $$$$=\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\left(\frac{\frac{\left({p}−\mathrm{1}\right)}{\mathrm{2}}{h}^{\mathrm{2}} −\frac{\left({m}−\mathrm{1}\right)}{\mathrm{2}}{h}^{\mathrm{2}} +…}{{h}^{\mathrm{2}} +\ast{h}^{\mathrm{3}} +…}\right) \\ $$$$=\:\frac{{p}−{m}}{\mathrm{2}}\:. \\ $$

Commented by tanmay.chaudhury50@gmail.com last updated on 27/May/18

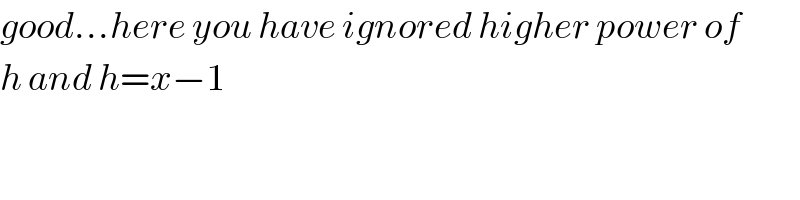
good...here you have ignored higher power of h and h=x−1

$${good}…{here}\:{you}\:{have}\:{ignored}\:{higher}\:{power}\:{of} \\ $$$${h}\:{and}\:{h}={x}−\mathrm{1} \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply