Question Number 101742 by bemath last updated on 04/Jul/20
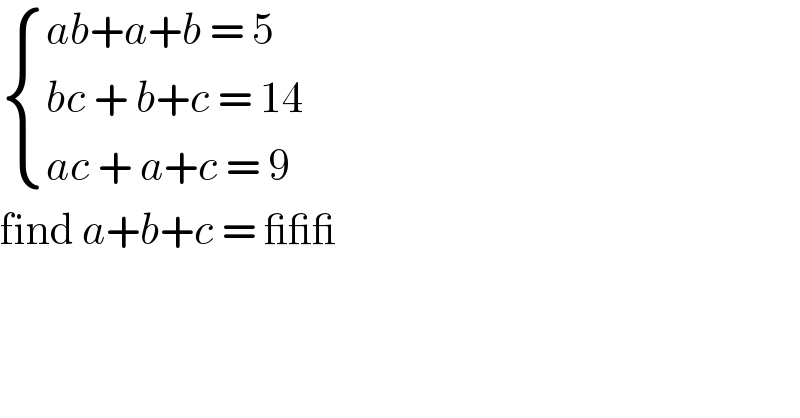
$$\begin{cases}{{ab}+{a}+{b}\:=\:\mathrm{5}}\\{{bc}\:+\:{b}+{c}\:=\:\mathrm{14}}\\{{ac}\:+\:{a}+{c}\:=\:\mathrm{9}}\end{cases} \\ $$$$\mathrm{find}\:{a}+{b}+{c}\:=\:\_\_\_ \\ $$
Answered by bramlex last updated on 04/Jul/20
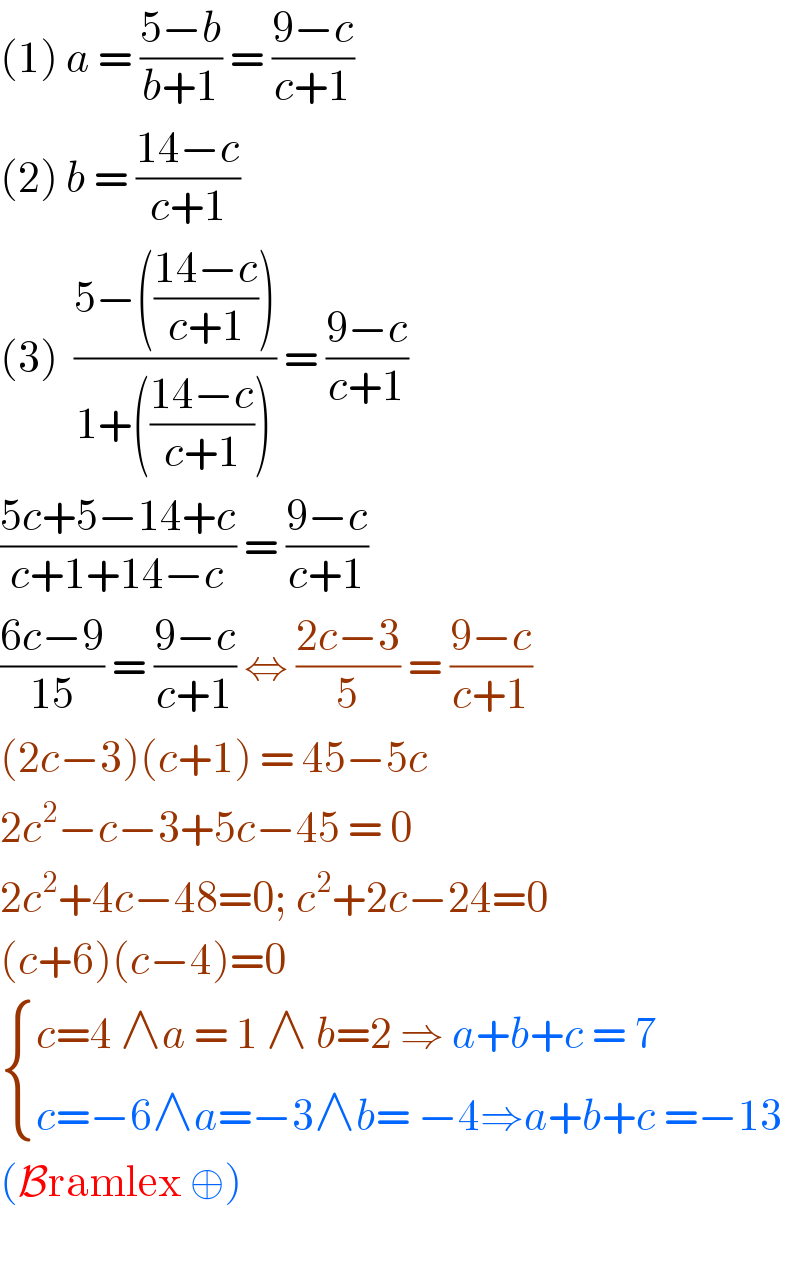
$$\left(\mathrm{1}\right)\:{a}\:=\:\frac{\mathrm{5}−{b}}{{b}+\mathrm{1}}\:=\:\frac{\mathrm{9}−{c}}{{c}+\mathrm{1}} \\ $$$$\left(\mathrm{2}\right)\:{b}\:=\:\frac{\mathrm{14}−{c}}{{c}+\mathrm{1}}\: \\ $$$$\left(\mathrm{3}\right)\:\:\frac{\mathrm{5}−\left(\frac{\mathrm{14}−{c}}{{c}+\mathrm{1}}\right)}{\mathrm{1}+\left(\frac{\mathrm{14}−{c}}{{c}+\mathrm{1}}\right)}\:=\:\frac{\mathrm{9}−{c}}{{c}+\mathrm{1}} \\ $$$$\frac{\mathrm{5}{c}+\mathrm{5}−\mathrm{14}+{c}}{{c}+\mathrm{1}+\mathrm{14}−{c}}\:=\:\frac{\mathrm{9}−{c}}{{c}+\mathrm{1}} \\ $$$$\frac{\mathrm{6}{c}−\mathrm{9}}{\mathrm{15}}\:=\:\frac{\mathrm{9}−{c}}{{c}+\mathrm{1}}\:\Leftrightarrow\:\frac{\mathrm{2}{c}−\mathrm{3}}{\mathrm{5}}\:=\:\frac{\mathrm{9}−{c}}{{c}+\mathrm{1}} \\ $$$$\left(\mathrm{2}{c}−\mathrm{3}\right)\left({c}+\mathrm{1}\right)\:=\:\mathrm{45}−\mathrm{5}{c} \\ $$$$\mathrm{2}{c}^{\mathrm{2}} −{c}−\mathrm{3}+\mathrm{5}{c}−\mathrm{45}\:=\:\mathrm{0} \\ $$$$\mathrm{2}{c}^{\mathrm{2}} +\mathrm{4}{c}−\mathrm{48}=\mathrm{0};\:{c}^{\mathrm{2}} +\mathrm{2}{c}−\mathrm{24}=\mathrm{0} \\ $$$$\left({c}+\mathrm{6}\right)\left({c}−\mathrm{4}\right)=\mathrm{0} \\ $$$$\begin{cases}{{c}=\mathrm{4}\:\wedge{a}\:=\:\mathrm{1}\:\wedge\:{b}=\mathrm{2}\:\Rightarrow\:{a}+{b}+{c}\:=\:\mathrm{7}}\\{{c}=−\mathrm{6}\wedge{a}=−\mathrm{3}\wedge{b}=\:−\mathrm{4}\Rightarrow{a}+{b}+{c}\:=−\mathrm{13}}\end{cases} \\ $$$$\left(\mathcal{B}\mathrm{ramlex}\:\oplus\right)\: \\ $$$$ \\ $$
Answered by nimnim last updated on 04/Jul/20

$$\mathrm{ab}+\mathrm{a}+\mathrm{b}+\mathrm{1}=\mathrm{6}\Rightarrow\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)=\mathrm{6}………\left(\mathrm{1}\right) \\ $$$$\mathrm{bc}+\mathrm{b}+\mathrm{c}+\mathrm{1}=\mathrm{15}\Rightarrow\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)=\mathrm{15}…….\left(\mathrm{2}\right) \\ $$$$\mathrm{ac}+\mathrm{a}+\mathrm{c}+\mathrm{1}=\mathrm{10}\Rightarrow\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)=\mathrm{10}……..\left(\mathrm{3}\right) \\ $$$$\left(\mathrm{1}\right)×\left(\mathrm{2}\right)×\left(\mathrm{3}\right) \\ $$$$\left(\mathrm{a}+\mathrm{1}\right)^{\mathrm{2}} \left(\mathrm{b}+\mathrm{1}\right)^{\mathrm{2}} \left(\mathrm{c}+\mathrm{1}\right)^{\mathrm{2}} =\mathrm{900} \\ $$$$\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)=\pm\mathrm{30}……\left(\mathrm{4}\right) \\ $$$$\mathrm{Solving}\:\left(\mathrm{1}\right),\left(\mathrm{2}\right),\left(\mathrm{3}\right)\:\mathrm{and}\:\left(\mathrm{4}\right)\:\mathrm{we}\:\mathrm{get} \\ $$$$\mathrm{a}+\mathrm{1}=\pm\mathrm{5}\Rightarrow\mathrm{a}=\mathrm{4},−\mathrm{6} \\ $$$$\mathrm{b}+\mathrm{1}=\pm\mathrm{2}\Rightarrow\mathrm{b}=\mathrm{1},−\mathrm{3} \\ $$$$\mathrm{c}+\mathrm{1}=\pm\mathrm{3}\Rightarrow\mathrm{c}=\mathrm{2},−\mathrm{4} \\ $$$$\therefore\left(\mathrm{a}+\mathrm{b}+\mathrm{c}\right)=\mathrm{7},−\mathrm{13} \\ $$
Commented by Rasheed.Sindhi last updated on 04/Jul/20

$$\mathcal{C}{ool}! \\ $$
Answered by 1549442205 last updated on 04/Jul/20
![(1)⇔(a+1)(b+1)=6 (2)⇔(b+1)(c+1)=15 (3)⇔(c+1)(a+1)=10 [(a+1)(b+1)(c+1)]^2 =900⇔(a+1)(b+1)(c+1)=±30 ⇒ { ((a+1=±2)),((c+1=±5)),((b+1=±3)) :} ⇔(a,b,c)∈{(2,3,5);(−2,−3,−5)} a+b+c∈{7;−13}](https://www.tinkutara.com/question/Q101761.png)
$$\left(\mathrm{1}\right)\Leftrightarrow\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)=\mathrm{6} \\ $$$$\left(\mathrm{2}\right)\Leftrightarrow\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)=\mathrm{15} \\ $$$$\left(\mathrm{3}\right)\Leftrightarrow\left(\mathrm{c}+\mathrm{1}\right)\left(\mathrm{a}+\mathrm{1}\right)=\mathrm{10} \\ $$$$\left[\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)\right]^{\mathrm{2}} =\mathrm{900}\Leftrightarrow\left(\mathrm{a}+\mathrm{1}\right)\left(\mathrm{b}+\mathrm{1}\right)\left(\mathrm{c}+\mathrm{1}\right)=\pm\mathrm{30} \\ $$$$\Rightarrow\begin{cases}{\mathrm{a}+\mathrm{1}=\pm\mathrm{2}}\\{\mathrm{c}+\mathrm{1}=\pm\mathrm{5}}\\{\mathrm{b}+\mathrm{1}=\pm\mathrm{3}}\end{cases}\:\Leftrightarrow\left(\mathrm{a},\mathrm{b},\mathrm{c}\right)\in\left\{\left(\mathrm{2},\mathrm{3},\mathrm{5}\right);\left(−\mathrm{2},−\mathrm{3},−\mathrm{5}\right)\right\} \\ $$$$\mathrm{a}+\mathrm{b}+\mathrm{c}\in\left\{\mathrm{7};−\mathrm{13}\right\} \\ $$
