cos-x-dy-dx-y-sin-x-2x-cos-2-x-y-pi-4-15pi-2-2-32-

Question Number 101848 by bramlex last updated on 05/Jul/20

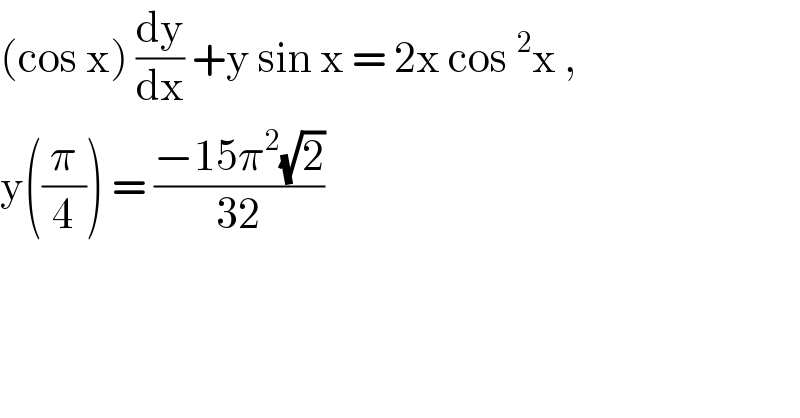
(cos x) (dy/dx) +y sin x = 2x cos^2 x , y((π/4)) = ((−15π^2 (√2))/(32))

$$\left(\mathrm{cos}\:\mathrm{x}\right)\:\frac{\mathrm{dy}}{\mathrm{dx}}\:+\mathrm{y}\:\mathrm{sin}\:\mathrm{x}\:=\:\mathrm{2x}\:\mathrm{cos}\:^{\mathrm{2}} \mathrm{x}\:, \\ $$$$\mathrm{y}\left(\frac{\pi}{\mathrm{4}}\right)\:=\:\frac{−\mathrm{15}\pi^{\mathrm{2}} \sqrt{\mathrm{2}}}{\mathrm{32}} \\ $$

Answered by john santu last updated on 05/Jul/20

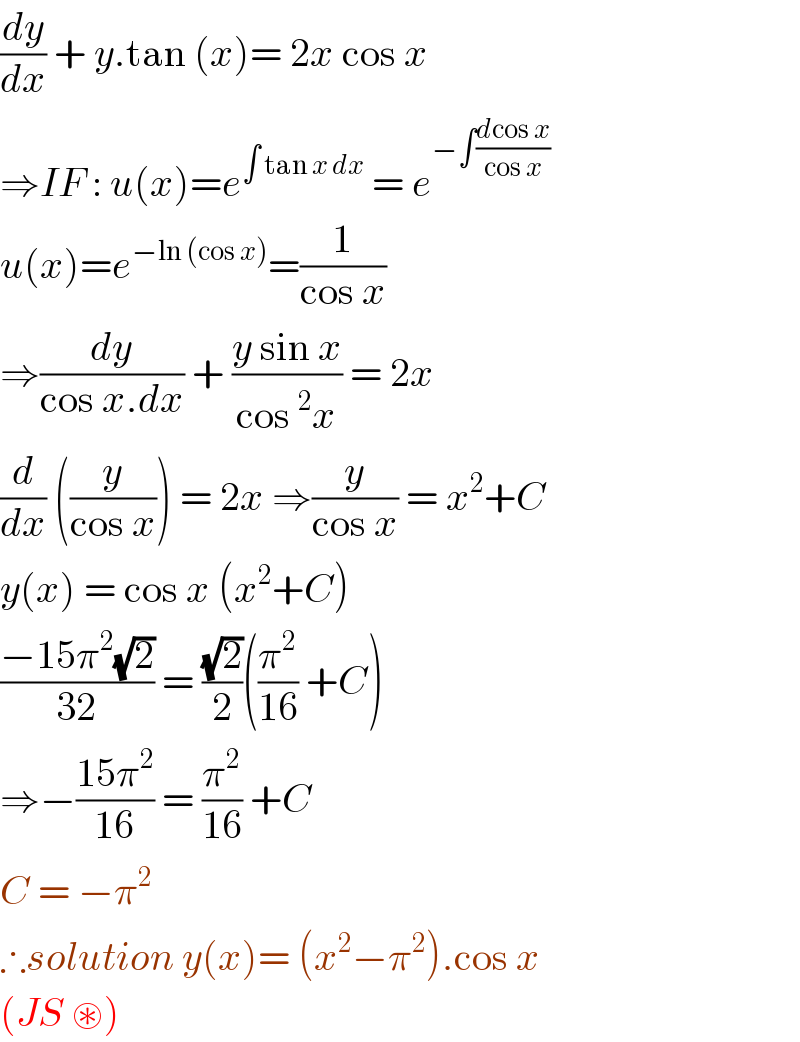
(dy/dx) + y.tan (x)= 2x cos x ⇒IF : u(x)=e^(∫ tan x dx) = e^(−∫((dcos x)/(cos x))) u(x)=e^(−ln (cos x)) =(1/(cos x)) ⇒(dy/(cos x.dx)) + ((y sin x)/(cos^2 x)) = 2x (d/dx) ((y/(cos x))) = 2x ⇒(y/(cos x)) = x^2 +C y(x) = cos x (x^2 +C) ((−15π^2 (√2))/(32)) = ((√2)/2)((π^2 /(16)) +C) ⇒−((15π^2 )/(16)) = (π^2 /(16)) +C C = −π^2 ∴solution y(x)= (x^2 −π^2 ).cos x (JS ⊛)

$$\frac{{dy}}{{dx}}\:+\:{y}.\mathrm{tan}\:\left({x}\right)=\:\mathrm{2}{x}\:\mathrm{cos}\:{x} \\ $$$$\Rightarrow{IF}\::\:{u}\left({x}\right)={e}^{\int\:\mathrm{tan}\:{x}\:{dx}} \:=\:{e}^{−\int\frac{{d}\mathrm{cos}\:{x}}{\mathrm{cos}\:{x}}} \\ $$$${u}\left({x}\right)={e}^{−\mathrm{ln}\:\left(\mathrm{cos}\:{x}\right)} =\frac{\mathrm{1}}{\mathrm{cos}\:{x}} \\ $$$$\Rightarrow\frac{{dy}}{\mathrm{cos}\:{x}.{dx}}\:+\:\frac{{y}\:\mathrm{sin}\:{x}}{\mathrm{cos}\:^{\mathrm{2}} {x}}\:=\:\mathrm{2}{x} \\ $$$$\frac{{d}}{{dx}}\:\left(\frac{{y}}{\mathrm{cos}\:{x}}\right)\:=\:\mathrm{2}{x}\:\Rightarrow\frac{{y}}{\mathrm{cos}\:{x}}\:=\:{x}^{\mathrm{2}} +{C} \\ $$$${y}\left({x}\right)\:=\:\mathrm{cos}\:{x}\:\left({x}^{\mathrm{2}} +{C}\right) \\ $$$$\frac{−\mathrm{15}\pi^{\mathrm{2}} \sqrt{\mathrm{2}}}{\mathrm{32}}\:=\:\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\left(\frac{\pi^{\mathrm{2}} }{\mathrm{16}}\:+{C}\right) \\ $$$$\Rightarrow−\frac{\mathrm{15}\pi^{\mathrm{2}} }{\mathrm{16}}\:=\:\frac{\pi^{\mathrm{2}} }{\mathrm{16}}\:+{C} \\ $$$${C}\:=\:−\pi^{\mathrm{2}} \\ $$$$\therefore{solution}\:{y}\left({x}\right)=\:\left({x}^{\mathrm{2}} −\pi^{\mathrm{2}} \right).\mathrm{cos}\:{x}\: \\ $$$$\left({JS}\:\circledast\right) \\ $$

Commented by bramlex last updated on 05/Jul/20

macho...��

Answered by mathmax by abdo last updated on 05/Jul/20

cosx y^′ +sinx y =2xcos^2 x with y((π/4)) =−((15π(√2))/(32)) he→cosx y^′ +sinx y =0 ⇒cosx y^′ =−sinx y ⇒(y^′ /y) =−((sinx)/(cosx)) ⇒ ln∣y∣ =ln(cosx)+c ⇒y =k cosx lagrange method →y^′ =k^′ cosx −ksinx e⇒k^′ cos^2 x−kcosx sinx +ksinx cosx =2xcos^2 x ⇒k^′ =2x ⇒k(x) =x^2 +λ ⇒ y(x) =(x^2 +λ)cosx y((π/4)) =−((15π(√2))/(32)) ⇒((π^2 /8) +λ)×((√2)/2) =−((15π(√2))/(32)) ⇒λ +(π^2 /8) =−((15π)/(16)) ⇒ λ =−(π^2 /8)−((15π)/(16)) =−((17π)/(16)) ⇒y(x) =(x^2 −((17π)/(16)))cosx

$$\mathrm{cosx}\:\mathrm{y}^{'} \:+\mathrm{sinx}\:\mathrm{y}\:=\mathrm{2xcos}^{\mathrm{2}} \mathrm{x}\:\:\mathrm{with}\:\mathrm{y}\left(\frac{\pi}{\mathrm{4}}\right)\:=−\frac{\mathrm{15}\pi\sqrt{\mathrm{2}}}{\mathrm{32}} \\ $$$$\mathrm{he}\rightarrow\mathrm{cosx}\:\mathrm{y}^{'} \:+\mathrm{sinx}\:\mathrm{y}\:=\mathrm{0}\:\Rightarrow\mathrm{cosx}\:\mathrm{y}^{'} \:=−\mathrm{sinx}\:\mathrm{y}\:\Rightarrow\frac{\mathrm{y}^{'} }{\mathrm{y}}\:=−\frac{\mathrm{sinx}}{\mathrm{cosx}}\:\Rightarrow \\ $$$$\mathrm{ln}\mid\mathrm{y}\mid\:=\mathrm{ln}\left(\mathrm{cosx}\right)+\mathrm{c}\:\Rightarrow\mathrm{y}\:=\mathrm{k}\:\mathrm{cosx} \\ $$$$\mathrm{lagrange}\:\mathrm{method}\:\rightarrow\mathrm{y}^{'} \:=\mathrm{k}^{'} \:\mathrm{cosx}\:−\mathrm{ksinx} \\ $$$$\mathrm{e}\Rightarrow\mathrm{k}^{'} \:\mathrm{cos}^{\mathrm{2}} \mathrm{x}−\mathrm{kcosx}\:\mathrm{sinx}\:+\mathrm{ksinx}\:\mathrm{cosx}\:=\mathrm{2xcos}^{\mathrm{2}} \mathrm{x}\:\Rightarrow\mathrm{k}^{'} \:=\mathrm{2x}\:\Rightarrow\mathrm{k}\left(\mathrm{x}\right)\:=\mathrm{x}^{\mathrm{2}} \:+\lambda\:\Rightarrow \\ $$$$\mathrm{y}\left(\mathrm{x}\right)\:=\left(\mathrm{x}^{\mathrm{2}} \:+\lambda\right)\mathrm{cosx}\: \\ $$$$\mathrm{y}\left(\frac{\pi}{\mathrm{4}}\right)\:=−\frac{\mathrm{15}\pi\sqrt{\mathrm{2}}}{\mathrm{32}}\:\Rightarrow\left(\frac{\pi^{\mathrm{2}} }{\mathrm{8}}\:+\lambda\right)×\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\:=−\frac{\mathrm{15}\pi\sqrt{\mathrm{2}}}{\mathrm{32}}\:\Rightarrow\lambda\:+\frac{\pi^{\mathrm{2}} }{\mathrm{8}}\:=−\frac{\mathrm{15}\pi}{\mathrm{16}}\:\Rightarrow \\ $$$$\lambda\:=−\frac{\pi^{\mathrm{2}} }{\mathrm{8}}−\frac{\mathrm{15}\pi}{\mathrm{16}}\:=−\frac{\mathrm{17}\pi}{\mathrm{16}}\:\Rightarrow\mathrm{y}\left(\mathrm{x}\right)\:=\left(\mathrm{x}^{\mathrm{2}} −\frac{\mathrm{17}\pi}{\mathrm{16}}\right)\mathrm{cosx} \\ $$

Commented by john santu last updated on 05/Jul/20

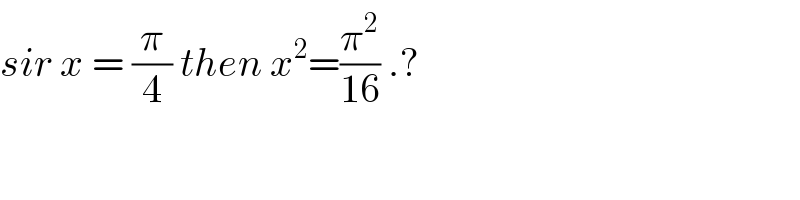
$${sir}\:{x}\:=\:\frac{\pi}{\mathrm{4}}\:{then}\:{x}^{\mathrm{2}} =\frac{\pi^{\mathrm{2}} }{\mathrm{16}}\:.? \\ $$$$ \\ $$

Commented by mathmax by abdo last updated on 05/Jul/20

sorry in the qustion y((π/4))=−((15π^2 (√2))/(32)) ⇒((π^2 /(16))+λ) ×((√2)/2) =−((15π^2 (√2))/(32)) ⇒ (π^2 /(16)) +λ =−((15π^2 )/(16)) ⇒λ =−π^2 ⇒y(x) =(x^2 −π^2 )cosx

$$\mathrm{sorry}\:\mathrm{in}\:\mathrm{the}\:\mathrm{qustion}\:\mathrm{y}\left(\frac{\pi}{\mathrm{4}}\right)=−\frac{\mathrm{15}\pi^{\mathrm{2}} \sqrt{\mathrm{2}}}{\mathrm{32}}\:\Rightarrow\left(\frac{\pi^{\mathrm{2}} }{\mathrm{16}}+\lambda\right)\:×\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\:=−\frac{\mathrm{15}\pi^{\mathrm{2}} \sqrt{\mathrm{2}}}{\mathrm{32}}\:\Rightarrow \\ $$$$\frac{\pi^{\mathrm{2}} }{\mathrm{16}}\:+\lambda\:=−\frac{\mathrm{15}\pi^{\mathrm{2}} }{\mathrm{16}}\:\Rightarrow\lambda\:=−\pi^{\mathrm{2}} \:\Rightarrow\mathrm{y}\left(\mathrm{x}\right)\:=\left(\mathrm{x}^{\mathrm{2}} −\pi^{\mathrm{2}} \right)\mathrm{cosx} \\ $$

Facebook Tweet Pin

cos-x-dy-dx-y-sin-x-2x-cos-2-x-y-pi-4-15pi-2-2-32-

Leave a Reply Cancel reply