Question Number 134670 by benjo_mathlover last updated on 06/Mar/21
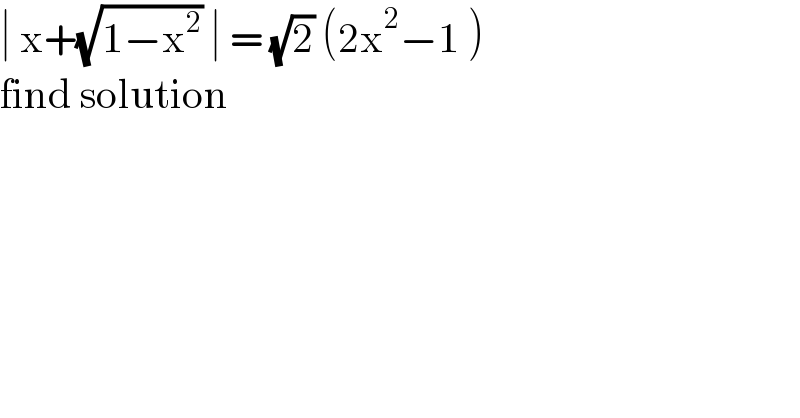
$$\mid\:\mathrm{x}+\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }\:\mid\:=\:\sqrt{\mathrm{2}}\:\left(\mathrm{2x}^{\mathrm{2}} −\mathrm{1}\:\right) \\ $$$$\mathrm{find}\:\mathrm{solution} \\ $$
Answered by EDWIN88 last updated on 06/Mar/21
![(1) 1−x^2 ≥ 0 ⇒ −1≤x≤1 (2) let x = cos t ; t∈ [ 0,π ] ∣cos t +(√(1−cos^2 t)) ∣ =(√2) (2cos^2 t−1) ∣cos t +∣sin t∣∣ = (√2) cos 2t ∣ cos t+sin t ∣ = (√2) cos 2t (cos t+sin t)^2 = 2cos^2 2t ; { ((cos 2t ≥ 0)),((0≤t≤π)) :} (i) 1+sin 2t = 2−2sin^2 2t 2sin^2 2t +sin 2t−1 = 0→ { ((sin 2t=−1)),((sin 2t=(1/2))) :} { ((t=−(π/4)+kπ)),((t=(π/(12))+kπ ; t=((5π)/(12))+kπ)) :}→ t=(π/(12)) ; t=((3π)/4) { ((x = cos (π/(12))= (√((2+(√3))/4)) = ((√(2+(√3)))/2))),((x=cos ((3π)/4)=−((√2)/2))) :} therefore the solution is determinant (((x=((√(2+(√3)))/2))),((x =−((√2)/2))))](https://www.tinkutara.com/question/Q134674.png)
$$\left(\mathrm{1}\right)\:\mathrm{1}−\mathrm{x}^{\mathrm{2}} \:\geqslant\:\mathrm{0}\:\Rightarrow\:−\mathrm{1}\leqslant\mathrm{x}\leqslant\mathrm{1} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{let}\:\mathrm{x}\:=\:\mathrm{cos}\:\mathrm{t}\:;\:\mathrm{t}\in\:\left[\:\mathrm{0},\pi\:\right] \\ $$$$\:\mid\mathrm{cos}\:\mathrm{t}\:+\sqrt{\mathrm{1}−\mathrm{cos}\:^{\mathrm{2}} \mathrm{t}}\:\mid\:=\sqrt{\mathrm{2}}\:\left(\mathrm{2cos}\:^{\mathrm{2}} \mathrm{t}−\mathrm{1}\right) \\ $$$$\:\mid\mathrm{cos}\:\mathrm{t}\:+\mid\mathrm{sin}\:\mathrm{t}\mid\mid\:=\:\sqrt{\mathrm{2}}\:\mathrm{cos}\:\mathrm{2t}\: \\ $$$$\mid\:\mathrm{cos}\:\mathrm{t}+\mathrm{sin}\:\mathrm{t}\:\mid\:=\:\sqrt{\mathrm{2}}\:\mathrm{cos}\:\mathrm{2t}\: \\ $$$$\left(\mathrm{cos}\:\mathrm{t}+\mathrm{sin}\:\mathrm{t}\right)^{\mathrm{2}} =\:\mathrm{2cos}\:^{\mathrm{2}} \mathrm{2t}\:;\:\begin{cases}{\mathrm{cos}\:\mathrm{2t}\:\geqslant\:\mathrm{0}}\\{\mathrm{0}\leqslant\mathrm{t}\leqslant\pi}\end{cases} \\ $$$$\left(\mathrm{i}\right)\:\mathrm{1}+\mathrm{sin}\:\mathrm{2t}\:=\:\mathrm{2}−\mathrm{2sin}\:^{\mathrm{2}} \mathrm{2t}\: \\ $$$$\:\mathrm{2sin}\:^{\mathrm{2}} \mathrm{2t}\:+\mathrm{sin}\:\mathrm{2t}−\mathrm{1}\:=\:\mathrm{0}\rightarrow\begin{cases}{\mathrm{sin}\:\mathrm{2t}=−\mathrm{1}}\\{\mathrm{sin}\:\mathrm{2t}=\frac{\mathrm{1}}{\mathrm{2}}}\end{cases} \\ $$$$\:\begin{cases}{\mathrm{t}=−\frac{\pi}{\mathrm{4}}+\mathrm{k}\pi}\\{\mathrm{t}=\frac{\pi}{\mathrm{12}}+\mathrm{k}\pi\:;\:\mathrm{t}=\frac{\mathrm{5}\pi}{\mathrm{12}}+\mathrm{k}\pi}\end{cases}\rightarrow\:\mathrm{t}=\frac{\pi}{\mathrm{12}}\:;\:\mathrm{t}=\frac{\mathrm{3}\pi}{\mathrm{4}} \\ $$$$\:\begin{cases}{\mathrm{x}\:=\:\mathrm{cos}\:\frac{\pi}{\mathrm{12}}=\:\sqrt{\frac{\mathrm{2}+\sqrt{\mathrm{3}}}{\mathrm{4}}}\:=\:\frac{\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}}{\mathrm{2}}}\\{\mathrm{x}=\mathrm{cos}\:\frac{\mathrm{3}\pi}{\mathrm{4}}=−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}}\end{cases} \\ $$$$\:\mathrm{therefore}\:\mathrm{the}\:\mathrm{solution}\:\mathrm{is}\:\begin{array}{|c|c|}{\mathrm{x}=\frac{\sqrt{\mathrm{2}+\sqrt{\mathrm{3}}}}{\mathrm{2}}}\\{\mathrm{x}\:=−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}}\\\hline\end{array}\: \\ $$
Commented by john_santu last updated on 06/Mar/21
![i think it should be t ∈ [ 0,2π ] then t = ((7π)/4) ∧ t = ((13π)/(12)) is the solution](https://www.tinkutara.com/question/Q134699.png)
$${i}\:{think}\:{it}\:{should}\:{be}\:{t}\:\in\:\left[\:\mathrm{0},\mathrm{2}\pi\:\right]\: \\ $$$${then}\:{t}\:=\:\frac{\mathrm{7}\pi}{\mathrm{4}}\:\wedge\:{t}\:=\:\frac{\mathrm{13}\pi}{\mathrm{12}}\:{is}\:{the}\:{solution} \\ $$
Answered by benjo_mathlover last updated on 06/Mar/21
![∣x+(√(1−x^2 )) ∣ = (√2) (2x^2 −1) ⇒x+(√(1−x^2 )) = ±(√2) (2x^2 −1) ⇒(√(1−x^2 )) = −x±(√2)(2x^2 −1) squaring ⇒1−x^2 = x^2 ±2(√2) x(2x^2 −1)+2(2x^2 −1)^2 ⇒0 = 2x^2 −1±2(√2)x(2x^2 −1)+2(2x^2 −1)^2 ⇒0=(2x^2 −1)[1±2x(√2) +2(2x^2 −1)] (i) 2x^2 =1 ; x_(1,2) =± ((√2)/2) → { ((≈0.707)),((≈−0.707)) :} (ii) 4x^2 ±2(√2) x−1= 0 → { ((x_(3,4) = ((−2(√2) ± 2(√6))/8)=((−(√2) ±(√6))/4) { ((≈0.25)),((≈−0.965)) :})),((x_(5,6) = (((√2) ±(√6))/4) → { ((≈0.965)),((≈−0.25 )) :})) :} (iii)for (√(1−x^2 )) defined on −1≤x≤1 (iv) 2x^2 −1≥0 ; x≤−((√2)/2) ∪ x≥((√2)/2) solution is {±((√2)/2); ((−(√2) ±(√6))/4) ; (((√2) ±(√6))/4) }](https://www.tinkutara.com/question/Q134677.png)
$$\mid\mathrm{x}+\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }\:\mid\:=\:\sqrt{\mathrm{2}}\:\left(\mathrm{2x}^{\mathrm{2}} −\mathrm{1}\right) \\ $$$$\Rightarrow\mathrm{x}+\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }\:=\:\pm\sqrt{\mathrm{2}}\:\left(\mathrm{2x}^{\mathrm{2}} −\mathrm{1}\right) \\ $$$$\Rightarrow\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }\:=\:−\mathrm{x}\pm\sqrt{\mathrm{2}}\left(\mathrm{2x}^{\mathrm{2}} −\mathrm{1}\right) \\ $$$$\mathrm{squaring}\: \\ $$$$\Rightarrow\mathrm{1}−\mathrm{x}^{\mathrm{2}} \:=\:\mathrm{x}^{\mathrm{2}} \pm\mathrm{2}\sqrt{\mathrm{2}}\:\mathrm{x}\left(\mathrm{2x}^{\mathrm{2}} −\mathrm{1}\right)+\mathrm{2}\left(\mathrm{2x}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{0}\:=\:\mathrm{2x}^{\mathrm{2}} −\mathrm{1}\pm\mathrm{2}\sqrt{\mathrm{2}}\mathrm{x}\left(\mathrm{2x}^{\mathrm{2}} −\mathrm{1}\right)+\mathrm{2}\left(\mathrm{2x}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{2}} \\ $$$$\Rightarrow\mathrm{0}=\left(\mathrm{2x}^{\mathrm{2}} −\mathrm{1}\right)\left[\mathrm{1}\pm\mathrm{2x}\sqrt{\mathrm{2}}\:+\mathrm{2}\left(\mathrm{2x}^{\mathrm{2}} −\mathrm{1}\right)\right] \\ $$$$\left(\mathrm{i}\right)\:\mathrm{2x}^{\mathrm{2}} =\mathrm{1}\:;\:\mathrm{x}_{\mathrm{1},\mathrm{2}} \:=\pm\:\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\:\rightarrow\begin{cases}{\approx\mathrm{0}.\mathrm{707}}\\{\approx−\mathrm{0}.\mathrm{707}}\end{cases} \\ $$$$\left(\mathrm{ii}\right)\:\mathrm{4x}^{\mathrm{2}} \pm\mathrm{2}\sqrt{\mathrm{2}}\:\mathrm{x}−\mathrm{1}=\:\mathrm{0} \\ $$$$\:\rightarrow\begin{cases}{\mathrm{x}_{\mathrm{3},\mathrm{4}} \:=\:\frac{−\mathrm{2}\sqrt{\mathrm{2}}\:\pm\:\mathrm{2}\sqrt{\mathrm{6}}}{\mathrm{8}}=\frac{−\sqrt{\mathrm{2}}\:\pm\sqrt{\mathrm{6}}}{\mathrm{4}}\begin{cases}{\approx\mathrm{0}.\mathrm{25}}\\{\approx−\mathrm{0}.\mathrm{965}}\end{cases}}\\{\mathrm{x}_{\mathrm{5},\mathrm{6}} \:=\:\frac{\sqrt{\mathrm{2}}\:\pm\sqrt{\mathrm{6}}}{\mathrm{4}}\:\rightarrow\begin{cases}{\approx\mathrm{0}.\mathrm{965}}\\{\approx−\mathrm{0}.\mathrm{25}\:}\end{cases}}\end{cases} \\ $$$$\left(\mathrm{iii}\right)\mathrm{for}\:\sqrt{\mathrm{1}−\mathrm{x}^{\mathrm{2}} }\:\mathrm{defined}\:\mathrm{on}\:−\mathrm{1}\leqslant\mathrm{x}\leqslant\mathrm{1} \\ $$$$\left(\mathrm{iv}\right)\:\mathrm{2x}^{\mathrm{2}} −\mathrm{1}\geqslant\mathrm{0}\:;\:\mathrm{x}\leqslant−\frac{\sqrt{\mathrm{2}}}{\mathrm{2}}\:\cup\:\mathrm{x}\geqslant\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$$\mathrm{solution}\:\mathrm{is}\:\left\{\pm\frac{\sqrt{\mathrm{2}}}{\mathrm{2}};\:\frac{−\sqrt{\mathrm{2}}\:\pm\sqrt{\mathrm{6}}}{\mathrm{4}}\:;\:\frac{\sqrt{\mathrm{2}}\:\pm\sqrt{\mathrm{6}}}{\mathrm{4}}\:\right\} \\ $$
