Question-102450

Facebook Tweet Pin

Question Number 102450 by naka3546 last updated on 09/Jul/20

Commented by naka3546 last updated on 09/Jul/20

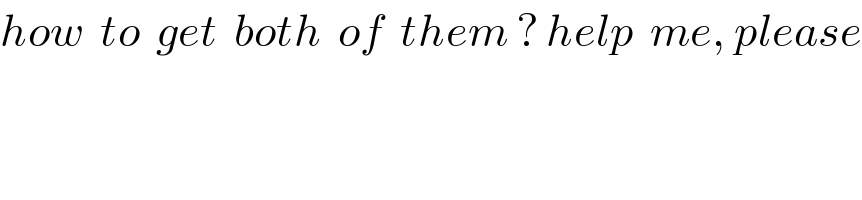
$${how}\:\:{to}\:\:{get}\:\:{both}\:\:{of}\:\:{them}\:?\:{help}\:\:{me},\:{please} \\ $$

Commented by bemath last updated on 09/Jul/20

$${maclaurin}\:{series} \\ $$

Answered by Rio Michael last updated on 09/Jul/20

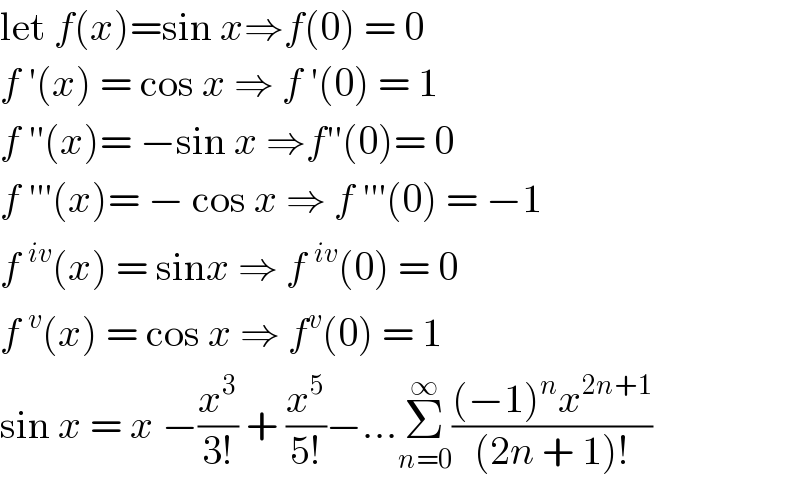
let f(x)=sin x⇒f(0) = 0 f ′(x) = cos x ⇒ f ′(0) = 1 f ′′(x)= −sin x ⇒f′′(0)= 0 f ′′′(x)= − cos x ⇒ f ′′′(0) = −1 f^(iv) (x) = sinx ⇒ f^(iv) (0) = 0 f^v (x) = cos x ⇒ f^v (0) = 1 sin x = x −(x^3 /(3!)) + (x^5 /(5!))−...Σ_(n=0) ^∞ (((−1)^n x^(2n+1) )/((2n + 1)!))

$$\mathrm{let}\:{f}\left({x}\right)=\mathrm{sin}\:{x}\Rightarrow{f}\left(\mathrm{0}\right)\:=\:\mathrm{0} \\ $$$${f}\:'\left({x}\right)\:=\:\mathrm{cos}\:{x}\:\Rightarrow\:{f}\:'\left(\mathrm{0}\right)\:=\:\mathrm{1} \\ $$$${f}\:''\left({x}\right)=\:−\mathrm{sin}\:{x}\:\Rightarrow{f}''\left(\mathrm{0}\right)=\:\mathrm{0} \\ $$$${f}\:'''\left({x}\right)=\:−\:\mathrm{cos}\:{x}\:\Rightarrow\:{f}\:'''\left(\mathrm{0}\right)\:=\:−\mathrm{1} \\ $$$${f}\:^{{iv}} \left({x}\right)\:=\:\mathrm{sin}{x}\:\Rightarrow\:{f}\:^{{iv}} \left(\mathrm{0}\right)\:=\:\mathrm{0} \\ $$$${f}\:^{{v}} \left({x}\right)\:=\:\mathrm{cos}\:{x}\:\Rightarrow\:{f}^{{v}} \left(\mathrm{0}\right)\:=\:\mathrm{1} \\ $$$$\mathrm{sin}\:{x}\:=\:{x}\:−\frac{{x}^{\mathrm{3}} }{\mathrm{3}!}\:+\:\frac{{x}^{\mathrm{5}} }{\mathrm{5}!}−…\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}} {x}^{\mathrm{2}{n}+\mathrm{1}} }{\left(\mathrm{2}{n}\:+\:\mathrm{1}\right)!} \\ $$

Answered by Dwaipayan Shikari last updated on 09/Jul/20

f(x)=f(0)+f ′(0)x+((f ′′(0))/(2!))x^2 +((f ′′′(0))/(3!))x^3 +((f ′′′′(0))/(4!))x^4 +.... f(x)=sinx f ′(x)=cosx f′′(x)=−sinx f ′′′(x)=−cosx f^(iv) (x)=sinx so f(x)=sinx=0+x+0−(x^3 /(3!))+0+(x^5 /(5!)).... sinx=x−(x^3 /(3!))+(x^5 /(5!))−(x^7 /(7!))+........

$${f}\left({x}\right)={f}\left(\mathrm{0}\right)+{f}\:'\left(\mathrm{0}\right){x}+\frac{{f}\:''\left(\mathrm{0}\right)}{\mathrm{2}!}{x}^{\mathrm{2}} +\frac{{f}\:'''\left(\mathrm{0}\right)}{\mathrm{3}!}{x}^{\mathrm{3}} +\frac{{f}\:''''\left(\mathrm{0}\right)}{\mathrm{4}!}{x}^{\mathrm{4}} +…. \\ $$$${f}\left({x}\right)={sinx} \\ $$$${f}\:'\left({x}\right)={cosx} \\ $$$${f}''\left({x}\right)=−{sinx} \\ $$$${f}\:'''\left({x}\right)=−{cosx} \\ $$$${f}^{{iv}} \left({x}\right)={sinx} \\ $$$$\:\:{so} \\ $$$${f}\left({x}\right)={sinx}=\mathrm{0}+{x}+\mathrm{0}−\frac{{x}^{\mathrm{3}} }{\mathrm{3}!}+\mathrm{0}+\frac{{x}^{\mathrm{5}} }{\mathrm{5}!}…. \\ $$$${sinx}={x}−\frac{{x}^{\mathrm{3}} }{\mathrm{3}!}+\frac{{x}^{\mathrm{5}} }{\mathrm{5}!}−\frac{{x}^{\mathrm{7}} }{\mathrm{7}!}+…….. \\ $$

Answered by PRITHWISH SEN 2 last updated on 09/Jul/20

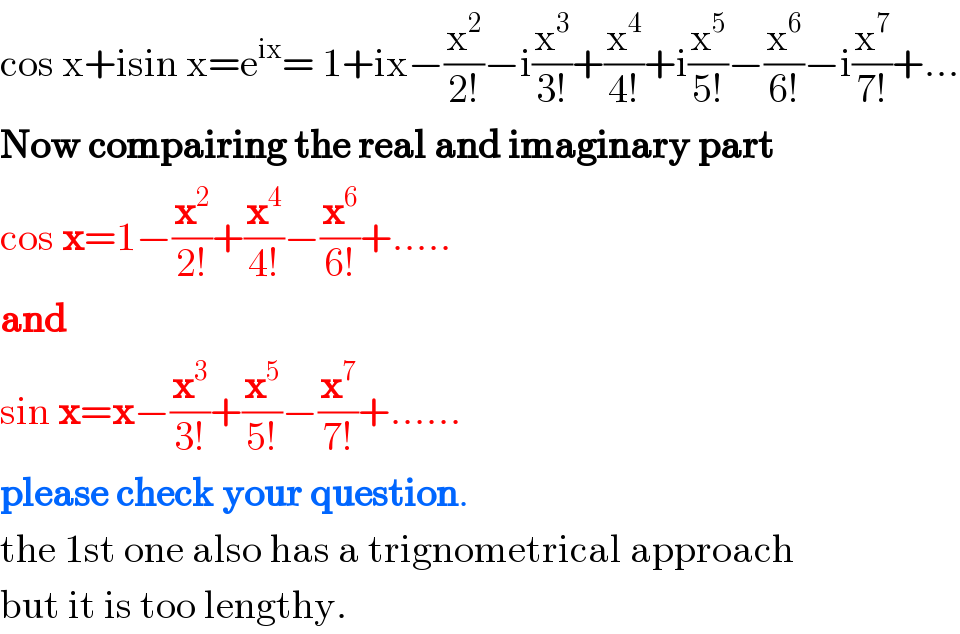
cos x+isin x=e^(ix) = 1+ix−(x^2 /(2!))−i(x^3 /(3!))+(x^4 /(4!))+i(x^5 /(5!))−(x^6 /(6!))−i(x^7 /(7!))+... Now compairing the real and imaginary part cos x=1−(x^2 /(2!))+(x^4 /(4!))−(x^6 /(6!))+..... and sin x=x−(x^3 /(3!))+(x^5 /(5!))−(x^7 /(7!))+...... please check your question. the 1st one also has a trignometrical approach but it is too lengthy.

$$\mathrm{cos}\:\mathrm{x}+\mathrm{isin}\:\mathrm{x}=\mathrm{e}^{\mathrm{ix}} =\:\mathrm{1}+\mathrm{ix}−\frac{\mathrm{x}^{\mathrm{2}} }{\mathrm{2}!}−\mathrm{i}\frac{\mathrm{x}^{\mathrm{3}} }{\mathrm{3}!}+\frac{\mathrm{x}^{\mathrm{4}} }{\mathrm{4}!}+\mathrm{i}\frac{\mathrm{x}^{\mathrm{5}} }{\mathrm{5}!}−\frac{\mathrm{x}^{\mathrm{6}} }{\mathrm{6}!}−\mathrm{i}\frac{\mathrm{x}^{\mathrm{7}} }{\mathrm{7}!}+… \\ $$$$\boldsymbol{\mathrm{Now}}\:\boldsymbol{\mathrm{compairing}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{real}}\:\boldsymbol{\mathrm{and}}\:\boldsymbol{\mathrm{imaginary}}\:\boldsymbol{\mathrm{part}} \\ $$$$\mathrm{cos}\:\boldsymbol{\mathrm{x}}=\mathrm{1}−\frac{\boldsymbol{\mathrm{x}}^{\mathrm{2}} }{\mathrm{2}!}+\frac{\boldsymbol{\mathrm{x}}^{\mathrm{4}} }{\mathrm{4}!}−\frac{\boldsymbol{\mathrm{x}}^{\mathrm{6}} }{\mathrm{6}!}+….. \\ $$$$\boldsymbol{\mathrm{and}} \\ $$$$\mathrm{sin}\:\boldsymbol{\mathrm{x}}=\boldsymbol{\mathrm{x}}−\frac{\boldsymbol{\mathrm{x}}^{\mathrm{3}} }{\mathrm{3}!}+\frac{\boldsymbol{\mathrm{x}}^{\mathrm{5}} }{\mathrm{5}!}−\frac{\boldsymbol{\mathrm{x}}^{\mathrm{7}} }{\mathrm{7}!}+…… \\ $$$$\boldsymbol{\mathrm{please}}\:\boldsymbol{\mathrm{check}}\:\boldsymbol{\mathrm{your}}\:\boldsymbol{\mathrm{question}}. \\ $$$$\mathrm{the}\:\mathrm{1st}\:\mathrm{one}\:\mathrm{also}\:\mathrm{has}\:\mathrm{a}\:\mathrm{trignometrical}\:\mathrm{approach} \\ $$$$\mathrm{but}\:\mathrm{it}\:\mathrm{is}\:\mathrm{too}\:\mathrm{lengthy}. \\ $$

Facebook Tweet Pin

Leave a Reply Cancel reply