Question Number 37084 by Rio Mike last updated on 08/Jun/18
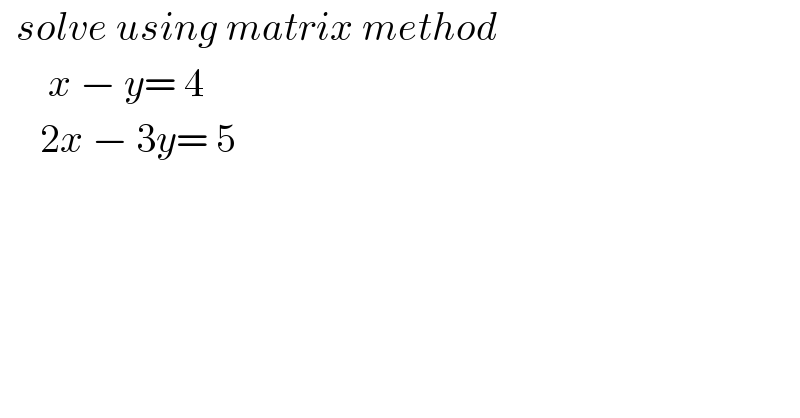
$$\:\:{solve}\:{using}\:{matrix}\:{method} \\ $$$$\:\:\:\:\:\:{x}\:−\:{y}=\:\mathrm{4} \\ $$$$\:\:\:\:\:\mathrm{2}{x}\:−\:\mathrm{3}{y}=\:\mathrm{5} \\ $$
Commented by math khazana by abdo last updated on 09/Jun/18
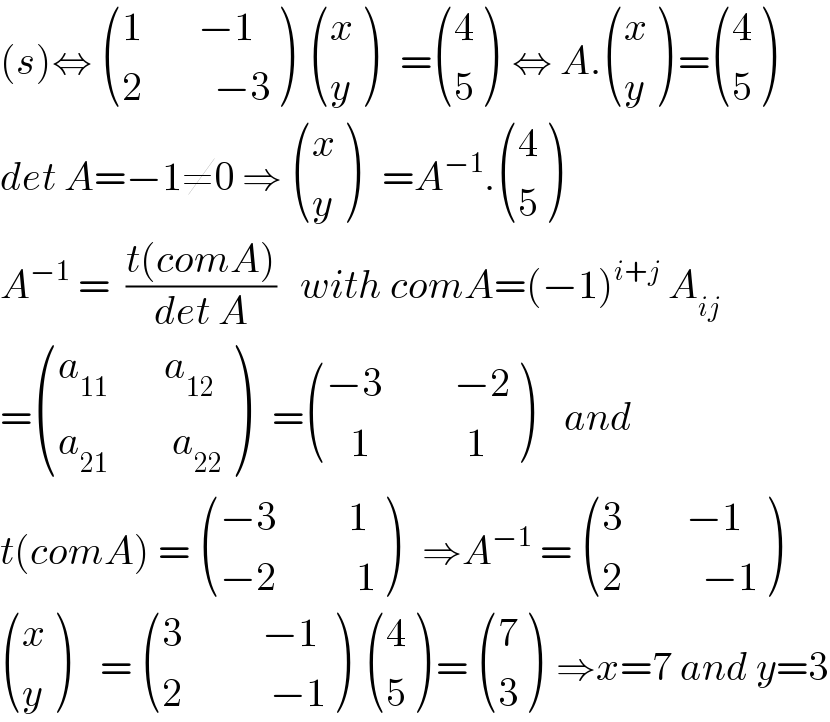
$$\left({s}\right)\Leftrightarrow\:\begin{pmatrix}{\mathrm{1}\:\:\:\:\:\:\:−\mathrm{1}}\\{\mathrm{2}\:\:\:\:\:\:\:\:\:−\mathrm{3}}\end{pmatrix}\:\:\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix}\:\:\:=\begin{pmatrix}{\mathrm{4}}\\{\mathrm{5}}\end{pmatrix}\:\:\Leftrightarrow\:{A}.\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix}\:=\begin{pmatrix}{\mathrm{4}}\\{\mathrm{5}}\end{pmatrix} \\ $$$${det}\:{A}=−\mathrm{1}\neq\mathrm{0}\:\Rightarrow\:\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix}\:\:\:={A}^{−\mathrm{1}} .\begin{pmatrix}{\mathrm{4}}\\{\mathrm{5}}\end{pmatrix} \\ $$$${A}^{−\mathrm{1}} \:=\:\:\frac{{t}\left({comA}\right)}{{det}\:{A}}\:\:\:{with}\:{comA}=\left(−\mathrm{1}\right)^{{i}+{j}} \:{A}_{{ij}} \\ $$$$=\begin{pmatrix}{{a}_{\mathrm{11}} \:\:\:\:\:\:\:{a}_{\mathrm{12}} }\\{{a}_{\mathrm{21}} \:\:\:\:\:\:\:\:{a}_{\mathrm{22}} }\end{pmatrix}\:\:\:=\begin{pmatrix}{−\mathrm{3}\:\:\:\:\:\:\:\:\:−\mathrm{2}}\\{\:\:\:\mathrm{1}\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{1}}\end{pmatrix}\:\:\:\:{and} \\ $$$${t}\left({comA}\right)\:=\:\begin{pmatrix}{−\mathrm{3}\:\:\:\:\:\:\:\:\:\mathrm{1}}\\{−\mathrm{2}\:\:\:\:\:\:\:\:\:\:\mathrm{1}}\end{pmatrix}\:\:\:\Rightarrow{A}^{−\mathrm{1}} \:=\:\begin{pmatrix}{\mathrm{3}\:\:\:\:\:\:\:\:−\mathrm{1}}\\{\mathrm{2}\:\:\:\:\:\:\:\:\:\:−\mathrm{1}}\end{pmatrix} \\ $$$$\begin{pmatrix}{{x}}\\{{y}}\end{pmatrix}\:\:\:\:=\:\begin{pmatrix}{\mathrm{3}\:\:\:\:\:\:\:\:\:\:−\mathrm{1}}\\{\mathrm{2}\:\:\:\:\:\:\:\:\:\:\:−\mathrm{1}}\end{pmatrix}\:\:\begin{pmatrix}{\mathrm{4}}\\{\mathrm{5}}\end{pmatrix}\:=\:\begin{pmatrix}{\mathrm{7}}\\{\mathrm{3}}\end{pmatrix}\:\:\Rightarrow{x}=\mathrm{7}\:{and}\:{y}=\mathrm{3} \\ $$
Commented by math khazana by abdo last updated on 09/Jun/18

$${this}\:{method}\:{is}\:{general}\:{for}\:{n}\:{unknown}\:\left({x}_{{i}} \right)\:{if} \\ $$$${the}\:{matrix}\:{A}\:{is}\:{inversible}\:. \\ $$
